This problem solving activity has a logic and reasoning focus.

In how many ways can she put two strawberry milk cartons into the crate?
- Rotate patterns through quarter and half turns.
- Count possibilities.
This problem develops two concepts. The first is counting all possible arrangements, and the second is noticing that some of these arrangements are ‘alike' and so might be considered to be the same.
The first part of this problem has students following these steps:
- find some answers to a problem;
- think about whether there are any more answers or not;
- try to explain why there are no more answers.
The students should be encouraged to try to find a number of answers and to reach a point where they have some systematic idea as to why there are no more answers. There are three important skills that are fundamental to all of mathematics; being able to find some possibilities, finding all possibilities, and then justifying that there are no more. We work through this sequence in the Solution to this problem.
The second idea in this problem is symmetry. This involves noticing that turning some arrangements of the milk cartons through quarter turns, will give another arrangement. The two arrangements are said to be ‘alike'. The aim is to find such arrangements, put them into groups and find how many such groups there are. This will confirm the number of different arrangements, or the number of groups that are not ‘alike'.
This problem is one of a series of 8 logic and reasonsing problems based around the theme of no-three-in-a-line. The other lessons in this sequence are Strawberry and Chocolate Milk, Level 1; Three-In-A-Line, Level 2; No Three-In-A-Line, Level 3; More No-Three-In-A-Line, Level 4; No-Three-In-A-Line Again, Level 5; No-More-In-A-Line; Level 6 and No-Three-In-A-Line Game, Level 6. The problems increase in difficulty through the sequence and the skills discovered in one problem are used in the next.
- Copymaster of the problem (Māori)
- Copymaster of the problem (English)
- Coloured pens and paper
- Bottle tops
- Square box or region (to act as the crate)
- 2 by 2 Copymaster
- 3 by 3 Copymaster
The Problem
Hannah has a square milk crate that can hold four cartons. In how many ways can she put two strawberry milk cartons into the crate?

Teaching Sequence
- Talk about Hannah's 'crate' or carton holder.
Why might she have a small crate?
What shape is the crate? What do we know about that shape?
How many cartons can she get into her crate? - Discuss how the students might approach the problem, including how they will record their ideas.
- Have students work on the problem and support them with questions.
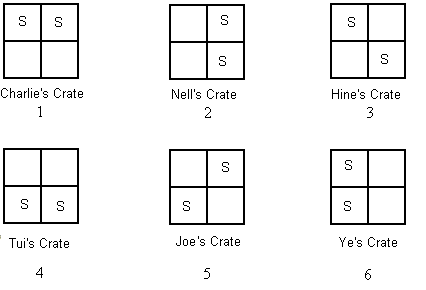
- As different solutions emerge, have students take turns to show to the class an arrangement they have found. Give a student's name to each solution.
- Have them display their solutions so that the class can refer to them. These can be added to if another one is discovered.
- Ask
How do you know if there are any more? - Encourage students to use a systematic approach to ensure they find all possible answers.
- Ask the class
What happens if you turn Charlie’s crate by a quarter turn?
Does this give you someone else’s crate? Whose?
What happens to Hine’s crate if she turns it through a half turn? - Then try asking questions like
If I have just turned the crate through a quarter turn and I’ve got Joe’s crate, whose crate did I start with? - Ask: Do any of these crates look the same? Do some of them have anything in common?
- Call crate arrangements that can be rotated into each other ‘alike'.
- Ask students to find groups of 'alike' crates. Have them see that rotating the crate will move one arrangement to another (see the Solution).
- Have them agree on a problem solution.
- The Extension problem provides an opportunity to apply their learning and to see if there are any answers that may be alike.
- Discuss their conclusions.
Extension
Hannah found a slightly bigger crate. This one had room for 9 cartons. In how many ways can she put 8 strawberry milk cartons into her new crate?

Solution
The students may use a drawing or equipment to solve the problem. They are likely to approach this unsystematically at first. For example:
The question is, are there any more?
To answer that question we need to be systematic. Draw it so that nothing is missed. One way to do this is to first put one milk carton in the top left-hand corner of the crate and then move the other around the crate from one possible arrangement to the next. This will give us the following possibilities.
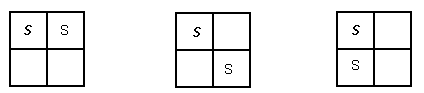
There are only three ways of doing this. We’ve now exhausted all possibilities for a bottle in the top left-hand corner of the crate. So now move on to the top right hand corner.

So there are only two new possibilities here. We list them below.

Now let’s put the first bottle in the bottom left-hand corner. This gives us only one new answer.

Any other arrangement that has a bottle in the bottom left-hand corner, forces the other bottle to be in a position we’ve already considered. By being systematic, we’ve accomplished the last two steps of the problem. We’ve got all possible answers (step (ii)) and we’ve shown that these are all there are (step (iii)).
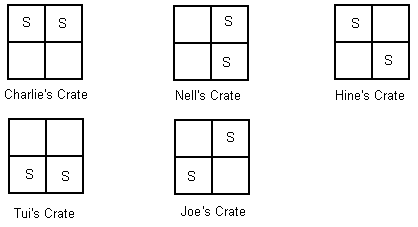
Here are the six possible answers.
Do some of the six answers above look the same? Do some of them look different?
3 and 5 look more like each other than 1, 2, 4, and 6. You see 2 and 5 show bottles opposite each other while in 1, 2, 4, and 6 the two bottles are on the same side of the crate.
Ask students to model rotations of the square crate that would produce arrangements that are 'alike'.
There are two groups of ‘alike' arrangements. These are 3 and 5, and 1, 2, 4, and 6. Therefore there are only two arrangements of the cartons in the crate and every other arrangement comes from one of these just by rotating the crate. These two arrangements are represented by the non-alike crates of Charlie and Hine.
Solution to the Extension
The easiest way to be systematic is to look at where the space without a bottle can be. Take it systematically round the crate starting at, say, the top left-hand corner of the crate. There are 9 possibilities.
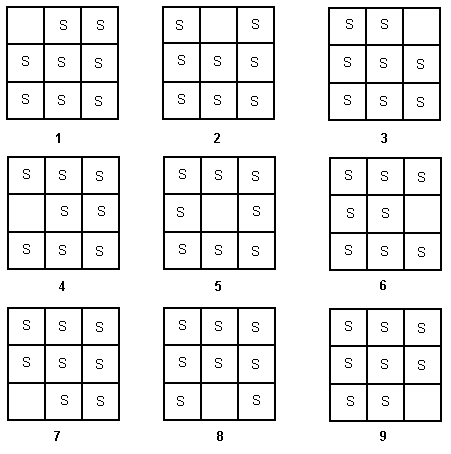
Then consider the notion of ‘alike'.
You can see that 1, 3, 7, and 9 are 'alike'. They all have a corner square free. You can rotate any one of these crates through a quarter turn onto any of the others.
Second, 2, 4, 6, and 8 are alike. They all have the middle square on a side empty. Again you can rotate from any one of these to any other.
Finally 5 is on its own. It has the centre space vacant. When you rotate it, the centre space stays in the centre. It can't be matched up to any other arrangement.
There are three distinct groups of answers under rotation. These are represented by the arrangements 1, 2 and 5.
You may like to consider the properties of the arrangements.
Are there any crates that stay the same after a rotation of a quarter turn?
Are there any crates that stay the same after a rotation of a half turn?
Consider further variations on the crate size and the number of cartons.