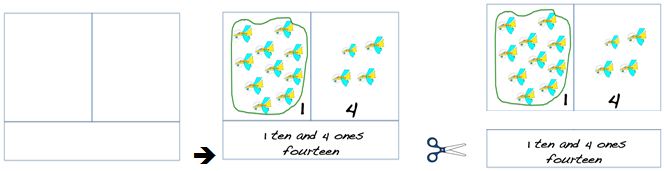
The purpose of this unit of sequenced lessons is to develop knowledge and understanding of the place value structure of numbers from ten to twenty.
- Instantly recognise patterns for teen numbers.
- Make groups of ten and represent teen numbers with materials.
- Recognise and record words and symbols for teen numbers.
- Understand that in a teen number the 1 represents one group of ten.
- Expand teen number notation and understand simple place value.
- Understand and apply a ten for one exchange.
- Understand how to decompose a ten in order to subtract.
When ākonga meet the number ten they meet a two-digit number for the first time. They begin to develop awareness of the concept that there are no more numerals to learn and we just ‘recycle’ them. This is their entry into the structural world of our tens based number system.
Ākonga are introduced to the language of digits, place and value. It is a considerable conceptual shift for ākonga to move from a face value understanding (i.e. that a numeral represents a number of units that can be counted) to a complex place value understanding in which a numeral can represent a group or a number of groups, that are in themselves made up of units that can be counted.
As ākonga study teen numbers and their meaning and structure (rather than simply ‘saying’ them in a counting sequence), the focus is on developing the understanding that the value of a digit depends on its' place. This is not trivial and it is made more challenging by the language of teen numbers.
Ākonga often confuse the number names such as ‘fourteen’ and ‘forty’ because the adult enunciation of the word endings is often unclear. In hearing ‘fourteen’ ākonga may expect to see the 4 appear first in the symbolic form because that is the number that comes first when they say it. Seeing 14 and hearing ‘four – teen’ therefore has the potential for confusion.
Ākonga need many opportunities to make these numbers with materials. When using place value material for the first time, ākonga need the opportunity to group single units to make one ten. By doing this they come to understand that ten ‘ones’ or units do in fact comprise one 'ten'. The first equipment to use therefore is that which can be physically grouped, one by one, to make or compose one group of ten, or a ‘ten’, and be able to be unpacked or decomposed again into ten ones. When this is complemented by symbolic recording that accurately matches the representation of the number, understanding of two-digit notation is developed. Equipment in which the tens are already pre-grouped can be used once grouping to make ten is well understood.
It is a considerable shift for ākonga to then use materials in which the ten looks completely different from the ones (for example, money) and to trust the 'ten for one' trade. The greatest abstraction is the digits in our number system, where the tens and ones look exactly the same but it is only their place that tells their value.
In depth exploration of place value with teen numbers is essential if our ākonga are to work with real understanding of the numbers within our number system.
The activities suggested in this series of lessons can form the basis of independent practice tasks.
The learning opportunities in this unit can be differentiated by providing or removing support to ākonga and by varying the task requirements. Ways to differentiate the tasks include:
- introducing fewer activities and repeating activities for those ākonga who need more support.
- working with the numbers 11-12 before introducing the numbers 13-19
- providing multiple sets of materials where ākonga can 'make ten' and then 'make teen numbers'
- using some of the suggested activities as independent activities for ākonga who need greater challenge. These could be set up as a bus stop activity.
Using the place value language structure within te reo Māori to develop and reinforce the understanding that teen numbers represent 'ten and' may support conceptual understanding of teen numbers. Display a chart to reinforce this conceptualisation. Oral communication of te reo Māori for numbers up to 20 will also be useful in the context of enhancing the interest and cultural relevancy of this learning.
| tahi | 1 | tekau mā tahi | 11 |
| rua | 2 | tekau mā rua | 12 |
| toru | 3 | tekau mā toru | 13 |
| whā | 4 | tekau mā whā | 14 |
| rima | 5 | tekau mā rima | 15 |
| ono | 6 | tekau mā ono | 16 |
| whitu | 7 | tekau mā whitu | 17 |
| waru | 8 | tekau mā waru | 18 |
| iwa | 9 | tekau mā iwa | 19 |
| takau | 10 | rua tekau | 20 |
- Tens frames
- Ice-block sticks
- Elastic hair ties or rubber bands
- Plastic beans (or other counters)
- Plastic containers
- Arrow cards Please note that you only need the arrow cards 0-9 and 10
- Playing cards (ace-9) or Numeral Cards 1-9
- Copymaster 1
- Copymaster 2
- Copymaster 3
- Copymaster 4
- Copymaster 5
Session 1
SLOs:
- Instantly recognise patterns for teen numbers.
- Make groups of ten and represent teen numbers with materials.
- Recognise and record words and symbols for teen numbers.
Activity 1
- Show the ākonga single tens frames. Have them show the same number of fingers as the number of dots, say and write the number with their finger in the air or on the mat.
- Show two tens frames, one of ten and the other of a number less than ten, together making a teen number. Have ākonga ‘write’ with their finger how many dots they see. For example:

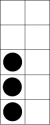 13
13
Repeat with several teen numbers.
- Write the numbers 11 – 19 in symbols and words on chart paper, highlighting the inconsistencies of the language and exploring for fun alternative forms of some of the teen numbers, for example, eleven (oneteen), twelve (twoteen), thirteen (threeteen), fifteen (fiveteen). For each word, as appropriate, underline teen in the word, practice saying it and hearing the final consonant, ‘n’. Make the connection between this ‘teen’ word and ‘ten’. Retain this chart to add to later. This chart could also include words for 'teen' numbers from other languages relevant to your ākonga.
- Ask your ākonga what they notice about all of these numbers. (They all have 'teen' at the end and are ten and ‘something’). They are known as teen numbers.
Activity 2
Have ākonga work in pairs to play Teen Pairs. Consider pairing together ākonga of mixed mathematical abilities to encourage tuakana/teina.
Purpose: to recognise and match teen number representations and the words ‘ ten and ____’
Ākonga place between them two piles of cards face down (Copymaster 1).
Pile 1: Tens frame teen number cards (showing two tens frames).
Pile 2: Word Ten and _____ cards.
Ākonga take turns to turn over one tens frame teen number card and say the number of dots they see. They then turn over a word card and read the words aloud. If the tens frames and word card match, they keep the matching pair. The winner is the ākonga with the most pairs.
For example:
Activity 3
- Have ākonga work in pairs to ‘make’ their own group of ten. Give each pair of ākonga forty ice-block sticks, two elastic hair ties, pens and chart paper or a mini whiteboard.
- Have each ākonga pair choose and write in symbols a number from eleven to nineteen and take that many sticks. Have them count out and make one bundle of ten using the hair tie, then write and draw what they have. For example:



12 is ten and two - Have them unbundle and return their sticks, then repeat with another teen number making use of spare sticks as required.
- Ask the ākonga to return to the whole group with their drawings, keeping them hidden. Have the ākonga take turns to describe to the class what they have drawn, and ask another ākonga to say what number it is. The drawing is then shown.
- The kaiako concludes by recording the ‘ten and _________’ words beside each of the teen numbers on the class chart begun in the earlier activity. Also consider exploring together the Place Value sticks animation, available from https://e-ako.nzmaths.co.nz/modules/PVanimations/
Session 2
SLOs:
- Make groups of ten and represent teen numbers with materials.
- Recognise and record words and symbols for teen numbers.
- Understand that in a teen number, the 1 represents one group of ten
Activity 1
- Have ākonga sit with a partner. Tell them that each pair is going to be making teen numbers on their fingers and ask them to discuss how they will do this. Look and listen for those ākonga who immediately identify that one of their pair will be the ‘ten person’, holding up ten fingers each time.
- Hold up a mixture of cards with number names in words, symbols and those reading ‘ten and ___’. (Copymaster 2). Each time, check to see if ākonga pairs can achieve the cooperative representation on their fingers.
- The kaiako makes a teen number from ice block sticks, having ākonga count to ten as the ten bundle is made.






Hold up the ten bundle and ask, 'Do I still have ten sticks here?' (yes) 'How many bundles of ten do I have?' (1). Record this on the chart, for example, I have fifteen. 15 is ten and five. 15 is 1 ten and 5 ones. Discuss the language of ‘ones’ and that sometimes ‘ones’ can be called ‘units’.
- Model some more examples then have ākonga individually draw and write about some of their favourite teen numbers. For example '12 is 1 ten and two ones'.
Activity 2
If available, have ākonga work in small groups or pairs to explore, find and display the four matching cards in the BSM 9-1-48 card game.
Alternatively, ākonga can make a class puzzle matching game. Provide each ākonga with card, pens and scissors. Have them make their own puzzle pieces which can then be combined with those made by their classmates and mixed up to make a matching pairs game. Before cutting these up, photocopy an extra 3 or 4 sets to be used later on in this unit.
Activity 3
- Return to the class chart started in Session 1. Record te reo Māori words for teen numbers, highlighting ‘tekau mā’ is ‘ten and’, connecting this mathematics language with the other expressions already recorded.
- Photocopy a few more sets of the matching game ākonga made in Session 2, Activity 2. Distribute this game so that your ākonga can play it in small groups of 3-4. The purpose of this game is to match word, pictorial and symbol representations of teen numbers.
Ākonga deal out 7 cards each. The remaining pile of cards is placed in the centre of the group. Ākonga take turns to ask one other player for a card needed to complete a set of 3 teen family cards. If the other player does not have the card sought, the requesting player takes one from the pile. As sets are complete, ākonga place these in front of them.
The winner is the player with the most complete sets.
Session 3
SLOs:
- Understand that in a teen number, the 1 represents one group of ten.
- Expand teen number notation and understand simple place value.
- Understand and apply a ten for one exchange.
Activity 1
- Display the chart started in Session 1. Record beside the numbers 11 – 19 the description ‘1 ten and x ones’ for each of the numbers.
- Using enlarged arrow cards demonstrate and discuss the place value notation that we use, highlighting tens and ones language.


- Introduce ākonga to plastic beans and containers. Have them work in pairs to make up containers with ten beans in each and discuss what the containers will be called. (a container is ‘one ten’ or ‘a ten’). Have ākonga discuss the similarities between the sticks they have been using and the beans.






NB. The container for the beans looks different from the ones, but can still be unpacked. This is a subtle and important shift. Also consider exploring together as a group or class the place value beans animation, available from https://e-ako.nzmaths.co.nz/modules/PVanimations/ - Give ākonga time to become familiar with the beans and the arrow cards. Have them make and model teen numbers with the equipment, explaining this to their partner.
- Have each ākonga complete a think board sheet (Copymaster 3) or a mini poster about one of their favourite teen numbers. Display these.
Activity 2
Ākonga play Go Teen in pairs. A tuakana/teina model could work well here.
Purpose: to use ten ones to make one group of ten, when adding two single-digit numbers.
Ākonga have playing cards (ace - 9), shuffled and face down between them. Alternatively you could print out numeral cards 1-9. They have single beans and empty tens containers (or single ice block sticks and hair ties), single digit and tens arrow cards available.
The players take turns to turn over two playing cards. When the two numbers are added, if they make less than ten they return them face down to a discard pile.
If they make more than ten they keep their playing cards, take the total number of beans, group the materials showing the total as 1 ten and units. They also show the number with the arrow cards.
However, if the number has already been made by their partner, (the arrow cards for that number have been used) the ākonga must simply return their playing cards to the discard pile.
The winner is the player with the most tens (containers with beans) when all the arrow cards have been used up.
Session 4
SLOs:
- Understand and apply a ten for one exchange.
- Understand how to decompose a ten in order to subtract.
Activity 1
- Kaiako models a teen number with containers and beans and asks, ‘What number is shown here?’. For example, 18:









A problem is posed in which the number being subtracted requires the ten to be ‘unpacked’ or decomposed:
'Here are the beans Gardener Gavin is going to plant. He plants 9 in the first row. How many beans are left to plant in the second row? How can we work this out?'
Ākonga can discuss strategies for subtracting 9 and suggest what they can do with the materials. Kaiako models this and some more examples can be explored together.
- Ākonga are provided with place value materials and are each given some subtraction problems to solve with decomposition (Copymaster 4). Ākonga should record with pictures, words and an equation what they did and what their result is. The thinkboard (Copymaster 3) could be used again - it could be laminated and be reusable with a whiteboard pen.
- Share as a class and discuss. The language of making ten (composing) and breaking ten (decomposing) can be introduced.
Activity 2
- The kaiako asks a ākonga to model twenty using place value material. Discuss what this represents: two tens is the same as twenty. Rua tekau is a good example of this concept.
- Have the ākonga play in pairs or small groups First to twenty.
Purpose: to understand how to compose and decompose a ten.
Equipment: Ākonga beans and containers, numeral cards 11- 14, a set of playing cards 2 – 5, a dice with a + or – symbol marked on each of the six faces, mini whiteboards and markers.
How to play:- Numeral cards are spread out face down.
- Each ākonga selects a card and makes that number using place value equipment.
- Players take turns to roll the dice and turn over a playing card. They follow the instructions on the card, either adding or subtracting from their materials.
- Each time an ākonga has a turn they are required to write the equation.
- The winner is the first ākonga who has two containers of ten beans (twenty).
For example: one ākonga turns over and models 13, rolls + and 3, and makes 16.
At their next turn the ākonga may have to – 4, followed on the next turn by – 3.
This will require the ākonga to decompose the ten.
The ākonga will have recorded for the three turns so far:
13 + 3 = 16
16 – 4 = 12
12 – 3 = 9
Conclude the lesson with a focus on the words, ‘place value’. The kaiako writes ‘place value’ on a chart and asks ākonga what this could mean. They are encouraged to look at all the recording of teen numbers completed throughout these lessons. Accept all responses, but conclude by highlighting and recording that 'the place of a numeral in a number tells us what it is worth or its value.' Show the enlarged arrow cards drawing attention to the words tens and ones.

Dear parents and whānau,
In maths this week we have been learning about teen numbers and how they are made of ten plus a single digit number. For example, thirteen is 10 + 3. We have also been introduced to place value, learning that the 1 in 13 is in fact one group of ten.
You can help your child practice this very important idea by playing the game, Teenager match.
Cut up the attached page to make separate cards. Spread them face down on the table. Take turns to choose a pair of cards. If they match, keep the pair. The winner is the person with the most pairs.
[Include a copy of Copymaster 5]