Properties of Operations

Session 1 Biscuits

Here the students try to find general rules relating to a subtraction problem disguised as a problem involving eating biscuits.

1. A family bought a packet of 20 biscuits and they ate 6. There were 14 left. 20 – 6 = 14. Illustrate this by using  for remaining biscuits and  for eaten biscuits
   .
   When you know that 20 – 6 = 14, what other subtractions do you immediately know the answer to?
   It might be useful to draw up a table to put the students’ suggestions that might include:

Suggestions	Illustrations	20 – 6 = 14
if they ate one more, there would be one fewer left
		20 – 7 = 13
if they ate two more, there would be two fewer left
		20 – 8 = 12
if they ate one fewer, there would be one more left
		20 – 5 = 15
if they had 5 more to start with, but ate the same number, there would be 5 more left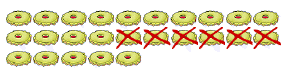
		(20 + 5) – 6 = 14 + 5 = 19
if they had 5 more to start with, and ate 5 more, there would be the same number left
		(20 + 5) – (6 + 5) = 14
if they had bought twice as many and eaten twice as many, there would be twice as many left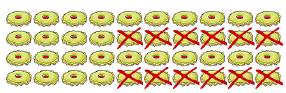
		(2 x 20) – (2 x 6) = (2 x 14)
if they had bought half as many and eaten half as many, there would be half as many left
		Half of 20 – half of 6 = half of 14

2. Discuss students’ suggestions and get them to illustrate why their idea works using the diagram (or models) of the biscuits.
3. Some of the students’ suggestions will be true only for the actual numbers involved. They will not be general properties of subtraction. Someone may, for example, suggest that if the family ate four more biscuits, there would only be ten left. Direct students to general properties, where possible, that will hold for all numbers of biscuits. For instance, all of the suggestions in the table above are general. This is because the verbal statements would apply to any number of biscuits you care to choose for the initial subtraction. General statements can be tested by trying other numbers of biscuits and seeing if the verbal statement still holds true.
4. Summarise the general properties and test them on other numbers.

Session 2 Subtraction

In this session, students explore and test properties of subtraction.

1. Ask a student to come and write a complicated subtraction on the board and work out the answer. Difficulty will vary from class to class, but the student may for example, choose

   

2. Ask students to suggest other subtractions they can now do easily, using this answer. They will probably suggest that the top line can be increased (e.g. by 1, 2, 100, 1000, see below), or decreased (e.g. by 30, see below) giving corresponding increases and decreases in the answers. Such examples can be done mentally and checked with a calculator or written algorithm.

   

3. Ask the students to explain the reason behind this property of subtraction with reference to a simple context such as buying something costing initially $473 from a bank account containing $2358.
4. Get students to explain, in their own words, why increasing (or decreasing) the number subtracted causes the answer to be decreased (or increased) by the same amount.
5. Ask the students to explain the reason behind this property of subtraction, with reference to a simple context.
6. Ask the students to suggest other things that we can easily work out using the answer to this subtraction and to explain their reasoning.

   Examples: if both numbers are increased by the same amount, the answer is not changed, if both numbers are doubled or halved, the answer would be doubled, if both are multiplied by ten, the answer is multiplied by ten.

7. Students can now choose their own complicated subtraction, work it out and make up some other subtractions that they can now easily do. These should be checked on a calculator. Then they write down the ten favourite subtractions that they have found and explain how they knew they would be correct.

Session 3 Multiplication

This session follows the same steps as the above session on subtraction, with a stronger emphasis on checking a variety of numerical examples.

1. Both relationships that work and relationships that do not work should be discussed. For example, if one number is multiplied by ten, then the answer is multiplied by ten. However, if one number is increased by ten, then the answer is not (usually!) increased by ten
2. Test proposed relationships on a variety of easy numbers e.g. to test proposal that “ if I double one number and have the other, the product remains the same” check: 10 x 6 = 60, 20 x 3 = 60, 5 x 12 = 60 and 4 x 5 = 20 and 2 x 10 = 20 and 8 x 2.5 = 20.
3. Calculators can be used to check harder examples too.

Session 4 Division

This session follows exactly the same steps as the above sessions on subtraction and multiplication.

Session 5 Addition and Consolidation

Here the students work by themselves on addition. This session is an attempt to bring together the ideas of the previous sessions.

1. Remind the class what has been done in the last four sessions. Ask them to work with another member of the class but this time concentrate on addition. Remind them that they have to guess a rule and then check it.
2. Get the various groups to report back to the class.
   What did you find?
   Why did it work?
   Will it always work?
3. Recall the different things that worked for the four operations.
   Are there any rules that are the same?
   Are there any rules that are different?
4. Get the class to summarise what they have found on a poster. Display the posters.