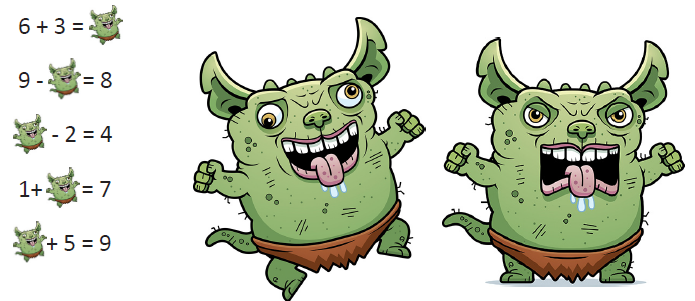
This problem solving activity has a number (addition and subtraction) focus.
Oh no, gremlins have been in and covered up some numbers on the chart.
Can you put the equations back together?
- Model and explain addition facts to 10 and then 20.
- Model and explain subtraction facts to 10 and then 20.
- Devise and use problem solving strategies (act, draw).
This problem reinforces the use of addition and subtraction equations. Students become so accustomed to seeing addition equations in the form 1 + 5 = ___ and 5 – 2 = ___, that they automatically think that the "answer" is always the sum or the difference. If the students are only exposed to the traditional format they miss the opportunity to form understandings about all parts of the equation and how each part relates to the other.
The context of this problem could be adapted to use the footprints or marks of a character from a picture book you have recently read in class.
- Copymaster of the problem (English)
- Copymaster of the problem (Māori)
- Pictures of gremlin footprints or "marks" to introduce the problem
The Problem
Oh no, gremlins have been in and covered up some numbers on the chart. Can you put the equations back together?
Teaching Sequence
- Create the scenario of gremlins visiting the class and drawing faces in place of numbers.
- Read the problem to the class.
- Ask the students to think about ways that they could solve the problem.
- Let the students solve the problems. As they work, circulate and ask:
Which numbers were easiest to figure out? Why?
Which numbers were hardest to find? Why was that?
How can you tell that you have found the right missing number?
Do you think that more than one number might work? Why or why not? - Share solutions.
What strategy did you use to find the missing number?
Did anyone use a different strategy?
Extension
The gremlins have covered more than one number. Find as many number combinations as you can that work
J + J = 13
6 + J = J
Solution
9, 1, 6, 6, 4
Solution to the Extension
At Level 1, students have not developed knowledge of negative numbers. Therefore, there is a limited number of possibilities for ? + ? = 13. Check that the students have remembered the (0, 13) pairing.
There are an infinite number of possibilities for 6 + ? = ? As the students work on this problem they will often use a sequence of numbers and notice the patterns in it, for example:
- 6 + 1 = 7
- 6 + 2 = 8
- 6 + 3 = 9 etc.