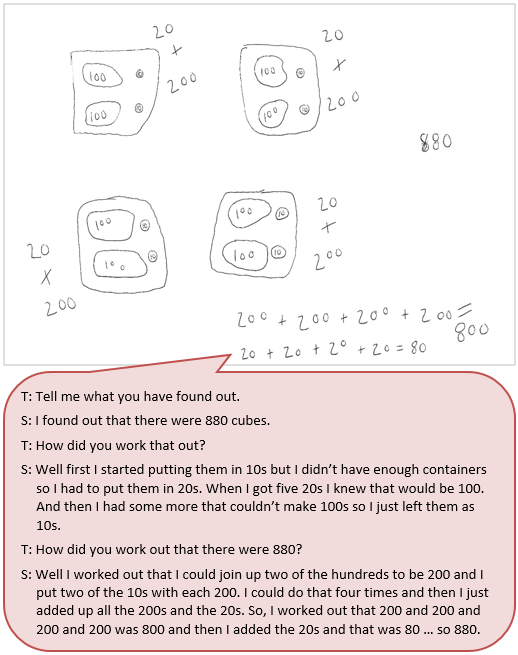
This counting collections activity engages students in counting a collection of objects.
Collections can be counted reliably and accurately by grouping sets of objects. Students can record their count using words, numbers and pictures.
Counting collections promotes number sense, and is an essential foundation for students to be successful mathematicians. Recent literature (e.g. Boaler, 2008) suggests that flexible grouping practices best supports equitable opportunities for student learning.
It is important to share the mathematical focus with students. This task provides students with opportunities to group objects strategically to make the count easier, to record their strategies and to share and justify their counts and strategies using a variety of representations. Estimation of quantities, and checking by counting promotes number sense. The progression in the sophistication of students’ thinking when asked to count a collection of objects goes from counting in ones, to counting in groups, reasoning additively to reasoning multiplicatively.
Consider the mathematical language your students are likely to use when grouping and counting, and the language you want to develop. Provide students with opportunities to work collaboratively, to record and share their thinking and their counts.
Student agency is promoted if students have choice over their own counting and recording methods. Rather than suggesting particular solutions or counting methods to students, teachers can use enabling prompts to support students who require assistance. Extending prompts can be offered when students have completed the task to build more sophisticated strategies and understandings.
- stationery (pencils, pens etc.
- counters
- playing cards
- puzzle pieces
- sports equipment
- equipment to aid grouping e.g. muffin trays, plastic cups, tens frames, set rings, number lines..
- Children can also be encouraged to bring their own collections along for the class to count.
Task Introduction
A wondering for mathematical inquiry can be developed by teachers in consultation with their students. Possible questions could include:
- I wonder how many … there are?
- I wonder whether we have all the pieces in the game?
- I wonder if we have enough … for everyone?
There are a number of picture books which could support this inquiry such as ‘The Button Box’ by Margarette S. Reid
Considerations when planning for the task introduction include:
- Do the students understand the context of the task?
- What key mathematical ideas and language do teachers need to clarify with students in order for them to engage with the task?
Student Exploration of the Task
- While the students are exploring the task, listen to their mathematical language and thinking, particularly around grouping and place value.
- If students are struggling to get started, enabling prompts can be offered, but only when students have had sufficient time to struggle with the problem themselves.
Examples include:
Start with counting this part of your collection.
Can you use something to help you group your count?
How can you prove that there are…
- Extending Prompts can be offered if students finish quickly or find the task easy. They encourage higher order thinking and generalisation within the same task.
Examples include:
Can you reorganise the grouping of that collection to count it more efficiently?
What patterns have you noticed in your count?
Record the patterns so that you can share them with others.