Primes and Emirps

Activities and Investigations

These activities and investigations are based around prime and non-prime numbers, including square numbers.
Activity One begins with an illustration that makes a connection between the number of factors that a number has and the number of rectangles that can be formed with that many square tiles.
Take, for example, the number 6. Two rectangles can be formed from 6 tiles:

The dimensions of these rectangles, 2 x 3 and 1 x 6, correspond to the factors of 6, which are {1, 2, 3, 6}.
For each prime number, only one rectangle can be formed. For example, here are the rectangles for the prime numbers 7 and 13:

So the factors of 7 are {1, 7} and the factors of 13 are {1, 13}. It is also true to say that, in the set of whole numbers, 7 is only divisible by 1 and 7 and 13 is only divisible by 1 and 13.
Prime numbers are sometimes referred to as non-rectangular numbers, and numbers with 3 or more factors as rectangular numbers.
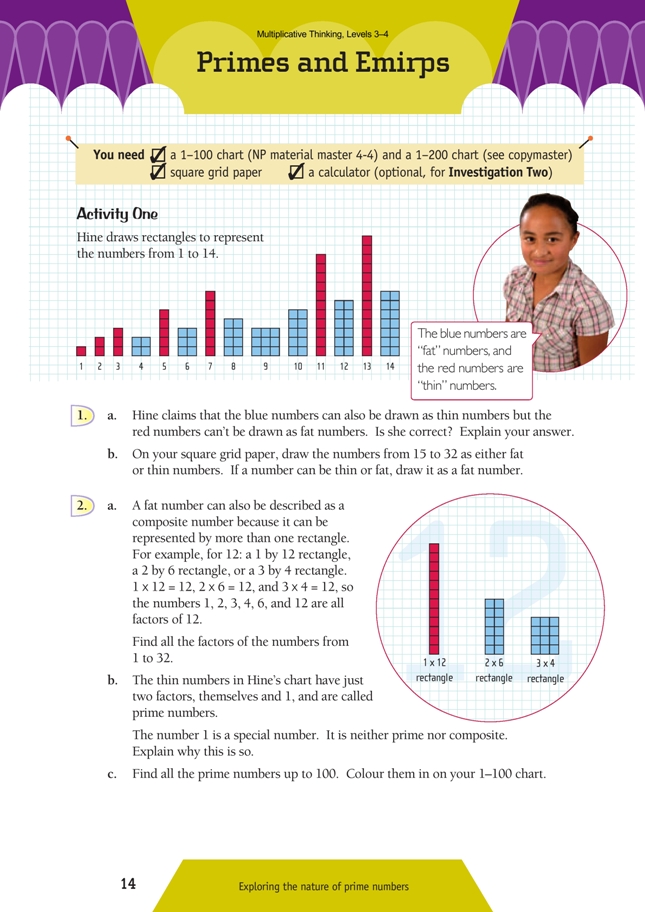
As can be seen with the factors of 6, factors usually exist in pairs. The number 12 has these pairs: 1 x 12, 2 x 6, and 3 x 4. Most rectangular numbers have an even number of factors – but not all do. For example, 16 is a rectangular number, but it has an odd number of factors: {1, 2, 4, 8, 16}.

The reason for this is that 16 is a square number, which is a special kind of rectangular number. A square number has a factor that is used twice (in the case of 16, this factor is 4), but it only appears once on the list of factors for that number.
Rectangular numbers are also referred to as composite numbers because they are composed of prime factors. This means that they can be expressed as the product of primes. For example, 12 = 2 x 2 x 3 and 15 = 3 x 5. The prime factorisation of any composite number is unique; no other combination will work. This is known as “the fundamental theorem of arithmetic”.
This theorem is the basis of the sieve of Eratosthenes, a method for finding prime numbers, which is explored in Activity Two. Eratosthenes, a mathematician of ancient Greece, was famous for calculating the circumference of the Earth. His sieve works by eliminating all the composite numbers, leaving only the prime numbers behind.
The process involves removing the multiples of 2, then 3, then 5, then 7, and so on. Because these numbers are multiples, they must be composite. The sieving process only needs to be continued until the square root of the largest number in the sieve is reached. So for the numbers 1 to 100, the multiples of 7 are the last to be eliminated because the next prime (11) is greater than the square root of 100 (10). This works because factors exist in pairs and for every prime factor less than the square root, there is a complementary factor greater than the square root. The factors close in on
the square root from below and above.
Investigation One asks students to work out a way to find primes up to 200. The Answers explain how to do this. The closest square root to 200 is √196 = 14, so the multiples of 13 are the last that need to be found. You could extend this activity by asking your students to find the primes up to 900. To do this, all the multiples of 2, 3, 5, 7, 11, 13, … 29 must be eliminated because these numbers are the primes less than 30, which is the square root of 900.
Prime numbers up to 10 000 can be found in this way using a spreadsheet program. The square root of 10 000 is 100, so each number needs to be divisibility-tested only for prime numbers up to and including 97.
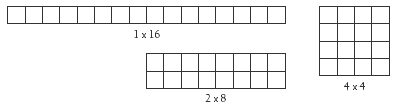
The spreadsheet shown here can be used to test any number up to 10 000 and see if it is prime. The number to be tested is entered in cell D2.

To set up this spreadsheet, enter all the prime numbers up to 97 in column A. In cell B2, enter the formula =$D$2/A2, then fill down in column B to cell B26 (opposite 97 in column A). The formula checks the number entered in D2 for divisibility by each of the prime numbers in column A. If a whole number appears in column B, then
the number in cell D2 is divisible by the complementary prime in column A, so it cannot be prime itself. In the example illustrated, 1 791 can’t be prime because 3 goes into it 597 times.

Investigation Two

The students can use the primes they found in Investigation One to find some lower value emirps in question 2. Have them discuss how they approached finding other 2- and 3-digit emirps. An Internet search on emirps, as suggested in the Answers, would be worthwhile, and the students could extend that to searching for information on primes. If nothing else, they will be exposed to the passion for maths that many mathematicians feel.

Answers to Activity

Activity One
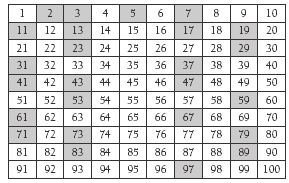
1. a. Yes, Hine is correct. The red numbers are prime numbers. Prime numbers only have two factors, themselves and 1, so they are always “thin” numbers. “Fat” numbers are not primes and have more than 2 factors. Two of their factors are themselves and 1. For example, 6 can be shown as a thin number because two of its factors are 6 and 1. But 2 and 3 are also factors of 6, so it is a fat number on the grid.
b. Fat numbers may vary, depending on the factors you choose.

2. a. The only factor of 1 is 1.
The factors of 2 are 2, 1.
The factors of 3 are 3, 1.
The factors of 4 are 4, 2, 1.
The factors of 5 are 5, 1.
The factors of 6 are 6, 3, 2, 1.
The factors of 7 are 7, 1.
The factors of 8 are 8, 4, 2, 1.
The factors of 9 are 9, 3, 1.
The factors of 10 are 10, 5, 2, 1.
The factors of 11 are 11, 1.
The factors of 12 are 12, 6, 4, 3, 2, 1.
The factors of 13 are 13, 1.
The factors of 14 are 14, 7, 2, 1.
The factors of 15 are 15, 5, 3, 1.
The factors of 16 are 16, 8, 4, 2, 1.
The factors of 17 are 17, 1.
The factors of 18 are 18, 9, 6, 3, 2, 1.
The factors of 19 are 19, 1.
The factors of 20 are 20, 10, 5, 4, 2, 1.
The factors of 21 are 21, 7, 3, 1.
The factors of 22 are 22, 11, 2, 1.
The factors of 23 are 23, 1.
The factors of 24 are 24, 12, 8, 6, 4, 3, 2, 1.
The factors of 25 are 25, 5, 1.
The factors of 26 are 26, 13, 2, 1.
The factors of 27 are 27, 9, 3, 1.
The factors of 28 are 28, 14, 7, 4, 2, 1.
The factors of 29 are 29, 1.
The factors of 30 are 30, 15, 10, 6, 5, 3, 2, 1.
The factors of 31 are 31, 1.
The factors of 32 are 32, 16, 8, 4, 2, 1.
b. 1 has only one factor, itself, so it is not regarded as a prime number.
c. The numbers shaded on the board below are primes between 1 and 100. (The only even prime number is 2. Apart from the number 5, all other prime numbers end in 1, 3, 7, or 9.)

Activity Two
The sieve should give you all the primes found on the hundreds board.
Investigation One
You have already found all the primes to 100 and discovered that apart from 2 and 5, all the primes end in 1, 3, 7, or 9. The next primes after 7 are 11 and 13.
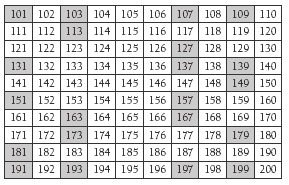
The most efficient way to find the primes between 101 and 200 is to sieve out any multiples of 2, 3, 5, 7, 11, or 13. New instructions might be:
6. Circle 11 because it is prime, and then cross out all the multiples of 11 except 11 itself.
7. Circle 13 because it is prime, and then cross out
all the multiples of 13 except 13 itself.
8. Now circle all the numbers that have not been circled or crossed out.
Following all the instructions for numbers between 101 and 200 will give you the extra primes in this range. Finding the multiples of 11 and 13 also covers off the
only multiple of 17 in this range that is a prime (17 x 11 = 187). Multiples up to 200 of primes larger than 17 are already covered off by finding the multiples of 2, 3, 5, and 7.
The shaded numbers below are the primes between 101 and 200 inclusive:

Investigation Two
1. a. No. Many palindromic numbers are even numbers or end in 5 and are therefore
multiples of 2 or 5. Some palindromic primes are: 131, 353, 373, 383, 727, 757, 787, and 919.
b. Some 5-digit palindromic primes are: 10301, 11411, 12421, 14741, 95959.
2. a. The 8 emirps on the hundreds board are: 13, 31, 17, 71, 37, 73, 79, 97. There are 28 three-digit emirps, including 107, 113, 149, and 157. (Try an Internet search
under “emirps”.)
b. Some 4-digit emirps are: 1009, 1021, 1031, 1033, 1061, 1069.