This is a level 2 activity from the Figure It Out series.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (558 KB)
The terms “chance” and “probability” are used almost interchangeably. Chance reflects the randomness of an event, for example, the result when a dice is thrown. Probability is the likelihood of an event occurring, for example, the likelihood of throwing a 5 on a dice is 1/6. Experimental situations help students develop their understanding of chance and probability. Data-handling skills, such as gathering and organising data, also come into play. Meaningful learning takes place when a student’s intuitive understanding of probability is challenged by the results of an experiment.
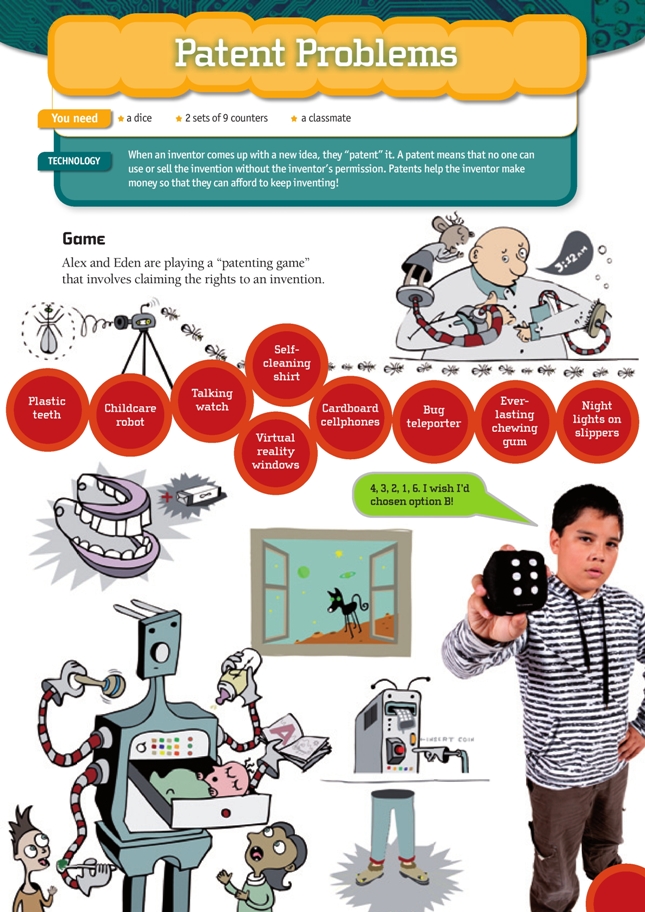
a dice
2 sets of 9 counters
FIO, Creative Technology, Levels 2+-3+, Patent Problems, pages 8 - 9
a classmate
Game
The game involves choosing an outcome and seeing whether the outcome can be obtained within 5 throws of a dice. Some outcomes are easier to obtain than others.
Activity One
Probability can be a difficult concept for students to grasp. In this activity, students examine the relative likelihood of a number of different outcomes.
Discuss the language of probability with the students:
- When we throw a dice 5 times, there is no way to state with confidence what the outcome will be. However, some outcomes are more likely than others. For example, we would be surprised if we threw five 4s in a row.
- The likelihood of an event occurring (for example, getting a 3 within 5 throws) is the same as its probability.
- The theoretical probability of an event can sometimes be worked out by calculating all the possible outcomes and seeing how many times a particular event is represented. This approach is not practical in this situation.
- Experimental estimates of probability involve conducting a number of trials and using the results to estimate the probability of an event. The more trials we do, the closer the experimental estimate of the probability of an event will be to its actual or theoretical probability. This is sometimes referred to as the law of large numbers.
The concept of variation is an important one in statistics. Individual students may get quite different results, but if students compare their results, they will get a clearer idea about the likelihood of each outcome being met. It is good for students to see that increasing the number of trials in an experiment increases the validity of any conclusion.
It is important to link these three different concepts:
- Individual students will obtain their own unique set of results.
- After a large number of trials, a pattern will emerge. This pattern should reflect the theoretical probabilities (where these can be calculated).
- Theoretical probability can tell us which outcomes are more likely, but it cannot predict the next outcome.
Probability is most useful when predicting long-term or long-run results. Even if we know the theoretical probability of every outcome, this will not give us any certainty about short-term outcomes in, say, a game. Throwing four 5s in a row is unlikely, but it’s not impossible. Chance is a factor in most games. Dealing with uncertainty and variation helps students develop the key competency thinking.
Activity Two
Students may find it difficult to devise new outcomes. If so, give them some suggestions and ask them to consider whether the outcomes are very likely or very unlikely. Examples include:
- getting 5 numbers that are all the same
- throwing a dice 5 times and not getting an even number
- throwing a dice 5 times and getting at least one 1 or one 2
- throwing a dice 5 times without getting 2 numbers the same.
Support for English Language Learners
Supporting students with understanding and expressing degrees of probability
English has many ways of expressing probability, including:
- adjectives such as “likely” and “unlikely”
- adverbs such as “maybe”, “probably”, “definitely”
- modal verbs such as “may”, “might”, “must”
- sentences with “if” (which express a condition and a consequence but often have elements of probability too).
The sentence structures and other grammatical rules that express probability are often complex and may be challenging for some students. As well as probability, sentences will often have other elements, such as time relationships and consequences or reasons.
You can support students with this language by providing:
- many examples of the language (and records, such as charts displayed on the classroom walls)
- opportunities to explore the sentence structures and grammatical rules
- explanations of the sentence structures and grammatical rules
- opportunities to co-construct sentences
- opportunities to create their own sentences
- feedback on their use of the language.
Language areas like probability are large and complex. You will need to select small chunks to focus on at one time and keep adding more each time students encounter the language.
Note that using a cline ranging from 0% certain to 100% certain can be a useful strategy for teaching and revising the meanings of different forms for expressing degrees of probability. For examples of clines, see ESOL Online at http://esolonline.tki.org.nz/ESOL-Online/Planning-for-my-students-needs/Resources-for-planning/ESOL-teaching-strategies/Vocabulary/Clines
As always, students also benefit from being able to make connections to their prior knowledge, including cultural and linguistic knowledge.
Technology-related student activities
- Learn about the importance of obtaining a patent, for example, the ownership and marketing of the ballpoint pen. See http://inventors.about.com/library/weekly/aa101697.htm
- Investigate the inventors of technological products such as aeroplanes, television, the electric fence, and bicycles. Emphasise that inventors seldom work in isolation and that most inventions actually result from several people’s ideas and work.
- Research the history of a relatively modern technology such as the telephone. Invite older people to share their stories of dial phones, party lines, and expensive toll charges.
- Predict how a technology in common use may evolve (for example, what will a telephone look like and do 20 years from now?) and make a concept drawing.
Exploring the technology-related context
Technological innovations can earn large amounts of money, and patent laws have been developed to protect inventors’ rights of ownership. These laws can be difficult to police, especially on a global scale. There are fascinating stories about patent disputes and specialist patent lawyers are busy people. Today, the pirating of products and intellectual property is a worldwide concern. Also, sometimes people dispute who actually invented a particular item; television’s origins are apparently claimed by Scotland, Russia, and the US.
Answers
Game
A game to develop your understanding of chance and probability
Activity One
1.–2. Conjectures, experiments, and results will vary. Note that while an experiment can suggest the likelihood of a particular outcome, it can’t provide a definite answer. The more trials you do in a chance experiment, the more accurate any conclusion will be.
Activity Two
1. a. Outcomes will vary. Suggestions include:
i. getting a 3 within 10 throws (based on option B)
ii. getting 4 numbers the same in 5 throws (based on option A)
b. Using the suggestions above, possible justifications include:
i. If you throw a dice 10 times instead of 5, you double the chance of getting a 3.
ii. Throwing the same number 4 times out of 5 is clearly more difficult than 2 times out of 5.
2. i. True. The chance of not getting a 2 or a 4 is the same as the chance of not getting a 5 or a 6 (or any other pair of numbers).
ii. False. Getting a 6 or a 3 are equally likely.
iii. The chance of getting 5 of the same number is extremely small, so “almost impossible” is a reasonable description.