This unit is about partitioning whole numbers. It focuses on partitioning numbers to “make a ten” or a decade when adding whole numbers, for example 8 + 6 can be solved as (8 + 2) + 4. The unit uses measurement as a context.
- Partition numbers less than 10.
- Know and use "teen" facts.
- Solve addition problems by making a ten, or making a decade.
- Solve addition problems involving measurements.
Students at Level Two should understand that numbers are counts that can be split in ways that make the operations of addition, subtraction, multiplication and division easier. From Level One students will understand that counting a set tells how many objects are in the set. At Level Two they are learning that the count of a set can be partitioned and that the count of each subset tells how many objects are in that subset. Students also need to understand that partitions of a count can be recombined. For example, a count of ten can be partitioned into 1 and 9, 2 and 8, 3 and 7, etc.
It is important that students understand that because there are many ways to partition a number and they will need to choice the best partition to suit the question. In this unit we focus on making partitions that allow numbers to make a ten. For example 8 + 6, the 6 can be partitioned to 2 + 4, the 2 then combines with 8 to make a ten 8 + 2, + 4. For this reason teachers should ensure that they select questions that are best solved using the "make a ten" strategy as opposed to partitioning to make doubles. For example, 8 + 7 also encourages the strategy 7 + 7 + 1 rather than 8 + 2 + 5.
This unit uses the context of measurement. Examples and problems should use like measures, and students should be encouraged to write the units when they give an answer, for example 8m + 5m = 13m.
- Plastic animals
- Unifix cubes
- Tens Frames
- Small bottle/s
- Ice cube tray/s
- Numeral flip strip (Material Master 4-2)
- Packet of 10 items, and single items (e.g pens)
Session 1
In this session students investigate the fact that whole numbers can be partitioned in a number of ways.
- Show the students a bag of 8 plastic farm animals and a piece of paper with 2 rectangles drawn to represent 2 paddocks. Tell the students you are going to find all the ways of splitting the animals between the 2 paddocks.
- Ask the students:
How many animals should I put in the first paddock? (Put that number (for example, 3) in that paddock and the rest in the second paddock)
How many animals are in the second paddock? Make sure the students understand there is still 8 animals. - Record for the students 3 + 5 = 8.
- Continue working with the students to find all the pairs of number that add to 8. For example, 6 + 2 and 2 + 6.
- Show the students a length of 7 unifix cubes. Work with students to find different ways of partitioning the 7 cubes. Record the partitions, for example 4 + 3.
- Students can continue to explore partitioning numbers for numbers up to 10. Students can work in pairs or individuals to partition the number and record the results. Students can use unifix cubes, sets of counters, strips of squared paper as materials to help. Students who know the basic addition and subtraction facts to 10 will be able to partition numbers without materials.
Session 2
In this session students investigate that partitioning teen numbers using the number ten. It is easiest to start by making teens numbers as 10 + x.
|
Object |
Length |
Splits |
|
Sellotape dispenser |
|
10 cm + cm |
|
Duster |
|
|
|
Stapler |
|
|
|
Notebook |
|
|
|
etc |
|
|
- Show the students a packet of pens (or item that comes in packs of 10) and 5 single pens.
- Ask the students: how many pens do I have? (Students may count on 11, 12, 13 etc,)
- Record the answer as 10 + 5 = 15. Do several more examples.
- Show the students two tens frames, one complete with 10 dots and another with 6 dots. Ask the students how many dots are there? Again record the answer as 10 + 6 = 16. Using the tens frames work with the students to do solve more examples.
- Students also need to be able to understand that teens numbers can be partitioned. Tell the students that in today’s session the numbers are going to be split into 10 and something.
- Show the students two blank tens frame and a pile of 16 counters. Tell the students you know there are more than 10 counters in the pile, but how many more? Put the counters on the tens frames.
- Ask the students: 16 can be split into 10 plus what?
- Record the answer as 16 = 10 + 6.
- Name some objects in the classroom that are between 11 and 20 cm. Ask the students to measure the length using their ruler and record the answer on the table.
- Other measurement contexts can be used to provide practice activities. For example, capacity. Pour enough water into a bottle to make about 18 ice cubes. Ask the students to pour the water into the ice cubes tray and count how many ice cubes it would make. Ask the students to write their answer as 10 + ? Bottles with different amounts of water can be used.
Session 3
In this session students solve addition problems by partitioning numbers. They use the strategy “make a ten”.
- Show the students a number strip (see Resources) from 0 – 20 and colour in the 10 square. Place 7 counters on the strip. Tell the students that you are going to use the number strip to help add 7 + 5. Show the students a group of 5 counters.
- Ask the students: how many counters will it take to get to 10? (3)
- Put on the 3 counters then ask the students:
How many counters are there to add on? (2)
What does 10 and 2 make?
What two numbers did we split the 5 into? (3 +2)
Why did we chose 3, then 2? (To make it up to 10) - Write the problem 8 + 6 for the students to see.
Ask the students:
How many counters would be need to get to 10? (2)
How many counters are there still to add on? (4)
What is 10 plus 4? (14)
Check the answer using counters and the number strip. - Draw a number line and pose the question 7 + 4.
Start at the 7, ask the students:
How many jumps do we need to add on? (4)
How many jumps is it to get to 10? (3)
How many left over from the 4? (1)
What is 10 and 1? (11) - Students can practise using the make a ten strategy on number lines. Pose questions using the context of measurement and encourage students to write the correct units beside the answer. Possible questions are:
- The bucket had 9 litres in it and Kitiona poured in another 6 litres. How many litres are now in the bucket?
- Anna put 7 cups of juice on the tray and Kiri added another 5 cups to the tray. How many cups were there altogether?
- The temperature was 8oC and it rose 3 degrees during the morning. What is the temperature now?
- Rangi left George’s house and ran 8 minutes then walked for 5 minutes before he got home. How many minutes did it take him to get home?
- Mum bought 7 kilograms of kumara and 4 kilograms of carrots. How much did the vegetables weigh?
Session 4
In this session students solve addition problems by partitioning numbers. They will solve problems that involve adding a 1 digit number to a 2 digit number. The “make a ten” strategy is applied to decade numbers, for example 28 + 7 is solved by making it up to 30 and then adding the remaining 5.
- Pose the problem: If the plant was 37cm tall and it grew 8cm, how tall is it now? Show the students a number line that ranges from 0 – 100, with the 10s numbers coloured in.
- Ask the students: Using the make a ten strategy we used yesterday, how could we solve this problem?
- Work with students to jump 3 to get to 40, then jump the remaining 5 to get to 45.
- Pose the question: The suitcase weighed 17 kilograms and the backpack weighed 5 kilograms. How much did the luggage weigh altogether?
Show the students how they can draw a number line to suit the question.
Ask the students: what numbers are we adding together? (17 and 5)
Draw a line and the number 17 underneath.
Ask the students: what number can be jump to from here? (20)
how many jumps is that? (3)
how many of the 5 are left? (2)
What is the answer? (22) - Students can practise using the make a decade strategy on their own number lines. Pose questions using the context of measurement and encourage students to write the correct units beside the answer. Possible questions are:
- Jane was going home on the bus. The bus took 26 minutes then she walked for 8 minutes. How long did it take for Jane to get home?
- Peni’s plant was 48cm tall. It grew another 7cm. How tall is Peni’s plant now?
- Dad went to the garden shop and bought 16kg of compost and 7kg of fertilizer. How much did it all weigh?
- The painter had 65 litres of paint and he bought another 8 litres. How much paint does he now have?
- In the first three weeks of October Wellington had 88mm of rainfall, in the rest of the month another 7mm fell. How much rain is that altogether?
Session 5
In this session you may wish to continue to give the students opportunities to practise addition partitioning with the make a ten or make a decade strategy. Problems could focus around one measurement theme, for example length. Or problems could focus around a theme such as camping and involve more than one measurement context, for example, weight of packs, time spent on activities, capacity of shower water, length of washing lines, etc.
Alternatively, you may wish to use the formats of session 3 and 4 to show students how this partitioning strategy can be used to solve subtraction problems. For example, 44 – 7, it takes 4 jumps to get back to 40, then the remaining 3 jumps takes us back to 37.
Dear Parents and Whānau,
This week in maths your child has been learning to use a number line and to make jumps to 10 to work out addition questions. Ask them to show you how they jump to the 10 first to solve 8 + 5.
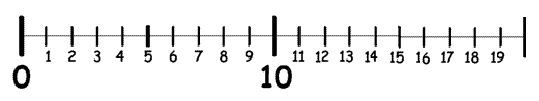
You could pose some other similar problems, like 7 + 4, 9 + 6 and have them use jumps to 10 to solve them. Thank you for your help.