Purpose
This unit supports students in using counting to compare and combine collections.
Achievement Objectives
NA1-1: Use a range of counting, grouping, and equal-sharing strategies with whole numbers and fractions.
NA1-2: Know the forward and backward counting sequences of whole numbers to 100.
NA1-5: Generalise that the next counting number gives the result of adding one object to a set and that counting the number of objects in a set tells how many.
Specific Learning Outcomes
- Use counting to find the number when two different collections are compared (difference).
- Use counting to find the number when two or more collections are joined (addition).
- Use counting to find the number when objects are removed from a collection (subtraction).
Description of Mathematics
Gelman and Gallistel (1978) provided five principles that young students need to generalise when learning to count. These principles are:
- The one-to-one principle
Just like in reading, when one spoken word is matched to one written word, counting involves one-to-one correspondence. One item in a collection is matched to one spoken or written word in the whole number counting sequence. - The stable order principle
The spoken and written names that are said and read have a fixed order. If that order is altered, e.g. “One, two, four, five,…”, the count will not work. - The cardinal principle
Assuming the one-to-one and stable order principles are applied then the last number in a count tells how many items are in the whole collection.
The first three principles are about how to count. The final two principles are about what can be counted.
- The abstraction principle
Items to count can be tangible, like physical objects or pictures, or they can be abstract, like sounds or ideas, e.g. Five types of animal. - The order irrelevance principle
The order in which the items are counted does not alter the cardinality of the collection. This is particularly challenging for students who think that counting is about assigning number names to the items, e.g. “This counter is number three.”
There are other principles of counting that may well be derivatives of Gelman and Gallistel’s original list that also seem important.
- The one more/one less principle
Adding one more item to a collection produces a count that is the ‘next number after’ the original count in the whole number counting sequence. Likewise, but more difficult, removing one item from a collection produces a count that is the ‘number before’ the original count in the whole number counting sequence. - The attribute principle
The number of a collection remains constant, and can be trusted, when items are replaced one for one with those of different size, colour, sound, texture, (or any other distracting feature). - The conservation principle
The number of a collection remains constant, and can be trusted, as the items are moved in space, particularly spread out or compressed, or moved from one part of the collection to another. Piaget created the original conservation experiments. - The comparison principle
The difference in number between two collections that are already counted is the difference between the counts. The difference between counts can be found by ‘counting up’ or ‘down to’ and anticipates the result of matching the items of the sets one to one.
Opportunities for Adaptation and Differentiation
The learning opportunities in this unit can be differentiated by providing or removing support to students, and by varying the task requirements. Ways to differentiate include:
- providing extended opportunities to use equipment to explore five based groupings
- ensuring students are confident operating with smaller numbers, before moving onto larger ones
- supporting students to use counting strategies to check their answers, if needed
- modelling appropriate counting, addition, and subtraction strategies in each session
- strategically organising students into pairs and small groups in order to encourage peer learning, scaffolding, and extension
- working alongside individual students (or groups of students) who require further support with specific area of knowledge or activities.
The contexts for activities can be adapted to suit the interests and experiences of your students. For example:
- Use te reo Māori kupu or terms from your students' home languages when counting numbers.
- Replace the dogs and bones and cars and garages with something that is more relevant to your students, their learning across the curriculum, their interests, and their culture backgrounds (e.g. warriors and tiaha or flagpoles and flags). You could also replace the animals on farms with birds in trees.
Required Resource Materials
- Transparent counters, plastic animals, hundreds board
- Copymasters:
Activity
Session 1
Comparison of two collections is a common task in real life. Along with joining and partitioning collections, comparison forms the suite of problem types to which addition and subtraction are applied. Comparison offers students a chance to trust their counting and apply their understanding of the one more and one less principle.
- Pose the following problem to the students using the pictures from Copymaster 4 to make a storyboard. The pictures can be snipped and copied to an interactive whiteboard or made into cardboard icons and fastened onto a storyboard using Velcro dots. Alternatively use equipment available in your class.
Here are 5 puppies and 6 bones. Is there a bone for each puppy?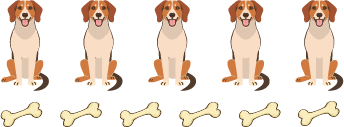
- Discuss how the problem might be solved. Students might suggest matching the puppies and bones in one-to-one correspondence to find out if any bones are left over or if any dogs end up with no bone. Counting both collections and using the whole number sequence to anticipate the difference is a more sophisticated strategy because it applies easily to collections of any size. Using one to one matching to anticipate the result of 99 bones with 101 dogs would be a lengthy process.
- You might illustrate difference by using two number strips with different coloured counters. Build five and six like this:
Visually students should see that one more added to five results in six. Therefore, in the puppy situation there will be one extra bone.
- Vary the puppies and bones problems by making the numbers larger and the differences up to three. This will encourage anticipation using trusting the count and make one-to-one matching more cumbersome. Keep the differences no more than three and use spatial grouping to encourage grouping strategies to count the number of puppies and bones.
For example, How many dogs are there? How many bones are there? Is there a bone for each dog?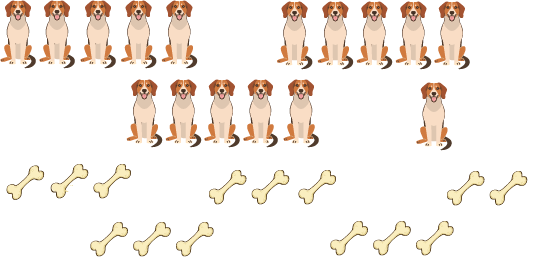
On the number strip the problem solution looks more obvious:
Note the difference of two can be found by counting up from 14 to 16, or by counting down from 16 to 14.
- Progress to imaging by turning the number strips over and building each collection. Without the numerals present students will need to image the counting on or back process.
- Increase the number size of both collections so students generalise the process of finding differences by addition or subtraction.
Session 2
- Use the pictures from Copymaster 4 to ask similar questions to the dog and bone ones from the previous session. For example: “How many more cars are needed to fill all the garages?”
- Provide the students with copies of the pictures from Copymaster 4. Using these pictures students can make up their own comparison problems for another student to solve.
- After students have completed their problems put them into groups of four to share. Bring the class together to discuss strategies for solving the problems. The problems can be made into a book of difference problems for independent work or class discussions.
- Alternatively, use or make up two dice with appropriate numbers. For example, one wooden cube might be labelled 4, 5, 6, 7, 8, 9 and the other 8, 9, 10, 11, 12, 13. The two cubes are rolled, and sets made of the numbers that come up, e.g. 12 cars and 9 garages. Students work out the difference between the numbers, e.g. “Three more garages are needed.”
Session 3
The most significant understanding that develops from counting is that collections can be partitioned and recombined without changing their total number. Part-whole understanding, as it is referred to commonly, is an extension of Piaget’s principle of conservation of number. Two components of part-whole understanding are significant:
- Trusting the count as collections are partitioned and recombined
- Appreciation of the strategic use of partitioning to make calculations easier and for transfer to similar calculations
This activity is aimed initially at the first component though it can be extended to include the second component.
- Draw a farm with paddocks joined by fences on a sheet of paper or the whiteboard. Copymaster 5 has a template if you want to create laminated sheets for student use. Use plastic farm animals or counters to represent animals. Ask a student to put ten animals on their farm or use a single farm as an instructional focus.
- Tell your students to watch as you move one or two animals across the “bridges” to a different paddock. Ask, “How many animals are on the farm now?” Some students are likely to recount while some may accept that the total number is invariant. Repeat, moving animals until the students accept that the total number of animals has not changed. Note that your students may indicate acceptance of the previous number based solely on repetition rather than conservation. So the repetition needs to be disrupted so students apply trust in the count.
- Move two or three animals to different paddocks, then put another animal on the farm in an obvious way. Ask, “How many animals are on the farm now?” Students who trust their previous counting are likely to realise that, in effect, one more animal has been added so the next number, eleven, gives the new number of animals. Similarly move some animals around then remove two animals.
- Your students can work in pairs with a Farmyard card (Copymaster 5), and some counters or plastic farm animals. They begin by putting ten animals on the farm. Then they take turns to rearrange the existing animals, then add or remove up to three animals, all while their partner is watching. The partner must then work out how many animals are now on the farm. Encourage your students to avoid counting the animals unless it is to confirm the number.
Session 4
- Return to the farm game from the previous session. Discuss as a class what you have learned from this game.
- Moving the animals around does not change the total number on the farm.
- You can count on or back to find the total number of animals if some are added or removed, rather than count them all.
- Extend the activity by introducing a “barn” in the form of a plastic cup. Begin with a given number of animals on the farm, say ten. Tell the students to close their eyes. Move some animals around and then place a plastic cup (barn) over the animals in one paddock. Tell the students the same animals are on the farm but some are in the barn. Ask them how many animals they think are in the barn. Discuss their strategies for working the missing number out. Encourage risk taking with a focus on grouping strategies, close approximations lead to known and trusted facts.
- Students can play the barn game in pairs. They can record their answers as equations, e.g. 4 + 2 + 4 = 10. The number of animals they work with can be varied to create more challenge.
- The farm scenario can be used to develop powerful strategies for working out 9 + and 8 + facts. Be aware that students will need to know the ‘teen’ code as prior knowledge, e.g. 10 + 4 = 14 (Fourteen means four and ten). Start with nine animals on one side of the farm and five on the other. Ask, “How many animals are on this (left) side? (nine) How do you know?” “How many animals are on this side? (five) How do you know?“ “How many animals are on the farm altogether?”
- Encourage students to use grouping strategies to count the number of animals. However one by one counting is helpful to illustrate the power of ‘up over ten’ strategies.
Once the answer is accepted (14) move one animal from right to left to form ten and four. “How many animals are on the farm now?” provides same example of developing 9 + facts.
Recording equations helps the students to see patterns that can lead to know facts, e.g. “For 9 + something just take one off the something and make it a teen number.” Similarly for 8 + facts two animals need to be moved.
- Be sure to extend the up through ten to more complex examples, e.g. “There are 29/79/99 animals on this side and five animals on this side. How many animals are there altogether?“
Home Link
Dear families and whānau,
This year we are learning about counting. We need to know the skills of counting like saying the numbers in the correct order forwards and backwards, like “Twenty, nineteen, eighteen,…”, and matching words and objects one to one.
We also need to understand that counting tells us how many objects are in a collection. If we trust that counting tells us how many then we can build on that trust to work out answers we don’t know. For example, we will learn to count on and count back to solve problems. We will also learn how to use facts we know to answer problems we don’t know, by moving objects from one collection to another. For example, we might change 9 + 6 into 10 + 5 because we know 10 + 5 = 15.
Attachments
CountingOnCounting-cm3.pdf182.9 KB
CountingOnCounting-cm4.pdf782.53 KB
CountingOnCounting-cm5.pdf203.69 KB
Add to plan
Level One