In this unit we do a thorough exploration of tan, leading to the students being able to use tan to solve right angletriangles and to solve equations.
- Use tan to solve problems involving right-angled triangles.
- Solve equations of the form tan(θ) = a, for a between –180º and 360º degrees.
- State the value of tan(θ) in special cases.
- Graph y = tan(θ)
This unit takes an investigative approach to tan by encouraging students to start with a definition (an idea) and develop the standard results themselves based on this.
- Strips of paper or light cardboard to make rulers calibrated to non-standard unit lengths
Session 1
In this session students are introduced to the notion of tan of an angle. ‘Tan’ is short for the fuller word ‘tangent’. Tangent is, for reasons which will become clear, what is called a circular function.
-
Get each student to draw a set of axes and a unit circle (say 5cm in radius). Thus 5cm is equivalent to one unit in length. Next draw a line parallel to the y axis that goes through (1, 0) and so touches the circle edge. This is the tangent line.
-
Now get the students to make a ruler calibrated in units and tenth of units using a strip of paper (or light cardboard) as the ruler. This ruler will be used to make measurements of tan of an angle for a unit circle of radius 5cm without the students needing to continually convert from a standard ruler. This special ruler will have 1 unit equivalent to 5cm and 0.1 of a unit equivalent to 0.5cm.
-
Show them the definition (the idea) of tan of an angle. See the diagram below.
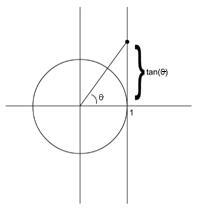
-
Explain that tan of an angle is the distance up the vertical tangent line starting at the x-axis. Get the students to carefully draw an angle of 50º and to measure (with their newly made ruler) tan of 50º. They should get a value of about 1.2 units. Ensure that every student understands this definition of tan(50º). Now do the same for tan(60º) and tan (40º). Their answers should be approximately 1.7 units and 0.8 units, respectively.
-
Now find tan(85º). Students will find, unless they are using a large piece of paper, that they will need a smaller unit circle in this case. Get them to draw a circle of radius 1cm and draw the tangent line. They can now measure tan(85º) using a conventional ruler. Their answers should be about 11 units.
-
Once you are confident that the students understand the definition of tan for angles larger than 0º and smaller than 90º, move on to discuss the definition of angles less than 0º and larger than -90º. Explain that the definition is the same as above, but clearly, in this case, because the angled line cuts the vertical tangent line below the x-axis, the tan will have a negative value. See diagram below.
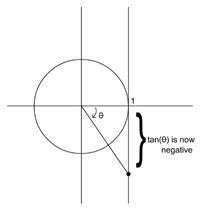
-
Ask the students to use the two unit circles (and the appropriate rulers) to find tan(-35º) and tan (-80º). The answers should be about -0.7 and -5.7, respectively.
-
Now move on to explain how the definition is to be understood for angles in the third quadrant (angles between 180º and 270º). It is important to stress that the definition is the same. There is only one central idea. The rest follows from this idea. The same tangent line is used (the one through the point (1,0)), it is just that the angle line has to be extended in the opposite direction in order to locate where it crosses the tangent line. See diagram below.
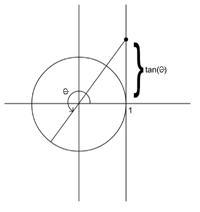
-
It will be clear that the tans of these angles are positive values.
-
Get the students to draw and measure tan(220º). Their answers should be approximately 0.8.
-
Now move on to explain how to deal with angles in the second quadrant. Again stress that the definition is the same as above; that is, there is only one definition for tan, there is only one tangent line. Everything follows from the single definition. See diagram below.
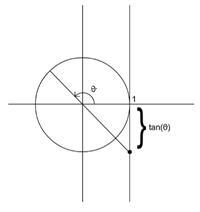
-
Get the students to draw and measure tan(145º). Their answers should be approximately -0.7.
-
It is crucially important that every student fully understands the definition (the idea) of tan and can find tans of the sorts of angles mentioned above.
-
At this point you can check to see if they have understood what you have shown them by asking them to find the angle which has a tan of 2 units. Those who understand should mark the point (2,0) on the tangent line and draw a line between it and the origin and measure the angle. The answer is approximately 63º. Now ask them if this is the only answer. Hopefully the students will realise, if they haven’t already done so, that there is another angle that also has a tan of 2. It is in the third quadrant and is approximately 243º. The fact that there are at least two answers to these sorts of questions is a very important point and worth stressing. See diagram below.
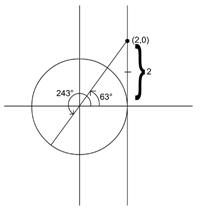
-
Finish this session by getting the students to make a poster that shows what the meaning of tan is.
Sessions 2 and 3
In these sessions students are asked to fully investigate the nature of tan.
-
Begin by revising the definition of tan introduced in Session 1.
-
Investigation Instructions: Using pencil and paper find out as many interesting facts about tan as you can. You should draw unit circles of the sizes you need and if you use unit circles with radii other than 1cm, make special rulers calibrated in the appropriate way to be used with them.
-
If a student has fully understood the definition of tan they will be able to participate in this investigation. The investigation is mainly allowing the students to pinpoint and articulate the sorts of results that they will know by intuition based on the work done in Session 1. In this investigation they will systematically explore the nature of tan and write down what they find.
-
This is a broad domain of investigation and many students will be able to work out for themselves many important results. Some may need some guidance but do not give this too readily because this will detract from the fact that this activity is an investigation of an idea. You need to trust that the work you did in Session 1 is sufficient for the students to do this investigation. However, if guidance is needed, here are some suggestions:
- Why is it easy to give the value of tan(0º)? Are there other angles that are similarly easy to give the tans of?
- Why is it easy to name the value tan(135º)? Which other angles have tans that are similarly easy to name? List them all.
- Describe in your own words the behaviour of the value of tan as the angle moves from 0º round to 360º.
- What happens when the angle is just before or just after 90º? You might need a small unit circle and a large piece of paper here.
- What about 270º? What do you think tan(90º) and tan(270º) should be?
- If you know that tan of an angle is 1.5, use a scale drawing to find one value of the angle. There is another angle that has the same tan. What is it? Can you predict whether the tan of an angle will be positive or negative? How? Can you write down the result as a theorem?
- Explain why it is that, if you know the tan of 10º, you can easily name tan(–10º), tan(170º) and tan(190º). Can you write this down as a theorem?
- If tan of an angle is negative, what can you say about the angle?
- Graph the value of tan, between 0º and 360º, by carefully drawing most of the angles between 0º and 90º in steps of 10 and measuring the lengths of tan. Note: you may need to change the size of the unit circles you use for some angles.
-
Students should make a poster that explains what they have discovered about tan.
-
Towards the end of Session 3 you will need to explain how we deal with the peculiar angles of 90º and 270º (or -90º). Explain that we simply say that there is no such thing as tan of these angles. We say, for instance, that tan(90º) does not exist. We do not say that tan(90º) is infinity because infinity is not a value. We can say that, as the angle gets closer and closer to 90º (from below), tan gets larger and larger. As the angle gets closer and closer to 90º (from above), tan gets larger and larger negative. Eventually tan of an angle just below 90º will become larger than 100. Eventually it will get larger than 1000. Eventually it will get larger than 1 million. Eventually it will get larger than a million billion. And so on. As the angle moves from just a tiny bit before 90º to just a tiny bit after 90º the tan does a massive jump from an enormously large positive value to an enormously large negative value; from positive 1 million billion to negative 1 million billion for instance, that is, a jump of 2 million billion. Name the largest positive number you can think of. You can find an angle (more than one in fact) that has a tan equal to this value. It is impossible to find a value that is so large that there is no angle in the tan relationship to it.
Session 4
In this session the investigation carried out in the previous session is consolidated and extended.
-
Begin this session with a light-hearted test (with a serious purpose). Present, in random order, each of the angle values that have tan values that are easy to name: 0º, 180º, 45º, 135º, 225º, -45º, -135º, and so on. Include 90º, -90º and 270º here, but only if it has become clear that these special values have no tans. Get the students to write down, based on their understanding of the definition, the tan of each of these angles. Then give them questions like this: If you know what tan(30º) is, which other angles can you easily name and what are the tans of these angles.
-
Any students who cannot answer these questions do not understand what tan means. It is important to clarify the situation for these students before any further work is done. The importance of being able to easily name the tans of these angles cannot be over stressed. Not much in the way of memorisation is involved. Ready familiarity with this material follows simply from the definition of tan. If students can gain this familiarity with tan, much of the work they do elsewhere in their current year of study, and in subsequent years of study, will become much easier.
-
Small activity: On the diagram below find the size of the angle BAC, the size of the angle ACB. Find the length AB.
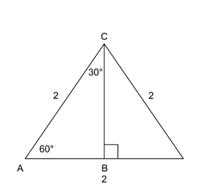
-
Use Pythagoras to find a surd expression for the length BC (answer = √3). Explain why tan(60º) = √3. By reducing the size of the triangle ABC by a factor 1/√3 show why tan(30º) = 1/√3.
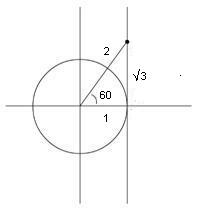
Most students will wonder why this is important. Why not just use a decimal approximation, for instance, and not one of these strange surd expressions? Point out that the square root of a prime number is irrational. That is, to put it in plain language, the value can never be written down as a decimal, no matter how may significant figures you use. And it cannot be written as a recurring decimal either. So the surd expression is exact. It also turns out that many properties of tan that the students will meet later are simpler to find if the surd (and not the decimal approximation) is used.
-
Spend some time memorising these two surd expressions (that is, the expressions themselves, not their decimal approximations): √3 and its reciprocal. Spend some more time becoming familiar with the method of producing these values using an equilateral triangle and Pythagoras. Make a poster showing this method.
-
Next, extend the ‘easy’ angles discussed earlier by including the surd expressions for 30º, 60º, -30º, -60º, 120º, 150º, 210º and so on. Thus, for instance, the students should be able to say fairly easily: tan(120º) is -√3.
-
At this point introduce the use of the calculator. Get the students to check that the ‘tan’ button gives the answers that one would expect from the above. Now show them that ‘inv’ ‘tan’ does the reverse of ‘tan’. Thus they can find an angle with a tan of 4, or 10, or –0.4, and so on.
-
Important: Note again that there are at least 2 angles that have a tan of 4, but the calculator only gives one of these. But because the students have been introduced to tan as they have been, it is easy for them to find the values that the calculator cannot give them.
Session 5
Finally we use tan to find unknown sides and angles in a right angles triangle.
-
Begin this session with another light-hearted test. Present, in random order, each of the values that have tans that are easy to name: 0º, 30º, 60º, 120º, 180º, 45º, 135º, 225º, -45º, -135º, -120º, 90º, -90º, 270º, and so on. Get the students to write down, based on their understanding of the definition (and their memorisation of √3 and its reciprocal), the tan of each of these angles. Then give them questions like this: If you know that an angle has a tan of √3, name two values that the angle could have? These light-hearted tests are very important because they consolidate the ideas presented above. Mostly the answers depend on little more than a sound understanding of what tan means. The only exceptions are the surd values, but these involve memorising two simple facts. It would be wise to continue these little light-hearted tests once the unit is completed. For instance, continue them daily for the first week following the unit and then weekly after that.
-
Now, all the above has been based on the unit circle. But what happens if we do not have a unit circle? What if the circle were of size 2, for instance? All that happens is that the previous situation has been enlarged by scale factor 2.
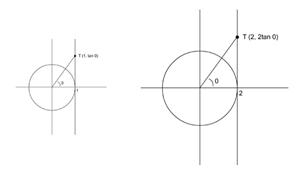
Thus the point T on the unit circle is now (2, 2tan(θ)).
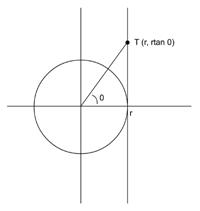
In general, if the circle has size r, the coordinates of T are (r, rtan(θ)).
-
The unit is now finished off by applying the above to the solving of some particular right-angled triangles. This now becomes a straightforward application of the above ideas. Go through the following examples.
-
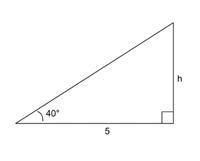
Example 1. Find the unknown, h, in the diagram below.
-
The important step is this: the student must decide which size of circle to use from step 2. In this case the circle must be of size 5. Thus the problem can be shown as below.
-
It is clear that the coordinates of T are (5, 5tan(40º)). Clearly the unknown, h, is just the y-value of T. So h = 5tan(40º). That is, using a calculator, h = 4.20 2DP.
-
Example 2. Find the unknown angle, θ, in the diagram below.
.jpg)
-
Again the important step is deciding the size of circle to use. In this case a circle of size 4 is the easiest (although the problem can be answered using a circle of size 6).
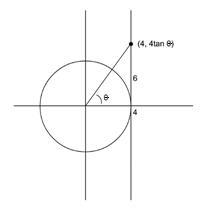
It is clear that 4tan(θ) = 6. So tan θ = 6/4 = 1.5. Find θ using the tan -1 button on the calculator. Thus θ = 56.3 degrees to 1DP.
-
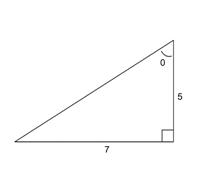
Example 3. Find the unknown angle in the diagram below.
-
The important step, again, is to decide which size of circle to use. In this case the circle must be of size 5. If students choose the inappropriate size by mistake they will quickly realise they cannot easily solve the problem, so they can then change to another size of circle. Thus the problem can be shown as in diagram below.
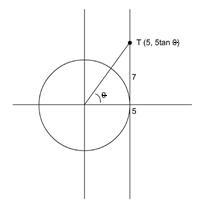
-
It is clear that the coordinates of T are (5, 5tan(θ)). Clearly 7 is the same as the y-value of T. So 7 = 5tan(θ). So tan θ = 7/5. Using the tan -1 button we can find θ. Thus θ = 54.5º to 1DP.
-
Give the students a range of similar problems to practise on.