In this unit we use spinners to develop our understanding of the probability of simple events occurring.
- Determine an experimental estimate of the probability of simple events using frequency tables.
- Determine the theoretical probability of simple events using percentages, fractions and decimals.
- Systematically find all possible outcomes of an event using tree diagrams and organised lists.
Two key ideas are explored in this unit:
- Probability: Each colour or segment of a spinner is a fraction of the total area of the spinner, and the likelihood of the spinner landing on each colour relates to the size of the coloured segment (a fraction).
- Uncertainty: The likelihood of a spinner landing on a particular colour doesn’t determine the actual outcome. Sometimes the spinner lands on the least likely colour.
The learning opportunities in this unit can be differentiated by varying the scaffolding provided or altering the difficulty of the tasks to make the learning opportunities accessible to a range of learners. Ways to support students include:
- providing templates for students to use to record results
- using a digital spinner tool
- minimising the focus on predicting outcomes, focusing instead on exploring results
- spending longer on some sessions, extending them over two days
- working alongside pairs of students who need additional support
- providing opportunities for students to work in flexible groups, thereby allowing students to be scaffolded and extended by their peers.
The activities in this unit draw on the interests and experiences of your students. In particular, sessions 2 and 5 give students the opportunity to use their own ideas to design and make spinners. Spinners can be designed with a cultural link, children can suggest ideas they have about their culture being included. There is a Māori cultural link to spinners which students could make connections to. There are instructions for making Māori spinners on the internet.
Te reo Māori vocabulary terms such as ūponotanga (probability, chance), pāpono (probability event) and matapae (predict, prediction) could be introduced in this unit and used throughout other mathematical learning. Te reo Māori words for numbers and colours can also be used during the creation of spinners.
- Sharp pencils
- Paper clips
- Prepared spinners
- Blank spinners divided into quarters
- Blank spinners divided into thirds
- 2 spinners (for each pair)
- Blank spinners
Session 1
We start the unit by trying to guess which colour the spinner will land on.

- We begin by playing a game.
- Give everyone a piece of red and green paper (any size will do).
- Show the students a spinner, which is coloured ½ red, and ½ green.
- Ask the students to guess which colour the spinner will land on. Tell them to hold that coloured piece of paper up in the air.
- Spin the spinner using a paper clip and pencil.
- Repeat a couple of times giving the students the opportunity to change their guess each time.
- Discuss the game in pairs or small groups:
What can you tell me about this game?
Is it fair? Why or Why not? Draw attention to the equal sizes of the two halves.
Did everyone have an equal chance of winning?
Who thinks they were lucky? What makes you think you were lucky?
What fraction of the spinner is red?
What fraction of the spinner is green?
How else can we write ½? (50%, 0.5)
- Pose the question: If I spun the spinner 30 times how many times do you think it would land on red?
- Spin and record in the results in a tally chart. Compare with students' predictions.
Were we correct? Why or why not?
- Play another game this time using a spinner that is ¾ red and ¼ green. Play the game a couple of times and then discuss.
Which colour did you choose? Why?
Did the spinner always land on that colour? Why not?
Is this a fair spinner? Why not? Draw attention to the differences in sizes of the parts.
Would this be a good spinner to use in a game? Why or why not?
What else can you tell me about the spinner?
What fractions are the colours on the spinner? Encourage students to write these as fractions, decimals, and percentages
Do you think that the size of the segments (colours) is important? Why?
What do you think would happen if I spun the spinner 20 times?
- Ask the students in pairs to design a spinner using three colours that they think would be fair. Tell them to try it out to see if it seems fair.
- Share spinners.
Session 2: Free-time spinners
In today’s session we write activities on our spinners and make predictions about whether we will get our preferred activity. You may be able to use the session to determine what students will do during a regular class activity such as Friday Funday, fitness, or a class game.
- Brainstorm a list of the class's favourite free-time activities, games, sports etc..
- Write 3 of these activities onto a spinner divided into thirds (blank template).
- Take turns getting volunteers to spin the spinner.
Which one do you want to get?
Do you think you will? Why?
What chance have you got of getting the one you want?
What chance have you got of getting one you don’t want?
- Discuss ideas for changing the spinner to make the favoured choice more likely. Hopefully one of the students will suggest changing the size of the segment.
How could we change the spinner to make it better for your choice?
- Give the students a blank circle and ask them to make their own spinner for free choice activities. Tell them that they are to include at least one activity that they don’t want to complete.
- Get the students to try out their spinners keeping track of the results on a tally chart. Ask them to spin it a total of 30 times. Before they spin the spinner ask them to predict how many times the spinner will land on their preferred activity. Encourage students to write these predictions as fractions, decimals, and percentages.
Before you start spinning I want you to write down your prediction for the number of times the spinner will land on your favourite activity.
- Share tally charts and spinners.
Did your spinner work the way that you thought it would? Why or why not?
Was your prediction close?
Which spinner would you choose to use? Why?
Session 3: Odds and evens
Today we will play a game. You will need to make two spinners, one divided in half with the halves labelled 1 and 2, and the other divided into thirds and labelled 1, 2, and 3.
- Give each pair the two spinners and tell them the rules of the game.
- Decide who will be player A and player B.
- Spin both spinners and add the two numbers together.
- If the sum is even, player A gets one point. If the sum is odd, player B gets one point.
- The first player to score 20 points wins.
- Before playing the game ask the students to predict what they think will happen and write this down.
- Remind the students that they need to keep track of the score. Support students to record the score using a frequency table.
We will use a frequency table to list the number of times we land on odd and even numbers.
We will record these as tally marks.
Once one player has scored 20 points, we will count up our tally marks and record them in frequency columns.
Alternatively the students could keep track of the score with counters or cubes.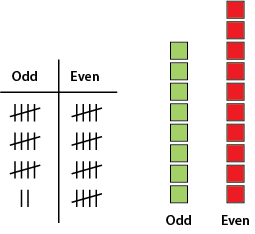
- After playing the game, ask the students to write about the results of the game.
Write about what happened in your game.
How did your results compare to your prediction?
Write down whether you think the game is fair or not.
If you don’t think the game is fair, write about how to make it more fair.
- At the end of the session bring the class together to compile, total and compare results.
How did your results compare with those of the class?
If you played the game again would you change your prediction? How?
Is it possible to predict exactly what will happen? Why?
Support students to consider using a tree diagram, a grid, or an organised list to identify all possible outcomes. Provide examples of these.
Session 5: Spinner games
Today we work with a partner to make spinner games.
- Show the class a spinner and ask for their ideas about a two-player game using the spinner.
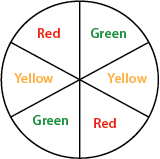
- As a class, write rules for a two-player game. For example:
Decide who will be player A and player B
If the spinner lands on yellow, player A gets 2 points. If the spinner lands on green or red, player B gets 1 point.
The first player to score 20 points wins.
- Ask students to make predictions about the game. They should be encouraged to make predictions not only about individual spins, but about which player they think will win the game.
- Explain to the students that they are to work with a partner to design their own two-player game. They need to design both the spinner and write the rules for their game. This could be linked to their interests or culture.
- After they have made the game ask them to make predictions about it and then play to see if their predictions are correct. The results of their game should be recorded in a frequency table.
- Swap games. Play the game and then write about the game.
Write about what happened in the game.
How did your results compare to your prediction?
Write down whether you think the game is fair or not.
If you don’t think the game is fair, write about how to make it more fair.
- Share findings with other groups or as a class.
Dear parents and whānau,
This week in maths we have been learning about probability. We have been playing games with simple spinners. Your child may like to show you how to make a spinner. Talk with them about how it works, and the kinds of games we have played.