Friends Forever

The calculations in these problems are straightforward, but the context makes them more difficult. Each pattern of beads in this activity forms an equal-groups structure, but the repetition of the pattern in a continuous form makes it harder for students to pick this out. The students will need to use their knowledge of multiples to help them work out the problems. They may begin by modelling or drawing the pattern. The clues with the questions prompt them to use their number knowledge.
You can generate additional examples by asking the students to form a repeating pattern by threading beads or using counters or attribute blocks. Then you can ask other questions based on the students’ patterns. With attribute blocks, you can make the questions more complex by “overlaying” patterns.
This would be a good activity to do before the students make their own designs in question 5. For example, the attribute-block pattern might go thick-thin-thick-thin, square-circle-hexagon-triangle-square- circle-hexagon-triangle, and red-blue-yellow-red-blue-yellow at the same time. Students could predict whether a thin square would ever appear and so on.
For question 1, explain the context, allowing students to thread some repeating patterns if necessary. Make sure they can see the pattern in the beads. Use the prompts to guide discussion about ways to find out the colour of the later beads. Encourage answers that use number properties, especially tables and multiples.
Question 2 asks the students to see the pattern “chunk” as a unit to be repeated to a certain length. At the same time, they have to apply this “chunking” to the two parts of the pattern, which is a useful encouragement of part–whole thinking.

The pattern in question 3 is more complex because it varies in both shape and colour. The question asks the students to explain how their times tables might help them, thus making explicit the number properties solution strategy we want students to move towards. These questions, especially the “How do you know?” part, will stimulate explanations of strategies. Recording these as a group and developing a shared understanding would be a powerful way to help those students who are solving the problem by drawing the pattern.
In question 4, students need to use the information in the diagram (3 shapes = 2 centimetres) and their 10 times tables. This question extends question 2 by including shape and colour elements.
For question 5, look carefully at the patterns the students design to see who can manage to make a pattern with shape and colour elements. Ensure that they can see the chunks of pattern that will be repeated. If they can’t discern the equal-groups structure, they won’t be able to use multiplicative thinking to solve the problems. Making the patterns with beads and putting some questions on cards would make a good display that other students could use as an independent activity. Bead
patterns could hang from the ceiling with questions attached (for example, “What colour will the xth bead be?”), and other groups could discuss and record their ideas.
 

Answers to Activity

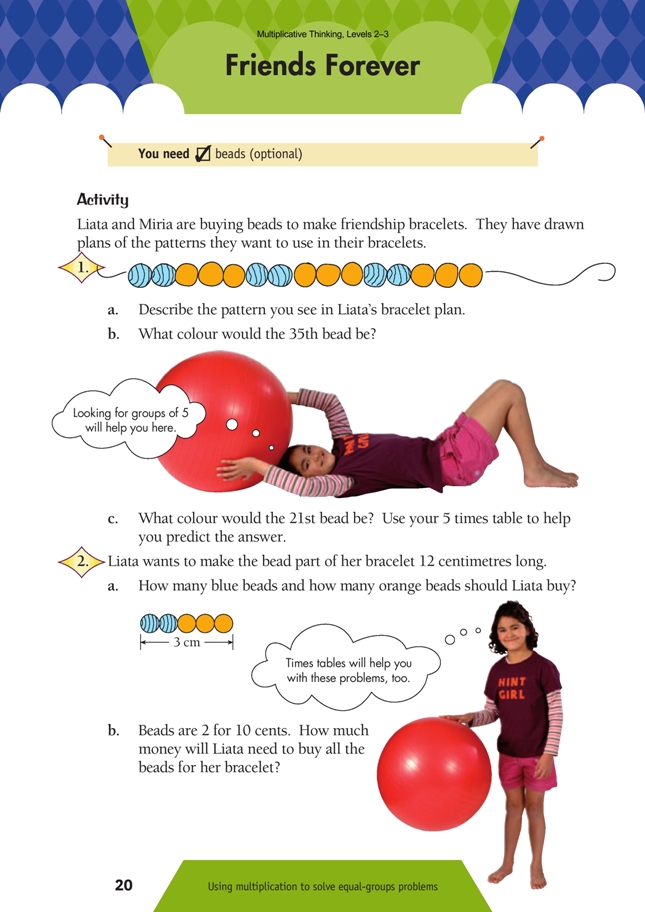
1. a. Liata’s pattern goes in groups of 5: each group has 2 blue beads then 3 orange beads.
b. Orange. (There would be 7 groups of 5, and the 35th bead would be the fifth bead in the seventh group of 5. The fifth bead in each group of 5 is always orange.)
c. Blue. (There would be 4 groups of 5 beads making 20, and the first bead in the next group of 5 would be the 21st one. The first bead in each group of 5 is always blue.)
2. a. 8 blue beads and 12 orange beads. To make a 12 cm string of beads, Liata will need to repeat her pattern 4 times. 4 x 2 = 8 blue beads, and 4 x 3 = 12 orange beads.
b. $1.00. 8 + 12 = 20 beads. 20 beads at 2 for 10c is 10 x 10c = 100c or $1.00.
3. a. Miria’s plan has shapes in groups of 3 (cube, cylinder, sphere [round bead]) and colours in groups of 4 (2 red beads and 2 green beads).
b. A red cube
c. The 20th bead would be green. The colour pattern repeats in groups of 4. 5 x 4 = 20. The 20th bead is the last bead in the 5th group of 4. The last bead in each group of 4 is always green.
d. The 3 times table, because the shapes repeat in groups of 3.
e. The 30th bead would be a sphere. 10 x 3 = 30. The 30th bead is the last bead
in the 10th group of 3. The last bead in each group of 3 is always a sphere.
4. a. 16 red beads and 14 green beads. Each group of 3 shapes is 2 cm long. There would be 30 beads altogether in a 20 cm string because 3 shapes x 10 repeats of the shapes pattern makes 20 cm = 30 beads. There are 2 red and 2 green beads in every 4 beads.  4 x 7 = 28, which is 14 red and 14 green beads. The last 2 beads, to make 30, are red. This makes a total of 16 red beads and 14 green beads.
b. 10. There are 10 lots of 2 cm in 20 cm, so there would be 10 spheres, one in each group of the shapes.
5. a. Practical activity
b. Answers will vary. You could use your 5 times table to help you. For example, if you have 2 triangle beads in your pattern and you repeat it 5 times, you could work out how many triangular beads you need by using 5 x 2 = .