This unit explores situations that involve multiplication and division using an equal sets model. Students learn to apply the properties of whole numbers under multiplication, to derive new answers from basic facts, and apply inverse operations to division.
- Derive from basic multiplication facts to solve multiplication problems with equal sets.
- Apply the commutative and distributive properties of multiplication to solve problems mentally and on paper.
- Recognise how both measurement and sharing division problems can be solved by ‘building up’ with multiplication.
In te reo Māori the word for multiplication is whakarea. Whaka means “to happen” and rea means “grow or make plentiful” like the offshoots of a plant. The word whakarea captures the scaling nature of multiplication, meaning the creation of many copies of equal sets.
The simplest form of multiplication problem involves finding the total of a given number of equal sets. Consider this problem:
There are eight cartons of eggs. Each carton contains four eggs.
How many eggs are there altogether?
The problem can be represented mathematically as 8 x 4 = □. Eight represents the number of sets (the multiplier). Four is the number in each set (multiplicand) and represents the unit rate of “four eggs per carton.” The x symbol represents “of” in the sense of connecting eight sets of four. The empty box is the product or total and the equals sign represents sameness of quantity or balance.
Division with equal sets takes two forms depending on which factor is unknown. The te reo Māori word for division is whakawehe which means “to make separation happen”. The separation into equal sets happens in two different situations.Sharing division comes for equally distributing a total number of objects, the dividend, into a given number shares (the divisor), which results in an amount per share (the quotient). For example:
There are 32 eggs and eight cartons of the same size.
How many eggs go into each carton?
Note that 32 ÷ 8 = 4 represents the sharing of 32 (the dividend) into 8 equal sets (the divisor) which results in a quotient of “4 eggs per carton.” Division also applies to measurement contexts such as:
There are 32 eggs. Four eggs go into each carton.
How many cartons are needed?
Note that the rate is known, “4 eggs per carton”, and that becomes the unit of measure. “How many fours are in 32?” answers the problem. That can be written as 32 ÷ 4 = 8.
Both equal sharing and measuring problems are common in the real world. Developmentally, students tend to build up solutions to these problems using addition at first, progressing to multiplication. With appropriate opportunities to learn, students later come to treat division as an operation in its own right.
The learning opportunities in this unit can be differentiated by providing or removing support to students and by varying the task requirements. Ways to differentiate include:
- providing physical materials so that students can anticipate actions and justify their solutions. Use materials like cubes and counters, and suitable collection vessels, such as clear plastic glasses or cut-down egg cartons, to model situations and connect strategies used by the students to the quantities that are represented.
- representing the place value structure of whole numbers using appropriate materials such as place value blocks or bundles of iceblock sticks.
- connecting symbols and mathematical vocabulary, especially the symbols for multiplication and division (x, ÷) and for equality (=). Explicitly model the correct use of equations and algorithms, and discuss the meaning of the symbols in context.
- altering the complexity of the numbers that are used. Multiplication with factors such as two, four, five, ten tend to be easier than factors such as three, six, seven, eight and nine. This classification is also true for divisors. Vary the size and place value structure of the multiplicands to make problems more accessible, e.g. 4 x 15 is easier than 4 x 14 or 4 x 17. A similar classification is true for the choice of dividends, e.g. 63 ÷ 3 is easier that 57 ÷ 3.
- encouraging students to collaborate in small groups and to share, and justify, their ideas.
- using technology, especially calculators, in predictive, pattern-based ways to estimate products and quotients, e.g., Is the answer to 57 ÷ 3 closer to ten, twenty or thirty? How do you know?
The context used for this unit is bacon and eggs, simply to provide everyday situations that students are likely to be familiar with. You may wish to change the contexts to situations more relevant to your students’ everyday lives, interests, or cultural identities. For example, eggs in cartons might become kumara in kete, players per team, rowers in a waka ama, or students in mini-buses. Encourage students to be creative by accepting a variety of strategies, and asking them to create their own problems for others to solve, in contexts that are meaningful.
- Calculators
- Linking cubes
- Empty egg cartons (dozens)
- Copymaster 1
- Copymaster 2
- Copymaster 3
- Copymaster 4
- PowerPoint 1
Session 1
- Begin with a growth problem (see PowerPoint 1: Eggs and chickens).
There are 12 eggs in one dozen.
How many eggs are in eight dozen?
What ways can you find to solve this problem?
Which way is the most efficient? (takes the least effort)
- Let your students work in small groups to answer the question. Provide place value materials such as connecting cubes, place value blocks, or iceblock sticks and rubber bands for students to access if they wish. Real empty egg cartons are very handy for later. Encourage students to rehearse the strategy they used so they are ready to share it with the whole class. After an appropriate time, bring the class together for discussion. Have eight egg cartons with cubes or counters as ‘make-believe’ eggs available to show what happens to the quantities.
Some students may use additive thinking. An example might be:
12 + 12 = 24 (That’s two cartons), 24 + 24 = 48 (That’s four cartons), 48 + 48 = 96 (Possibly using 50 + 50 = 100, that’s eight cartons).
You might record the strategy using multiplication, like this:
2 x 12 = 24 → 4 x 12 = 48 → 8 x 12 = 96
Repeated doubling can be a useful strategy for multiplying by 4, 8, and 16.
Additive thinking gets the job done but it is an inefficient process. Look for multiplicative strategies to highlight and share. These strategies might include:
12 is made up of ten and two. 8 x 10 = 80 (That’s the tens) and 8 x 2 = 16 (That’s the twos). 80 + 16 = 96.
Or (less likely):
10 x 12 = 120 (That’s two dozen too much) 2 x 12 = 24 (That’s two dozen)
120 – 24 = 96
Both strategies use the distributive property which means one factor is ‘distributed’. In this case 12 is distributed into 10 and 2, and 10 is distributed into 8 and 2.
- Use the egg carton model to show how each strategy works, including the repeated doubling. PowerPoint 1 can be used if you do not have the physical model of egg cartons. Pose this problem for the students to solve in small groups of two or three (See PowerPoint One). Let students have access to materials if they need them.
There are 15 rashers of bacon in each pack.
How many rashers are in six packs?
What different ways can you find to solve this?
- Look for students to:
- Apply multiplicative strategies rather than additive ones.
- Recognise which strategies are the most efficient.
- Record their strategies using equations or other written working.
- Show how their written recording can be represented with physical materials.
- After a suitable time, bring the class together to share ideas. Look for students to think critically about how a multiplier of 6, rather than 8, changes their use of strategies. Six does not lend itself to doubling only strategies though doubling then multiplying by three, or vice versa, works.
Good examples of using the distributive property are:
15 = 10 + 5, 6 x 10 = 60 and 6 x 5 = 30. Therefore, 6 x 15 = 60 + 30 = 90.
6 = 5 + 1, 5 x 15 = 50 + 25 = 75 and 1 x 15 =15. Therefore, 6 x 15 = 75 + 15 = 90.
An example of using the associative property is:
6 = 2 x 3, 2 x 15 = 30, 3 x 30 = 90. Therefore, 6 x 15 = 90.
- Ask students to show what each strategy looks like with materials. Place value-based models are particularly good for this problem. If time permits take each problem (Eggs and Bacon) and change the numbers. For example, you might create a tray of 24 eggs. How many eggs are in three trays?
Session Two
- Remind your students of problems they solved the session before. You might show them some examples as a reminder.
What was the same about these problems? What was different?
- Students should note that while the stories and numbers were different there was a common structure to the problems they solved. That structure involved a collection or set of some kind, like a packet, and a multiplier that gave how many of the collections were considered. Take one problem and ask your students:
Where is the collection or set in this problem?
Where is the multiplier?
- Provide a different problem and ask the same questions. You might use:
Eighteen hens lay four eggs each in one week.
How many eggs is that in total?
Be aware that students might think that the multiplier is always less than the size of the equal collections. Share some strategies for solving 18 x 4 = 72.
- Pose this challenge which involves computational thinking.
Imagine you trained a robot to solve problems like these ones. Make up a set of instructions for the robot. What actions should the robot take? Put the actions in order.
- Let your students work in small teams to devise a set of instructions. Look for them to:
- Define the equal collections and multiplier as factors, with some criteria
- Record the factors and steps symbolically
- Follow a sequence of instructions that work to give the correct product
- The aim of the follow-up discussion is to create an algorithm, a set of steps that will reliably produce the answer. Act out some instructions that students provide with the example problem. Have place value materials available to check what is going on with quantities as the steps are followed.
You might end up with a sequence that looks like this:
Step 1: Find the number of things in the equal collections, call that number [c]
Step 2: Find the number of equal collections, call that number [n]
Step 3: Multiply [n] and [c] by:- Breaking up [c] into the number of tens [a] and ones [b]
- Multiplying both [a] and [b] by n
- Add the two answers, n x a and n x b, together
- Challenge your students to adapt their algorithm to solve this problem in small groups (see Copymaster 3):
There are 144 eggs on each pallet.
You have 4 pallets. How many eggs is that altogether?
- Provide place value materials to support students to work out the product. Look for students to:
- correctly establish 144 as the multiplicand - the size of the equal collections
- correctly identify 4 as the multiplier
- recognise that 144 contains hundreds as well as tens and ones
- multiply hundreds, tens and ones separately then add the partial products.
- Ask how the set of procedures needs to be changed to accommodate the new problem. Do students recognise the two steps that need minor change?
Be open to other ways that students might solve 4 x 144, particularly repeated doubling, i.e. 2 x 144 = 288, 2 x 288 = 576. You might try writing an algorithm for the doubling strategy. That will require a first step of deciding whether, or not, the numbers are suitable.
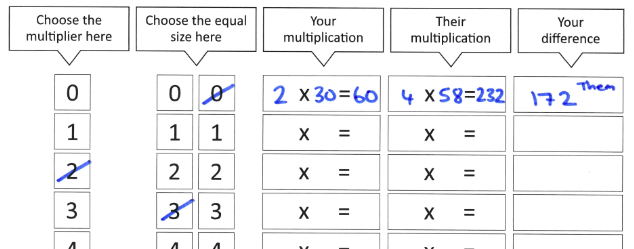
- Finish the session with Copymaster 1 that is a paper game for practising multiplication of a two-digit number by a single digit number. Some students may need a calculator to support their participation.
The rules are:
Players play in pairs. Each person has their own sheet of paper (Copymaster 1)- At the same time they choose two factors by crossing off digits. The first factor is single digit and the second factor has two digits. Players do no know what digits their opponent is choosing.
- Players multiply their factors and record the product on the game sheet.
- Both players compare their products. The player with the largest product wins the round. They get the difference between their product and their opponent’s product.
- The player with the highest total of differences wins.
Here is an example of a partly completed scoresheet.
Session Three
In this session students transfer the strategies they developed for multiplication to problems involving division. Understanding that asking themselves the question, “How many x’s fit in y?” structures division problems and supports students’ fluency in calculation. By connecting division to multiplication students learn to apply their multiplication strategies and connect multiplication and division as inverse operations.
- Begin with this division problem:
Henrietta gathers 52 eggs one morning.
How many half-dozen cartons (six-packs) can she fill?
- You may need to clarify with the students that a half-dozen carton holds six eggs. Let the students explore the problem in small teams, using materials for support, if needed. Ask your students to record their strategies to share with the class. Do your students…?
- Use additive thinking to ‘build up’ to a solution, e.g. 6 + 6 = 12, 12 + 12 = 24, 24 + 24 = 48, leaves 4 eggs to make 52.
- Use multiplicative ‘build up’ strategies, e.g. 5 x 6 = 30 so 6 x 6 = 36 … 8 x 6 = 48.
- Use the distributive property of multiplication, e.g. 10 x 6 = 60 (too many eggs) so 9 x 6 = 54 and 8 x 6 = 48, therefore 8 six-packs are possible with 4 eggs remaining.
- Apply basic facts knowledge, e.g. 8 x 6 = 48 and 9 x 6 = 54 and 52 lies between the two products.
- Pay attention to how students handle the remainder of 4 eggs.
How much of a six-pack are four eggs? (two-thirds)
- Gather the class to share strategies. Choose teams that use strategies representative of the points above. Discuss efficiency. Which strategies require the least work?
- Introduce division equations in the context of the eggs problem.
52 ÷ 6 = 8 r4
- Make the meaning of the symbols explicit , i.e., equals as a sign of balance, division as meaning “measured in units of”, 52 as the dividend (total items to be measured), 6 (unit of measure), 8 (number of equal units that fitted), and r4 as the ‘leftovers’ or remainder. You might discuss other ways that the remainder might be recorded, i.e. 4/6 of a carton, 0.66… of a carton.
- Pose the following ‘bits missing’ problem. Let the students make up the missing data in the problem, i.e., number of eggs collected, and size of the carton.
Henrietta gathers __ eggs one morning.
How many __ cartons can she fill?
- Encourage the students to solve more than one problem with a focus on this question:
How do your strategies change as the numbers in the problem change?
Observe how students approach the task. Challenge students who ‘play safe’ to try more difficult numbers.
What numbers do you think would make the problem more challenging?
Why do the problems become more difficult with those numbers?
What strategies do you use with more challenging problems?
- After a suitable time gather the class together to process their problems. Categorise the problems as easy, medium and hard.
What makes a problem easy? (small numbers, no remainder, access to basic facts)
What makes a problem hard? (bigger numbers, remainders, tricky divisors such as three or seven, or outside the range of basic facts)
- Choose one hard problem and ask the students to solve it. Discuss what knowledge might be handy for solving it?
How will we use that knowledge?
- Make a list of strategies such as distributing the dividend, rounding up to a tidy number, halving, etc.
Session Four
- Recap the previous session using examples of the problems that students created. Record the problems using division equations, e.g. 72 ÷ 4 = 18. Ask students to be explicit about what the symbols represent in the equation.
What is the same about these problems? (all the problems are about measurement, i.e. How many x’s fit into y?)
- Tell the students that they will solve a different type of division. Pose this problem:
Three hens laid 48 eggs altogether over two weeks.
Each hen laid the same number of eggs.
How many eggs did each hen lay?
- Let students solve the problem in small teams. Allow them to use materials if needed. Encourage students to record their strategies for sharing later. Look for students to:
- Recognise that the problem involves sharing the eggs into three equal sets.
Note that the problem semantics suggest multiplication, i.e. 3 x □ = 48. - Use efficient build up strategies that involve multiplication, e.g. 3 x 10 = 30 (18 eggs left), 3 x 5 = 15 (3 eggs left), 3 x 1 = 3, so each hen lays 10 + 5 + 1 = 16 eggs.
- Possibly use place value with tidy numbers, e.g. 60 eggs would mean 20 eggs each, 48 is 12 eggs less so each hen gets 4 eggs less than 20.
- Recognise that the problem involves sharing the eggs into three equal sets.
- Gather the class to share strategies. Look for a team of students that work the problem out as “How many threes are in 48?” If no students do that then introduce the idea as your strategy.
Can someone explain how that works? The problem is about sharing not measuring.
- You may need to act the problem out with materials, like cubes, to support students see the connection between the two forms of division. Deal out ‘eggs’ to three students acting as ‘hens’, pausing each time around is complete.
How many eggs have I shared so far?
Students should notice that three eggs are used up each time a round of dealing is complete.
Why are three eggs used up each time?
- Provide your students with Copymaster 2 which contains variations on the first problem. They can solve the problems individually or in small teams. Encourage them to use their multiplication facts and ask, “How many x’s are in y?” thinking.
Session Five
In this session students explore an open-ended problem that allows them to demonstrate their multiplication and division strategies. The problem requires them to impose or find some information, i.e., the number of hens, how many eggs hens lay per week, and the size of the trays. Invite them to research online if they need to and use their knowledge of everyday context, e.g., trays that eggs commonly come in.
- Use Copymaster 4 to introduce the problem and allow students to work on the task independently. Insist that they show their working clearly so someone else can see their reasoning.
On Happy Chook poultry farm there are about 80 free range hens.
Each Saturday all the eggs for the week are sold at the Farmers’ Market.
How many trays are needed each week?
- After a suitable time put the students in pairs to compare their work. Ask some reflection questions such as:
Was there enough information to solve the problem?
If not, where did you get your information?
Were your strategies efficient?
How might you have solved your problem differently?
- Gather the work samples as evidence of your students’ current thinking about multiplication and division.
Dear family and whānau,
This week at school we are learning to solve multiplication and division problems. The problems are all about eggs with a little bacon.
Here is an example:
Henrietta has 72 eggs that she wants to put into six-packs.
How many packs can she make?
We encourage children to use multiplication knowledge rather than adding on because multiplication is more efficient. For example, it is more efficient to work out how many sixes are in 72 than to keep adding six until 72 is found.