Pipe Music with Decimals

Background Maths

Students who are at the Advanced Additive stage are developing a range of multiplicative strategies. Multiplicative thinking is essential for the understanding of equivalent fractions and ratios. Understanding that 9/12 is the same quantity as 3/4 involves seeing that the multiplicative relationship that exists between 9 and 3 also exists between 12 and 4.

This is based on knowing that three-twelfths is the same as one-quarter.The equivalent fractions concept is essential for an understanding of decimals and percentages. For example, 1 1/4 equals 125/100, which is 1.25.Decimals and percentages are special cases of equivalent fractions where thedenominators are restricted to powers of 10 (10, 100, 1 000, etc.).

Students must come to understand the idea of continuity in the number line. Through early experiences, the students know the sequence of whole numbers. This knowledge has to be reconfigured to include an infinite set of numbers called fractions that coexist with whole numbers.

Using Materials

Show the students a “one” pipe on the floor and say you want them to consider this as “one” for today’s lesson. Hold the pipe up to a whiteboard and use it to make a number line between zero and one.

Write the numbers 3 and –2 on the board and ask the students where three and negative two would be on this number line. This is to help students recognise how this number line fits with their existing view and consolidate the acceptance of the pipe as “one”. Students should recognise that three will be three pipe lengths to the right of zero.

Lay the “one” pipe down and show the students a “one-tenth” pipe. Comparing the two pipes, ask them, “If this is “one”, how much do you think this is?” Let the students discuss what fraction they think the newest pipe is. For those who think it is one-tenth ask them, “What is the smallest number of these pipes I would need to join to confirm that they are one-tenth pipes?”

Some students will say that five one-tenth pipes should be half of the length of the one pipe. This can be checked by threading five one-tenth pipes onto a piece of dowelling and aligning it to the one.

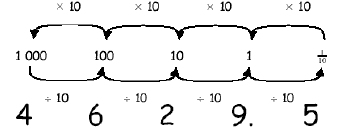
Ask the students, “What is the decimal for one-half?” Most will know this as 0.5, but many will not be able to explain what the five represents.Write a four-digit whole number on the board to develop the idea of decimal places to the right of the ones (for example, 4629.5). The next place to the right is found by dividing the previous place by 10 and a decimal point is used to mark the ones place.

So the place to the right of the ones is the tenths (1 ÷ 10 = 1/10

). Discuss the extension of further places to the right, hundredths, thousandths, etc. Note that the system is infinite.

Return to the pipe model. Ask a student to show you what one-quarter would look like if made with the pipes. Look for the students to realise that one-quarter cannot be made with a whole number of tenths. Often they will say that one-quarter is two and a half tenths, which is correct.

Comment that the calculator does not show 0.2 1/2. Ask them what the calculator does show. Some will know that the decimal for one-quarter is 0.25.Use this to extend the place value diagram to hundredths. Ask the students to show you with their forefinger and thumb how long one hundredth will be.

Produce the hundredths pipes and ask a student to show one-quarter as a decimal by threading pipe pieces onto a length of dowelling. This can be compared to the one half already made.

Note that 0.25 is two tenths and five hundredths, which is also 25 hundredths. Ask the students what the decimals for 2/4 (0.5), 3/4 (0.75), 4/4 (1.0), 5/4 (1.25), and 6/4 (1.5) would be, noting that equivalent fractions have the same decimal and that, like fractions, decimals can be greater or less than one.

Put the students into pairs and have each pair build one of the decimals below using pipes:

0.37 0.4 1.2 0.365 2.09

Each decimal brings out different considerations of the decimal system. Write 0.37 and 0.4 on the board and ask the students which decimal they think is larger. Look out for whole-number thinking, as in, “0.37 is bigger because 37 is bigger than 4.”

Also be aware that students may say 0.4 is bigger on the basis of the relative size of tenths and hundredths without attending to the place value. They may say, “0.4 is bigger because it has 4 tenths and 0.37 only has 3 and tenths are way bigger than hundredths.”

Align the pipe models of these numbers and ask the students how much needs to be added to 0.37 to make 0.4 (three hundredths). The students should recognise that 10 hundredths make one tenth.

Making 1.2 and 2.09 will further consolidate the place of one as the students will need to access ones pipes to model these numbers. It is interesting to ask the students where these decimals lie in the original 0–3 number line. Focus on potential problems with 2.09 as students may use nine tenths instead of nine hundredths and highlight the significance of zero in holding the tens place.

0.365 shows the need for thousandths, and the students should be asked to show how large they think one-thousandth might be by indicating with a gap between thumb andforefinger.

Using Imaging

Pose addition and subtraction problems that can be modelled with the pipes. Fold back to using the pipes where students need support. Otherwise encourage them to image what the models might look like.

Problem examples might be:0.4 + 0.13 = 0.53

Note that whole number thinking students might get 0.17 rather than adding tenths then hundredths.

1.6 + 2.7 = 4.3

Note that whole-number thinkers might get 3.13 rather than recognising six tenths and seven tenths gives 13 tenths, which is one and three tenths.

0.56 – 0.3 = 0.26

Note that whole-number thinkers might get 0.53.

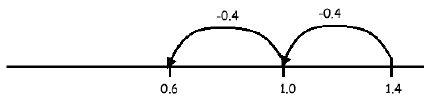
1.4 – 0.8 = 0.6

Look for application of whole number strategies across the decimal point, like reversibility, e.g., 0.8 + = 1.4, = 0.6, or decomposition, e.g., 1.4 is 14 tenths, 14 tenths less 8 tenths is 6 tenths.

Capture students’ responses on empty number lines. For example:1.4 – 0.8 as: 1.4 – 0.4 = 1.0, 1.0 – 0.4 = 0.6.

Using Number Properties

Pose more complex addition and subtraction problems with decimals. Look for the students to apply the same mental strategies to decimals that they use for whole numbers. Encourage the students to use empty number lines to describe their mental strategies. The examples below suggest possible strategies:

2.7 + 3.09 = 5.79

Using place value: 2 + 3 = 5, 5 + 0.7 = 5.7, 5.7 + 0.09 = 5.79
4.6 + 1.95 = 6.55

Using tidy numbers: 4.6 + 2 = 6.6, 6.6 – 0.05 = 6.55
4.18 – 2.9 = 1.28

Using tidy numbers: 4.18 – 3 = 1.18, 1.18 + 0.1 = 1.28
7.36 – 6.8 = 0.56

Using reversing: 6.8 + 0.2 = 7.0, 7.0 + 0.36 = 7.36, 0.2 + 0.36 = 0.56
0.675 – 0.49 = 0.185

Using tidy numbers: 0.675 – 0.5 = 0.175, 0.175 + 0.01 = 0.185