Effective teachers select tools and representations of mathematical concepts that are transparent, meaningful, and functional for students. Tools and representations include mathematical words, symbols, stories/metaphors, pictures/diagrams such as graphs, physical materials, and technology.
Guidance for effective practice:
- Use materials to support ākonga to represent and work with mathematical concepts and procedures.
Some students in Joel’s Year 1 class are learning to count, read, and say numbers to 20. Their one-by-one counting is getting reliable. Joel wants them to understand that adding one more to a set results in the next number in the counting sequence, e.g. If one is added to six objects, then there are seven objects.
Joel gives each student a number strip and a collection of counters.
Joel: Can you please count five red counters, and hold them in your hand?
The students all count five counters.
Joel: If you put the counters onto the strip like this … (one at a time, starting at one) … what number will the last counter cover?
Some students seem to think the answer is obvious, but others need to place the counters to check.
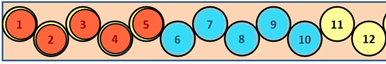
Joel gives each student one counter of a different colour, and he asks, “If you put that counter on your strip, how many counters will you have then?”
All the students say, “six”, realising that the 6th circle will be covered.

He gives his students two more examples, eight counters, and one more, and 12 counters and one more. On the last example, Joel gets the students to turn over the strip so they cannot see the numbers (masking).
Next students put the number strip behind them and solve other ‘one more’ problems without it.
Joel: Get ten red counters for me. Put them in your hand and close it. If I give you one more counter how many will you have? If I give you one more again, how many counters will you have?
Joel: What do you think happens if I take one counter away instead?
Joel supports his students to visualise the result of actions on materials, through gradual withdrawal of those materials.
- Develop the diagrammatic and symbolic literacy of your ākonga to represent and work with mathematical concepts and procedures.
Mel downloads the teaching unit Getting Partial to Percentages from nzmaths. She uses the percentage download bar for computer files to represent percentage problems throughout the unit.

Mel uses the words rate, base, and amount in conjunction with the bar model, and supports her students to use that vocabulary to explain their strategies.
Her students become adept at using the percentage bar to support their problem solving across a variety of types, depending on whether the rate, base, or amount is the unknown. For example, Ofa creates this diagram to find [ ]% x 24 = 18.
