In this unit students find out the length of their pace when walking and running, and compare these with the paces of others.
- Make estimates of lengths between approximately 50cm and 1.5m (lengths of strides)
- Measure lengths in metres and centimetres
- Convert between metres and centimetres.
In this unit the students use tape measures to measure lengths in centimetres, in metres and other decimal parts of a metre. The metre is the base unit of length in the International System of Units (SI).
Longer units of length are created by collecting multiples of 1 metre. The most common unit is the kilometre. Kilo is the prefix for 1000 so 1 kilometre is equal to 1000 metres.
Shorter units of length are created by partitioning 1 metre into equal parts. For example, centi is the prefix for one hundredth, and milli is the prefix for one thousandth. Therefore, 1/100 of 1 metre = 1 centimetre and 1/1000 of 1 metre = 1 millimetre.
The symbols for units of length reflect the basic unit, m for metre, and the prefix. For example, μm is a micrometre since micro is the prefix for 1 millionth.
The learning opportunities in this unit can be differentiated by providing or removing support to students, by varying the task requirements. Ways to support students include:
- providing measurement tools so students can develop the practical skills required
- physically acting out the iteration of stride lengths
- providing calculators to support difficult divisions and conversions
- promoting collaborative group (mahi tahi) work with sharing and justification of ideas
- explicitly modelling the relationships between units of length, e.g. dividing a metre strip of paper into tenths of tenths to show centimetres
- linking division with decimals to division with whole numbers, e.g. “How many fives fit in 30?” so “How many lots of 0.5 metres fit in 3 metres?”
Tasks can be varied in many ways including:
- manipulating the accessibility of the distances being measured
- reducing recording required, through the use of tables and other templates
- encouraging flexibility in the way students choose to solve problems.
The contexts for this unit can be adapted to suit the interests and cultural backgrounds of your students. For example:
- using distances that are familiar to students, such as the length of, or distance between, sports fields, buildings, playgrounds, and their marae.
- measuring the length of trips they commonly make, such as to a friend or relative’s house, to their parent’s workplace, or to a favourite recreation site
- investigating how people's measures were used in the past, such as indigenous Māori using their arms to measure the girth of tall trees for the purpose of making waka or how buildings were constructed using personal measures, like stride or foot length, of assigned people. They were usually a significant high ranking chief. In some areas raura (flat plaited cord or durable timber) were used with the stick passed down through generations as a taonga.
Te reo Māori vocabulary terms such as ine (measure), mita (metre), and mitarau (centimetre) could be introduced in this unit and used throughout other mathematical learning.
- Tape measures, metre rulers, trundle wheels
- Calculators
- Access to Google Maps
- Pedometers (optional)
Session 1
LO: Finding the length of one’s stride.
- Ask students to estimate the length of their stride. Clarify that a stride is a ‘stretched out’ pace or step. Ask for suggestions as to how we could find the length of our normal walking stride. Introduce the idea of a people’s measure - as was used by early Māori peoples. An internet search for “Inenga. Māori Measurement Using Your Body” reveals information that could be shared with students. You could invite all students to share ways of measurement that are relevant to their cultural backgrounds.
- In pairs, have all the students measure the length of their walking stride in two ways:
Method 1: Measure out ten metres. One student walks ten metres while the other counts the number of strides, expressing the last part-stride as a fraction or decimal, for example ‘about 14.5 strides’.
How will you work out the average length of one stride? (Divide 10 metres by the number of strides),
My calculator says, 10 ÷15 = 0.66… What does that mean? (66.6… centimetres)
How will you record the measure of one stride? (67cm or as a decimal of 1 metre, e.g. 0.67m rounded)
Ask students to record their stride length in two ways; for example, ‘0.69m, 69 cm.’
Method 2: Walk 10 strides, and measure and record the total distance..
Should we start/finish measuring at the heel or toe of your foot? Why is consistency important?
Suppose I walked a total of 7 metres and 60 centimetres. How will I calculate the distance of my average stride? (Express the distance using one unit, e.g. 7.6m or 760cm then divide that distance by 10.)
Record the stride length in two ways; As metres and centimetres, for example “My stride averaged 0.6.8m or 68cm.”
These data could be entered in a table, either digitally or as a hard-copy:
| Total | Average length of stride (m) | Average length of stride (cm) | |
| 10 metres | 14.5 strides | 0.69m | 69cm |
| 10 strides | 6. 80 metres | 0.68m | 68cm |
Comment: I estimated my stride would be about 80cm. It was shorter than I expected. My average stride is about 68-69 cm long. My measurements using both methods were within 1 cm.
- Discuss the different results and the methods used.
Were your two results similar, or very different? Why?
Were your initial estimates quite accurate or not? Why? - It is important to discuss sources of measurement error, such as going to the nearest mark, misreading the scale, converting between units, using a different measurement baseline (heel or toe), variation in walking style, etc. This could be modelled for the class.
Which method do they think is more reliable? why?
How could we test out the accuracy of our average stride length?
Students might suggest measuring a larger distance and matching the result with expectations from the stride measure.
Session 2
- In this session, students test the reliability of their stride length estimate. They do this by predicting the number of strides needed to measure a known distance, measuring that distance by pacing, and comparing the predictions with the results.
- Establish some trusted point-to-point journeys within the school. Students might use trundle wheels, tape measures, or metre rulers to measure those lengths in metres. For example, the length of the rugby field equals 75 metres or the longest wall of the hall equals 36 metres. Google Maps reduces to a scale of 5 metres so students might use that tool to find measures or confirm their own measurements.
Ask: If your stride length is consistent, how many strides will it take you to measure these distances?
For each distance, create an estimate and record it in a table, as below. Allow the use of calculators as appropriate to the needs of your students.Distance Measurement (in metres Predicted number of strides Actual number of strides Length of rugby field 75m Longest side of hall 36m Perimeter of junior playground 57m Etc.
Do students use the correct operation to calculate the number of strides? (Note that the number of strides should be greater than the number of metres. Why?)
Do students convert the distances and their strides to the same unit, metres or centimetres?
- Discuss the operations students use. Do they use division, e.g. 5700 ÷72 = 97.22?
Do they recognise that more strides than metres fit into the same space?
Do they interpret the answer in correct ways? (strides, not metres or centimetres) - Send the students out in smaller teams to test their predictions. Ask them to fill in the actual number of strides for each distance.
- After completing the task, gather your class and discuss:
How accurate were your predictions?
What might cause inaccuracies? (Miscounting, variable stride length, etc.)
How reliable is your stride length for measuring distances?
Could you build a path using strides instead of metres as your unit? - Ask: How many strides would you take walking from home to school?
How would you predict the number of strides?
You might go to Google Maps, locate a hypothetical address for “Manu from Room 67”. Get directions to the school and that will display the walking distance by shortest routes.
Suppose Manu lives 1.3 kilometres from school. His stride length is 60 centimetres. How many strides will he take? - Let students use calculators. What is the correct operation to perform?
1300 ÷0.6 1300 x 0.6 1.3 ÷ 60 1.3 x 60
130 000 ÷60 130 000 x 60 - Discuss the need to express both the total distance, 1.3 km, and the stride length, 60 cm, in the same unit. Since 1.3 km equals 1300 metres it must also equal 100 x 1300 = 130 000 cm.
You might need to support students to realise that “How many 60s fit into 130 000?” is a division problem, so 130 000 ÷ 60 = 2166.67 is the correct answer. Manu would take almost 2167 strides. - Let students calculate the number of strides they would take between home and school using Google Maps to measure the total distance, and their own calculation for stride length. Ask your students to record their calculations using correct units of measure.
Session 3
In this session students consider the impact of varying the unit of length. They encounter inverse proportional situations in which increasing the length of the unit results in a decrease in the number of units, in a given space.
- Ask: Do taller students have longer strides?
What is your prediction? Why? - Ask students to measure each other’s height, in pairs, and enter all heights and stride lengths on a class chart or spreadsheet.
- How might we display the relationship between height and stride length?
You may need to suggest that a scatterplot is the best way to display these data, as both measures appear on a single graph. Create a scatterplot using chosen spreadsheet application (e.g. Google Sheets, CODAP, Microsoft Excel) or on paper.
Example of scatterplot: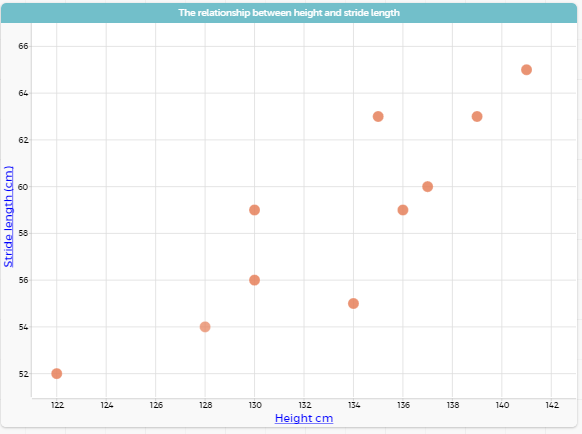
- Ask: Is there a relationship between height and stride length?
How can we tell?
Students might notice that taller students tend to have longer stride lengths. - Ask: Could you use this graph to predict the stride lengths of younger/shorter and older/taller students? How?
- Ask pairs of students to draw their own scatter graphs of heights and stride lengths for the students in your class. Ask them to write a comment explaining what the graph shows.
- Measure the length of the classroom, e.g. 10 metres. Choose two students whose stride lengths are tidy fractions of each other. For example, Mere’s stride length equals 60cm and Stefan’s equals 75cm.
Who will take the most strides to measure the length of our classroom?
Why will Mere take more strides?
Let’s get Stephan to measure. How many strides do you expect him to take?
How would we calculate that? (1000 ÷75 = 13.33 so 13 and 1/3 strides)
Let Stephan act out his measurement by strides.
If we know Stephan takes a bit over 13 strides, how many strides do we expect Mere to take? - Invite estimates. Some students will try to calculate using Mere’s stride length, e.g. 1 000 ÷60 = 16.67 so almost 17 strides. Other students might attempt to use inverse proportions intuitively. For example, Mere takes 5 strides to cover 3 metres and Stephen only takes 4 strides. There are more than three lots of 3 metres in the length of the classroom so Mere should take at least 3 more strides than Stephan (The actual answer is 5/4 of 13.33 = 16.67).
- Pair up the students and give them a point to point distance to measure in strides. Ask them to record the results and why one person took more strides than the other.
Session 4
Collect data about the stride lengths of other students, adults or animals, depending what resources are available. The following possibilities could provide rich tasks:
- Mark out ten metres and ask one or two teachers, parents, or other available adults to walk the distance while the class first estimates, then counts strides, and calculates the length of their stride.
- Consider stride length if students run rather than walk.
- Students may be able to find the stride length of their pet cat or dog (Warning that counting of strides will not be easy!
- Video from online will provide examples of athletes running over 100 metres or other distances. Videos of athletes like Usain Bolt often have slowed down footage that allows counting the number of strides. What is the athlete’s average stride length? Is the stride length longest at the start, end or middle of the race? (Over the 100m top speed is reached in the middle of the race). Do marathon runners have the same stride lengths as sprinters? Students are likely to be surprised at how far out their estimates of stride length are. Videos are also available of animals, like cheetahs and horses, running over a set distance, or swimmers (count the number of strokes and calculate the length between strokes), rowers or kayakers.
- Work out how many strides an athlete, horse or giant, would take from your home to school.
- Use stride lengths to measure speed. How many strides do you take in a minute? How long will it take you to walk home from school? How long would a cheetah take?
Session 5
After a class discussion of results, students can write up a detailed account of what they have found out during the week and suggestions for further investigations. These could be used to direct further lines of inquiry or could be displayed to show other classes or parents.
Dear family and whānau,
In mathematics this week we are measuring and comparing the lengths of our strides. It would help our investigation if your child could check how many of your strides fit into ten metres? Other family members could be counted as well. Your child will need to record the information they collect and bring the homework back to school.