A Sizeable Problem

This imaginative scenario illustrates an important but often neglected principle related to increase and decrease, that is, if you increase an amount and then decrease it by the same percentage, you don’t get back to the original amount. The same principle is at work every day in GST calculations: add 1/8 (12.5%) or multiply by to include GST; subtract 1/9 (11.1%) or multiply by to remove GST.
Check that your students can express the percentages on the buttons as their fraction or decimal equivalents. They may need to be told that the 67% and 133% buttons are rounded percentages but represent the fractions 2/3 and 4/3 respectively.
Discuss how an amount or size is changed when it is multiplied by a percentage less than 100% and more than 100%. The effect is exactly the same as when multiplied by a fraction less than 1 or more than 1.
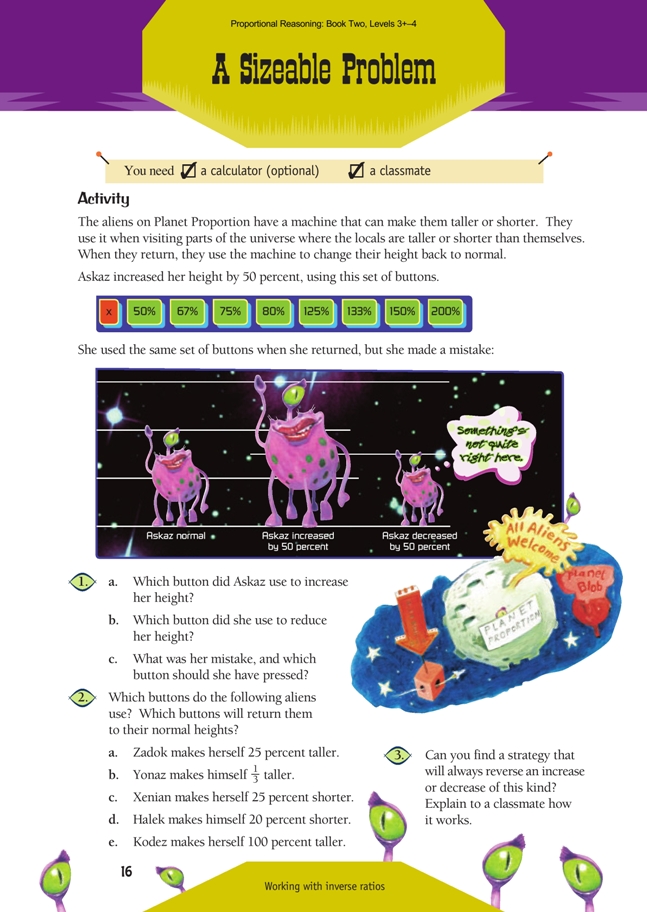
Question 1 is carefully designed to clarify the principle discussed in the introductory paragraph above. Put the students into mixed-ability problem-solving groups (no more than four in a group). Get them to work through the question together and be prepared to discuss their thinking in the wider group.
Some students may find it difficult to get their head around question 1: they are not told what Askaz’s height is, so how can they increase it by 50%? If this is a problem, suggest that they make Askaz 100 cm high. (Any height will do, but 100 is easy to work with.) Demonstrate this height with the help of a metre rule or tape measure, and then increase it by 50% to 150 cm. Then reduce her new height by 50% and show that it is 75 cm, not the original 100 cm.
Ask “What fraction of the new height is the original height?” This can be written:
orginal height/new height = 100/150 = 2/3. So the students need to multiply 150 by 2/3 to get back to the original height of 100 cm.
Now bring together the ratio for increase and the ratio for decrease so that the students can see how they are related: “To increase the original height by 50%, we multiply it by 1150/100 or 3/2. To get back to the original height, we multiply the new height by 100/150 or 2/3.” Height x 3/2 x 2/3 = height.
Have the students examine this multiplication and see that 3/2 x 2/3 = 6/6 = 1.
Before your students try question 2, they should have a clear understanding of whether the fraction they are looking for will be greater or less than 1 and should understand that these fractions can equally well be expressed as percentages greater or less than 100%. A fraction and its percentage are mathematically identical: they only look different.
Question 3 brings together the ideas developed earlier. Students should now be able to explain why multiplying by the inverse fraction returns a size or an amount to its original state: “Multiplying by a pair of inverse fractions is the same as multiplying by 1. Multiplying by 1 leaves what we started with unchanged. Each of the inverses ‘undoes’ or ‘neutralises’ the effect of the other.”
Two fractions that multiply together to make 1 are often called reciprocals. Reciprocals always come in pairs. It doesn’t matter which is named first; each is the reciprocal of the other: 3/4 and 4/3, 5/2 and 2/5, and 10 (10/1 ), 7/6 and 6/7 , 125% (5/4) and 80% (4/5).
You could ask your students why there is no button with 100% on it. 100% is the percentage equivalent of 1. (100% = 100/100 = 1) There is no need for a 100% button because multiplying by
100% or 1 has no effect whatsoever.

Answers to Activity

1. a. The 150% button
b. The 50% button
c. Askaz thought that increasing her height by 50% then decreasing the result by 50% would get her back to her normal height. But 50% of her increased height returns her to just 75% of her normal height. She should have pressed the 67% (2/3) button.
(150% x 67% = 100% or 3/2 x 2/3 = 1)
2. a. The 125% button and the 80% button.
(125% x 80% = 100% or 5/4 x 4/5 = 1)
b. The 133% button and the 75% button.
(133% x 75% = 100% or 4/3 x 3/4 = 1)
c. The 75% button and the 133% button.
(75% x 133% = 100% or 3/4 x 4/3 = 1)
d. The 80% button and the 125% button.
(80% x 125% = 100% or 4/5 x 5/4 = 1)
e. The 200% button and the 50% button.
(200% x 50% = 100% or 2 x 1/2= 1)
3. There is only one strategy, and that is to multiply by the inverse. But this strategy can be explained in different ways and using different examples.
Here is one explanation:
If Yonaz increases his height by 1/3, his height is multiplied by 1 + 1/3 = 4/3. To get back to his normal height, he needs to multiply his (increased)
height by the inverse of 4/3, which is 3/4.