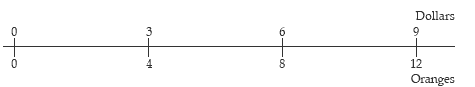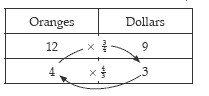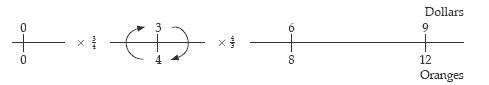Solve problems involving rates.
Number Framework Stage 8
Using Materials
Pose this problem to the students:
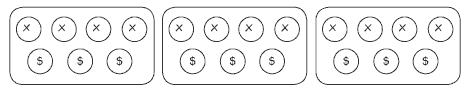
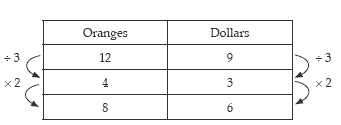
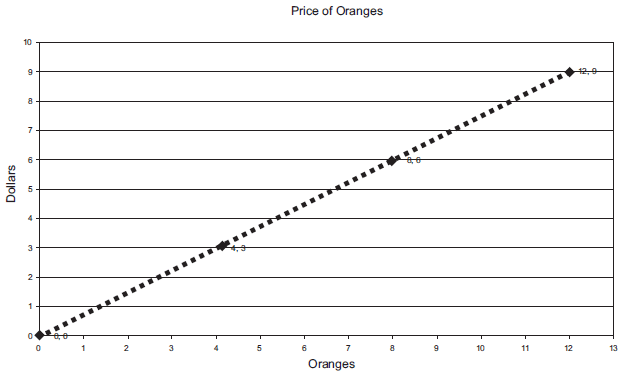
“You can buy twelve oranges for nine dollars. How much will you pay for eight oranges?”
Represent the problem in these four ways:
1. Equipment
2. Table
3. Graph
3. Double number line
The most common strategy employed by students is likely to be based on common factors (see the table on the previous page) because it avoids having to work between the measurements. It is important for students to have a variety of approaches for solving problems such as these. Ask them “How many oranges do you get for one dollar?” This gives the unit rate of oranges per dollar. Some students may observe that this unit rate can be found by dividing 12 by 9 because there are nine dollars. They may be surprised to find that the rate obtained on the calculator (1.333 oranges per dollar) is 1 1/3, which is 4/3.
Remind them that in algebra, 12 ÷ 9 = 12/9 = 4/3.
Ask the students, “What is the price of one orange in dollars?” Some students may work out that this can be found by dividing $9 by 12 because there are 12 oranges in the given rate. Remind them that 9 ÷ 12 = 9/12 = 3/4.
Ask them to predict the calculator result (0.75 dollars per orange). Ask how this rate could have been used to find the cost of eight oranges (8 x 0.75 = 6 or 8 x 3/4 = 6). Connect the dollars per orange rate to the slope of the line connecting the ordered pairs on the graph. For movement of one unit to the right (one more orange), the line increases 0.75 units up (3/4 of a dollar)
Tell the students that 3/4 and 4/3 are known as reciprocals, i.e., fractions with transposed numerators and denominators. Ask, “What is the answer to 3/4 x 4/3 ?” (one).
Show these operators on the table and the double number line:
Pose similar problems with the expectation that students will connect the equipment, table, graph, and double number line representations. Suitable problems might be:
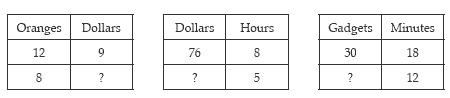
“Lisa worked at the bookshop. She earned a total of $76 for working 8 hours. How much would she earn if she worked for 5 hours?” (Answer: $47.50)
“The machine made 30 gadgets in 18 minutes. How many gadgets would it make in 12 minutes?” (Answer: 20 gadgets)
Discuss what the problems have in common. (They are all rate problems because they involve comparing two different types of measurement.) Connecting the tables will help the students to see this structure.
Note that the unknown may appear in any cell within the table and this, as well as the numbers involved, may make a big difference to the difficulty of the problem.
Using Imaging
In this phase of the lesson sequence, students are required to anticipate how eachrepresentation of a given rate will appear before they construct that representation.
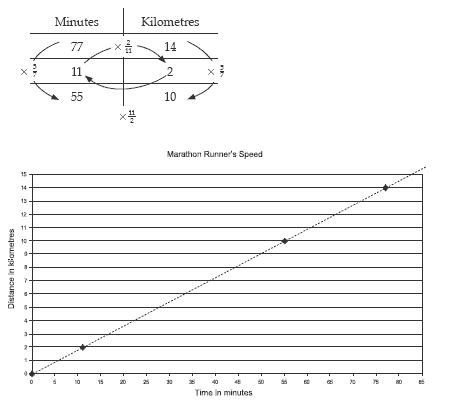
For example, ask the students, “A marathon runner tries to travel at the same speed to save energy and avoid overheating. In 77 minutes, she runs 14 kilometres. What is her time for 10 kilometres?”
Let the students chose the representation they prefer to solve the problem but require them to image what it will look like. Compare their predictions before connecting the representations. For example, the table and graph appear like this:
Ask the students, “What is the runner’s speed, in kilometres per minute?” The unit rate in this case is 14 ÷ 77 = 2 ÷ 11 = 2/11 km/m (two-elevenths of a kilometre per minute). This is the slope of the graph line (increase in distance per increase in time).
“What is the number of minutes she takes to run 1 kilometre?” This is the reciprocal of 2/11 , which is 11/2 = 5 1/2 m/km (minutes per kilometre). Multiplying this rate by ten, for ten minutes, to get 10 x 5 1/2 = 55 minutes, solves the problem. Pose other problems that involve finding missing values in rate problems. Suitable problems are:
“Tracey uses 42 apples to make 30 pies. How many apples would she need to make20 pies of the same kind?” (42:30, so 7:5, so 28:20)
“Kahu uses 36 litres of paint to spray-paint 16 similar cars. How many cars could he paint with 27 litres?” (36:16, so 9:4, so 27:12)
Emphasise the use of between strategies, such as working out the unit rate or other rates connecting the measures, and within strategies, where the given rate is scaled to match the other value given. For example, in the paint problem, the given rate of 36 litres per 16 cars can be scaled down to 27:12 through multiplying by 27/36 = 3/4 i.e., 3/4 x 16 = 12. This is an example of a within strategy.
Using Number Properties
The aim at this progression is for students to choose within and between strategies appropriately, depending on the numbers given in rate problems. They should operate on the numbers rather than relying on materials or diagrams, though folding back may be useful at times. Students should be exposed to problems involving rates created from standard measures because this has important applicability to science and technology. Through these problems, students explore derived measures such as grams per square centimetre (g/cm2) kilograms per cubic metre (kg/m3) for density, and kilometres per hour (km/h) for speed. Also encourage the students to choose appropriately between mental calculation, written recording, and using a calculator. Calculators have an important role to play where the calculations are cumbersome but must be supported by conceptual understanding of what each calculation step is doing.
Suitable problems are:
“A 10 000 cm3) cubic centimetre) piece of pine wood has a mass of 5300 grams. What would be the mass of a 4000 cm3 piece of the same wood?” (10 000:5300, so 1000:530, so 4000:2120)
Note: Mature pine has a density of 530 kg per m3. m3 is the symbol for cubic metre, which is 1 000 000 cm3 (one million cubic centimetres).
“A bus travels 56 kilometres in 35 minutes at a constant speed along the desert road. How long does the bus take to cover the first 24 kilometres at that speed?” (56:35, so 8:5, so 24:15, or 35 is 5/8 of 56, so 5/8 of 24 = 15)
“A colour television uses 450 watts of electricity in the 120 minutes it is on. How many watts would it use in 70 minutes?” (450:120, so 15:4; 15/4 = 3 3/4 watts per minute, 70 x 3 3/4 = 262.5 watts)
“Forty-eight cubic metres of water pass through the turbine of a power station in 30 seconds. How much water passes through the same turbine in 18 seconds?”(48 ÷ 30 = 1.6 m3/s, 18 x 1.6 = 28.8 m3)
“The vet decides how much of a drug to give dogs on the basis of body weight at a proportional rate. If a 28 kilogram dog gets 364 milligrams for each dose, how muchshould a 7 kilogram dog get?” (364 ÷ 28 = 13 mg/kg, 7 x 13 = 91 mg).