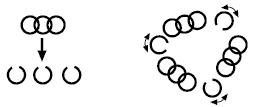These are level 4 geometry and level 5 number problems from the Figure It Out series.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (287 KB)
solve problems involving division and decimals (Problem 2)
use fractions of two dimensional shapes to solve puzzles (Problem 3)
Problem One
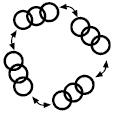
An obvious strategy is for students to try to keep each length of chain intact.
This joining will require four links to be opened and closed. That will cost 4 x $1.20 = $4.80, so it cannot be done within budget.
If the jeweller opens and closes all three links in one length of chain, the job can be done within budget.
The total cost will be 3 x $1.20 = $3.60.
As an extension, challenge students to join these lengths of chain into one bracelet for the least cost:
This can be done by opening and closing six links.
Problem Two
This problem explores the idea of common ratios or equivalent fractions. Students should know that an answer greater than one can only be obtained if the number being divided (the dividend) is greater than the divisor, for example, 8 ÷ 4 = 2, 6 ÷ 4 = 1.5, 3 ÷ 2 = 1.5.
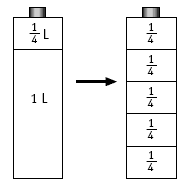
1.25 L is a common capacity of soft-drink bottles, and some students may know it as “one and a quarter litres”. A representation of this might be:
So 11/4 litres is five quarters.
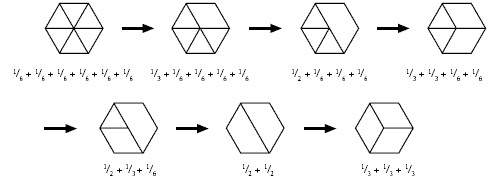
One way of getting 1.25 is 5 ÷ 4. Students may know that 5 ÷ 2 = 2.5 or 21/2, so dividing five by twice two (four) will halve the answer (1.25).
From this solution, an infinite number of other possibilities can be developed. For example:
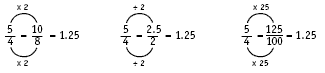
Problem Three
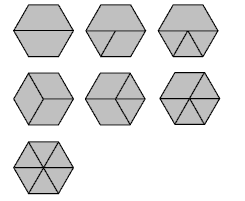
Some students may need to use pattern blocks to find the different ways of making hexagons.
A systematic way of working is:
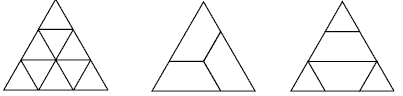
As an extension, students might explore how many ways a triangle could be filled using a number of these shapes:
For example:
Problem Four
The first four equations in the pattern are:
4 x 44 = 176
4 x 444 = 1 776
4 x 4 444 = 17 776
4 x 44 444 = 177 776
In the pattern, there is always one more four in the second factor than there are sevens in the answer. Reversing this …
leads to 4 x 444 444 = 1 777 776
Answers to Problems
1. Yes, but only if the jeweller opens and closes 3 links of one chain and uses these to connect the other chains. Cost = 3 x 50c + 3 x 70c = $3.60
2. There are an infinite number of answers.
Solutions include: 2.5 ÷ 2; 5 ÷ 4; 7.5 ÷ 6; 10 ÷ 8; 12.5 ÷ 10; 15 ÷ 12 … (Notice the pattern: the dividend increases by 2.5, and the divisor increases by 2.)
3.
4. a. 176; 1 776; 17 776
b. 177 776
444 444
There is always one more 4 in the second factor than there are sevens in the answer.