The purpose of this unit of three session is to develop understanding of how to recognise and record relationships of (equality and) inequality in mathematical situations.
- Understand the equals symbol as an expression of a relationship of equivalence, and explain this.
- Recognise situations of inequality and use the inequality (‘is not equal to’) symbol, ≠.
- Understand that < and > symbols can make equivalent statements.
- Use relationship symbols =, <, > in equations and expressions to represent situations in story problems.
- Understand how to find and express the difference between unequal amounts.
The first relationship symbol that most students encounter is the equals symbol, =, which communicates a relationship of equivalence between amounts. It is important for students to understand that symbols help us to express relationships between numbers and that equivalence is just one such relationship.
Inequality is the relationship that holds between two values when they are different. Their relative value is described with specific language including ‘is greater than’, ‘is more than’, ‘is bigger than’ or ‘is less than ‘ or ‘is fewer than’. These are expressed using the symbols, <, >, which are said to show ‘strict’ relationships of inequality. Whilst not introduced here, the symbols, ≤ , meaning ‘is less than or equal to’, and , ≥, meaning ‘is greater than or equal to’, are known as ‘not strict’. The notation ≠, meaning ‘is not equal to’ is briefly introduced here as it is a useful, if infrequently used, relationship symbol.
Algebra is the area of mathematics that uses letters and symbols to represent numbers, points and other objects, as well as the relationships between them. Through exploring both equality and inequality relationships, and the symbols used to express these, students develop an important and heightened awareness of the relational aspect of mathematics, rather than simply holding the computational view of mathematics that arises from the arithmetic emphasis that is dominant in many classrooms.
The learning opportunities in this unit can be differentiated by providing or removing support to students and by varying the task requirements. The difficulty of tasks can be varied in many ways including:
- encouraging students to work collaboratively in partnerships such as older or more skilled learners working with younger or less capable learners (tuakana/teina)
- varying the complexity of the numbers used in the problem to match the number understanding of students in your class. For example, increase the complexity by using larger numbers for students who are able to count-on to solve problems.
- using the activities suggested to form the basis of independent practice tasks.
The contexts for this unit can be adapted to suit the interests and experiences of your students. For example:
- instead of heights of buildings you might use heights of trees in a native forest, the heights of mountains such as the Southern Alps, or heights of people
- change the stories from the copymaster to include more familiar contexts
- te reo Māori that could be introduced in these sessions are orite (equal) and kaore e orite (not equal)
- Unifix cubes
- Street map diagram (a simple, made up one), A1 or A2 size, for example:

- Small blank cards
- Copymaster 1
Session 1
SLOs:
- Understand the equals symbol as an expression of a relationship of equivalence, and explain this.
- Recognise situations of inequality and use the ‘is not equal to’ symbol.
- Recognise and describe in words the relative size of amounts.
Activity 1
- Begin by talking about buildings in your school, suburb, town, city, or a city nearby. List any tall buildings which are known by name. Ask if anyone knows any (familiar) buildings that might be the same height. If using trees, begin by talking about the trees in a local bush reserve, forest or garden and have an image of a forest scape ready for step 2.
- Show a skyline picture of Auckland City and ask what features the students notice. (eg. ‘buildings are of different heights’).

Elicit descriptive, comparative language: tall, taller, tallest, short, shorter, shortest, same).
Point out that we have been comparing and describing the buildings in relation to one another and explain that we will now be investigating relationships between numbers. - Make unifix cubes available to the students and tell them to think of their favourite number between (and including) one and ten. Ask them to take this number of cubes of one colour.
For example, one student takes seven pink cubes.
Place a simple city street map in front of the students, or create one with them.
Have the students join their cubes to make buildings for this city. When they have made their ‘tower buildings’, have them locate them, standing up, in places of their choice between the streets, creating a ‘cityscape'. If adapting to use native forest, rivers and streams could replace the roads of the street map, and cubes can become 'trees'. This adaptation would be continued on throughout the remainder of the sessions and activities. - Have students look carefully at their ‘city’ and identify any buildings that they think might be the same height. Select several students to test their idea by taking the two identified ‘towers’, standing them side-by-side and comparing their heights. If they are the same they should count the number of ‘storeys high’ they are (number of cubes) and, on the class chart, write an equation and words to show this. For example:
 5 = 5 five is equal to five
5 = 5 five is equal to five
Read the equation together, “Five is equal to five” and “Five is the same as five.”
The buildings are then returned to their place ‘in the city’. - Have students now identify towers that are not the same in height.
Have a student describe how these numbers (of storeys) are ‘related’: “six is more than four”, “four is less than six”, “six is not equal to four.” Ask, “How can you write this?” Record the students’ suggestions, accepting all ideas. - On the class chart write "6 ≠ 4, six is not equal to four" and have students in pairs, read this expression of inequality to each other, read it together.
Have students identify more ‘unequal buildings’ and record these as inequality statements on the chart and read them.
If possible retain the class ‘cityscape’ for Session 2.
Activity 2
- Make plain A4 paper, felt pens, and cubes available to students. Have them work in pairs to create their own small ‘city’ with street map and cube buildings (or forest or mountain range).
- On separate paper, each student is to write about the buildings in their ‘city’. They should draw at least four pairs of buildings and for these, write both equality and inequality statements in words and symbols, as modeled in Activity 1, Step 5 (above).
Have student pairs retain their maps for Session 2.
Activity 3
Conclude the session by having the students share their recording and discussing how the symbols = and ≠ show us how numbers are related to each other.
Photograph the class and pair ‘city models’ to display with student recording from Activity 2, and from further sessions.
Session 2
SLOs:
- Recognise situations of inequality and use the appropriate symbols, ≠, <, >, to express this.
- Understand that in using < and > symbols, we can make equivalent statements.
Activity 1
- Place the class ‘city’ from Session 1 with its map and tower buildings in front of the students. Review the symbols = and ≠ and ask the students, “What is common about these symbols?” (They both express a relationship between numbers.)
Explain that there are more relationship symbols and that they will learn about two more in this session. - Ask for a volunteer to find two towers that match this number expression:
6 ≠ 4
Have student pairs discuss the towers
then, as a class, record their observations, including ‘6 is more than 4’ and ‘4 is less than 6.’ Ask if anyone knows symbols that show each of these relationships. - Write these symbols on the class chart.
< >
Write the words, ‘is more than’, and ‘is greater than’, together on the class chart, and ‘is less than’ or ‘is fewer than’ together.
Have students discuss these in pairs and decide which symbol goes with which pair of phrases and why they think that. - Accept all ideas. Conclude, agree, model and record "six is greater than/is more than 4".
Activity 2
- Make available to the students small pieces of card (the same size)and felt pens or pencils. Explain that they are now to work in pairs with their own ‘city’.
Each student is to write at least four inequality cards for pairs of ‘buildings’. For example: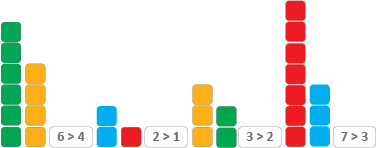
- Have the students then mix up their cards so that they don’t match the ‘buildings’. They then swap with another student pair, and correctly match their cards and ‘buildings’.
Activity 3
- As a class, discuss and conclude that the same relationships can also be expressed using the “is less than’ or ‘is fewer than’ symbol. Demonstrate with ‘buildings’ (cubes) from Activity 1, Step 4. (above):

"Four is less than/is fewer than 6" - Have students return to their own displays from Activity 2, Step 1 and write four more cards expressing ‘is less than’ relationships.
Each student should now have written at least 4 pairs of cards; 16 cards in total for the pair.
Activity 4
- Have students shuffle the cards they have made in Activity 3, Step 2 and swap these with another student pair.
Each pair is to play a short Memory game with these cards by spreading them out face down in front of them and trying to find matching pairs of statements. For example:

- Students who finish quickly can create towers to match some of the pairs of inequality statements.
Activity 5
Conclude the session by reviewing the four relationship symbols (one of equality and three of inequality) that have been used in Sessions 1 and 2.
=, ≠, <, >.
Retain student ‘cities’ and relationship cards for Session 3.
Session 3
SLOs:
- Use relationship symbols =, <, > in equations and expressions to represent situations in story problems.
- Understand how to find and express the difference between unequal amounts.
Activity 1
- Begin asking, “Who walked to school this morning?” Say that you are going to read a short story (Copymaster 1).
Explain that the students must listen very carefully to the story. As they do so, they should record relationship expressions, in order, for any numbers that they hear. Read the story once. Highlight an example (eg. 3 >2 weetbix) and read the story again. - Have students compare their expressions and equations in pairs.
- Share and discuss the expressions and equations as a class, recording them on the class chart.
Activity 2
- Write the word ‘difference’ on the class chart. Ask students to explain this, giving examples from their own life, and record their ideas. For example: "There’s a difference between the number of people in my family and Maia’s family. There’s five in my family and eight in Maia’s. They’re not the same."
- Refer to the inequality expressions recorded on the class chart in Activity 1, Step 2
For each discuss and record the difference. For example:
Weetbix: 3 > 2, 2 < 3,
Three is one more than two. Two is one less than three.
The difference is one.
Age: 60 > 50, 50 < 60
Sixty is ten more than fifty. Fifty is ten less than sixty.
The difference is ten.
Cats: 6 > 0, 0 < 6
Six is six more than zero. Zero is six less than six.
The difference is six.
Dog: 1 = 1
One is the same as one. There is no difference.
The difference is zero.
Activity 3
- Make available to the students, small pieces of card (the same size) and felt pens or pencils.
Display two ‘towers’ from the class ‘city’. Ask. “What is the difference between the two towers? How do you know?” For example:
Show:

 and write
and write 
Elicit explanations such as 'there are two more green ones, there are two less/fewer yellow ones'.
Write 6 – 4 = 2 on the class chart and on a card.
Highlight the fact that when we solve a subtraction problem we are finding the difference. - Have student pairs go to their ‘cities’ and relationship cards from Session 2.
Explain that they are to write a difference card and a subtraction equation card as shown in Activity 3, Step 1 for each of their inequality expression pairs. Have partners check each other’s cards.
For the pair, there are now 32 cards in total, 8 sets of four cards.



These can be put together in a bag, or combined with an elastic band. - Have student pairs exchange full sets of cards. Have pairs, or fours, play Fish for Four with one set of cards.
Purpose: To recognise equivalent pairs of inequality expressions, and their matching subtraction equation and difference statements.
How to play:
Cards are shuffled. Five are dealt to each player. The spare cards are put in a pile, face down, handy to all players.
Players check to see if they have any complete sets in their hand. If so, these are displayed face up in front of them. Each player then privately identifies which set they will collect and they take turns to ask one other named player for a specific card to complete their set.
For example:
In hand: and
and 
At their turn, the player says, “Name, do you have the card, four is less than six?”
If the named player has the card, they must forfeit it. The successful player can ask again till they are told, “No. Go fish.” That player then takes a card from the top of the upturned pile of spare cards. It is then the turn of the next player.
The winner is the player with the most complete sets when all cards are used.
Activity 4
Conclude this session by reviewing key learning from this series of three lessons. Sets of cards can be used as an independent consolidation task.
Dear parents and whānau,
In maths we mostly write equations. These use the equals (=) symbol. This symbol tells us that two amounts are equivalent. However sometimes numbers or amounts are not equal.
In maths this week, the students have been learning how to record inequality expressions such as 8 > 6, (eight is greater than or more than six) and 6 < 8 (six is less than or fewer than eight.) They have also learned to find the difference between numbers by solving a subtraction equation (in this case 8 – 6 = 2) and to state the difference (the difference is 2).
They have made their own card games to play with you at home.
Your child will explain how to play Fish for Four.
We hope you enjoy playing Fish for Four, and enjoy helping your child to practice their learning about inequality expressions and number relationships.