This is a level 5 number activity from the Figure It Out series. It relates to Stage 8 of the Number Framework.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (1024 KB)
find fractions of numbers using multiplication and division
Number Framework Links
Use this activity to help students consolidate and apply their knowledge of fractions and proportions (stage 7).
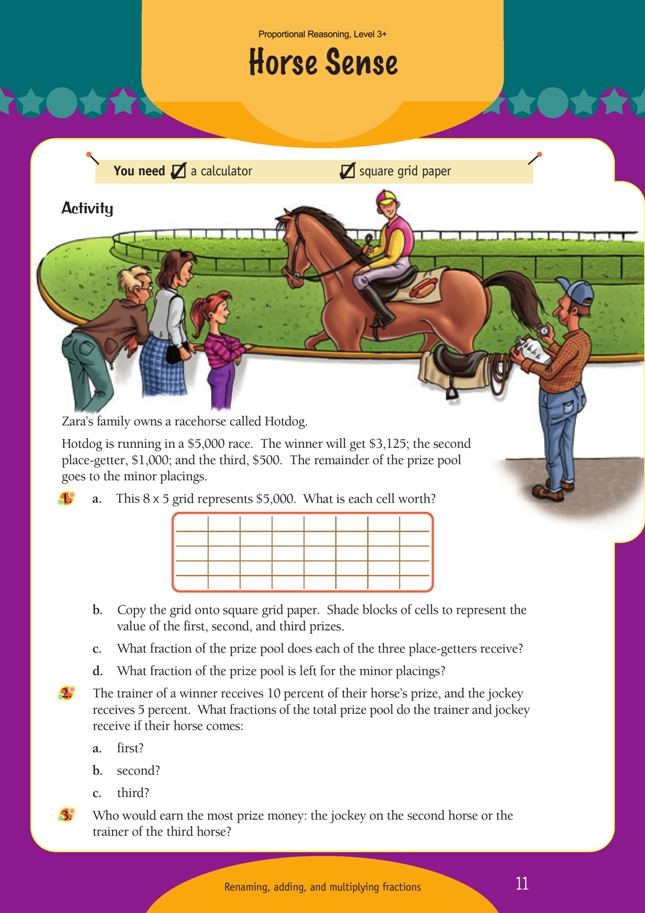
FIO, Level 3+, Proportional Reasoning, Horse Sense, page 11
Square grid paper
This activity is based on a real-life context: prize pools. Although the book suggests that a calculator can be used, students are likely to learn more about proportional reasoning if they manage without one. Answers expressed as fractions will picture the ratios better than decimals.
Question 1a provides a visual model of the prize pool and asks students to work out the value of each cell ($125). They might do this using a partitioning strategy: 40 x 100 = 4 000, leaving 1 000; 40 x 20 = 800, leaving 200; 40 x 5 = 200. So one cell is worth 100 + 20 + 5 = $125. Alternatively, they might note that the grid has 5 rows worth $5,000, so 1 row of 8 cells must be worth $1,000. 8 x 100 + 8 x 20 + 8 x 5 = $1,000, so again 1 cell is worth 100 + 20 + 5 = $125.
In question 1b, students shade blocks of cells to represent the value of the first three prizes. To do this, they first need to work out how many lots of $125 equate to the value of each prize. Perhaps the simplest strategy is to use repeated doubling to establish that 2 cells are worth $250, 4 are worth $500, and 8 are worth $1,000. Using this information, students should be able to see that the winner ($3,125) is represented by 3 x 8 + 1 = 25 cells, and the second and third placegetters
($1,000 and $500) by 8 and 4 cells respectively.
In question 1c, students work out what fraction of the prize pool goes to each of the three placegetters. This is the same as working out what part of the grid has been shaded for each of the three placings. All three fractions can be expressed with 40 as the denominator, but you should be encouraging your students to always simplify fractions if possible.
The 3 unshaded cells should tell the students that the answer for question 1d is 3/40. At this point, you could ask them why the grid was divided into 40 rather than 20 or 50 or some other number. The reason is, of course, that 40 is the lowest common denominator of the four fractions, 5/8, 1/5, 1/10, and 3/40.
For question 2, students need to calculate the trainer’s fraction; they can then halve it for the jockey. They first must know that 10% is the same as 1/10. The Answers show how the answers can be worked out by multiplying two fractions then simplifying.
Once students have solved question 2, they can use their solutions to provide the answer to question 3. No further calculation is needed.
Answers to Activity
1. a. $125. (5 000 ÷ 40 = 125)
b. Here is one way the grid can be shaded:
c. First: 25/40 = 5/8; second: 8/40 = 1/5; third: 4/40 = 1/10
d. 3/40
2. a. 5/8 x 1/10 x 5/80 = 1/16 for the trainer and 1/32 for the jockey.
b. 1/5 x 1/10 = 1/50 for the trainer and for the jockey.
c. 1/10 x 1/10 = 1/100 for the trainer and 1/200 for the jockey.
3. Both earn the same (1/100 of the total prize pool).