This is a level 3 number and measurement activity from the Figure It Out series.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (1027 KB)
use multiplication to solve perimeter and area problems.
Number Framework links
Use this activity to encourage transition from advanced additive strategies (stage 6) to advanced multiplicative strategies (stage 7).
Activity
This activity is based on the perimeter and area of rectangles. As a general introduction, have your students look at this rectangle where the side lengths are given as l and w.
The area can be found by: area = l x w.
The perimeter can be found by: perimeter = 2 x l + 2 x w.
Problems that involve maximising or minimising one measurement while either holding the other constant or minimising it are common in the real world. Fred’s fence is typical of constrained maximisation or minimisation problems.
Students exploring question 1 are likely to try different side lengths that will result in an area of 80 square metres. The problem requires a systematic approach, so encourage your students to organise their results in a table or organised list:
| Side A | Side B | Area | Perimeter |
| 1 | 80 | 80 | 162 |
| 2 | 40 | 80 | 84 |
| 4 | 20 | 80 | 48 |
| 5 | 16 | 80 | 42 |
| 8 | 10 | 80 | 36 |
In this way, the students can find all the solutions with whole-number side measurements and calculate the perimeters at the same time. They may notice that the closer the side measurements become to each other, the smaller the perimeter becomes.
Encourage your students to explore the minimum perimeters for rectangles with the areas 16, 36, and 64 (square numbers). They will find that the perimeter is minimised when the rectangle is a square. In this situation, the length of each side is the square root of the area. They can then go back to question 1 with the knowledge that the solution is the closest whole number to √80 = 8.944 (to 4 significant figures). Students are likely to argue that the question asked for a rectangle and that a rectangle is not a square. It is worth stopping to discuss this reasonable view. In everyday use, a rectangle and a square are different shapes, but in mathematics, a square is just a special case of a rectangle.
Provide the students with a set of rectangles and squares and ask them to describe the attributes of these shapes. Encourage them to come up with minimal definitions, listing just the attributes that are absolutely necessary to define the shape. Students will typically say that a rectangle has:
• 4 sides
• 4 right-angled corners
• 2 pairs of parallel sides.
If you ask them to draw a 4-sided polygon that has right-angled corners but does not have 2 pairs of parallel sides, they will find that this is impossible. So it is not necessary to state that opposite sides must be parallel. This gives us the minimal definition for a rectangle. The minimal definition of a square is “a 4-sided polygon with right-angled corners and equal sides”. Squares are therefore a subclass of rectangles.
In the Investigation, students try to find rectangles that have the same number for the measurement of their perimeter as they do for the measurement of their area.
One solution is a square with sides of 4 metres. Its perimeter is 16 metres, and its area is 16 square metres. If they are systematic, students should be able to establish the existence of two other whole-number solutions.
They could begin by setting the length (at, say, 2 metres) and exploring what widths might work. They will discover that no whole-number solution will work for a side length of 2. But if they then try 3, they will find that a 3 x 6 rectangle has an area of 18 square metres and a perimeter of 18 metres. 6 x 3 is a third solution, but this is not a genuinely different rectangle.
Having got this far, your students may guess that there are other rectangles that meet the requirement but that they do not have whole-number sides. There are in fact an infinite number of such rectangles. In the table below, there are six rectangles that happen to have a whole-number measurement for one of their two dimensions. You could give your students the length of side b and challenge them to find the length of side a (in bold in the table), using a trial-and-improvement strategy.
| Side a | Side b | Area | Perimeter |
| 7 | 2.8 | 19.6 | 19.6 |
| 10 | 2.5 | 25 | 25 |
| 12 | 2.4 | 28.8 | 28.8 |
| 18 | 2.25 | 40.5 | 40.5 |
| 22 | 2.2 | 48.4 | 48.4 |
| 27 | 2.16 | 58.32 | 58.32 |
There is an algebraic relationship between the pairs of values of a and b that satisfy the requirement that the number of perimeter units must be equal to the number of units of area. The relationship can be expressed in this way: ![]()
(To find the length of the second side, double the length of the first and divide by its length less 2.) Students who are developing an understanding of symbolic notation may like to try using this formula to find other pairs for a and b with the help of a calculator or spreadsheet program such as that shown.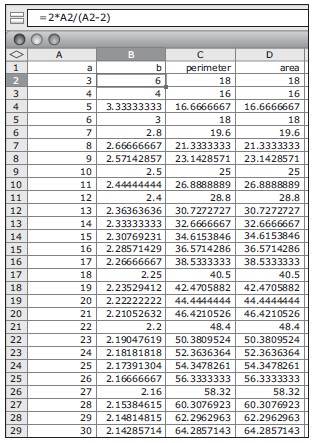
Links
Numeracy Project materials (see https://nzmaths.co.nz/numeracy-projects)
• Book 9: Teaching Number through Measurement, Geometry, Algebra and Statistics Investigating Area, page 11
Figure It Out
• Number: Book Three, Years 7–8, Level 4 Orchard Antics, page 23
• Number Sense and Algebraic Thinking: Book One, Levels 3–4 Tile the Town, Tiny!, pages 20–21
Answers
1. a. 5 different rectangular shapes are: 1 m by 80 m, 2 m by 40 m, 4 m by 20 m, 5 m by 16 m, and 8 m by 10 m. Only the last two shapes would suit the dodgems (the other three would be too narrow).
b. The 8 m by 10 m rectangle would use 36 panels and cost $108, which is cheaper than the other options. It is one of the shapes that would suit the dodgems.
2. a. There are 12 different-sized rectangles that could be made.
| Width | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 |
| Length | 23 | 22 | 21 | 20 | 19 | 18 | 17 | 16 | 15 | 14 | 13 | 12 |
b. The largest option is 12 m wide and 12 m long, which gives an area of 144 m2. The shape of this area is a square.
3. 50 m. The length must be 15 m because 10 x 15 = 150. 2 x (10 + 15) = 2 x 25 = 50 gives the perimeter.
Investigation
Answers may vary. There are three whole-number solutions: 4 x 4, 3 x 6, and 6 x 3 (which is the same as 3 x 6). There is an infinite number of solutions if rectangles with only one whole-number side or no whole-number sides are included.
