Entertaining Patterns

Activity One

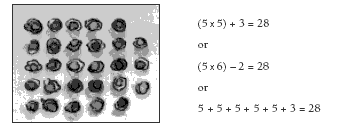
This activity gives the students a chance to apply their number-sense skills and knowledge of operations. They should be able to do quick mental operations by translating readily seen patterns into numbers of subtotals. You will need to give the students an opportunity to share and to discover which methods are most efficient. For example, the students may see this group of people as:

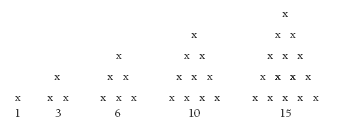
The students will find that they can easily count some groups if they use the multiplication array and their knowledge of triangular numbers. For example, the first five triangular numbers are:

The students will see that this group of people is the third triangular number, 6.
Any number up to 4 can be seen instantly and could be added mentally as a separate group. It may be helpful to break the diagram into regions, as shown below.

Activity Two

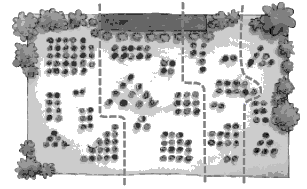
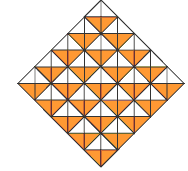
The students can use several strategies to identify and continue the pattern on the adults’ costumes. They could use the mosaic pattern blocks issued as part of the Beginning School Mathematics resource (Ministry of Education, 1985–1993) to make up the pattern as shown and then extend it to the fifth row. They could then count the number of triangles of each colour.
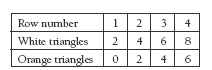
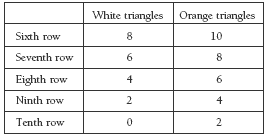
Another strategy is to record the number of triangles in each row in a table and then analyse it:

The students should quickly see that the number of triangles of each colour increases by two in each successive row. The students could generate this sequence from most calculators, using the constant function:
+ 2 = = = = = .
Finally, the students could draw the fifth row of the pattern, as they are required to do for question 1b.
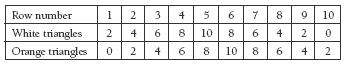
Before the students begin question 2, make sure that they understand that after the fifth row of the adults’ costumes, the number of triangles decreases in each successive row. (The copymaster shows how the overall shape comes back in again.) You could draw the sixth row with the students. You might also like to ask them to work in small groups to make up the complete, 10-row pattern. The number of triangles in each row of the adults’ costumes is:

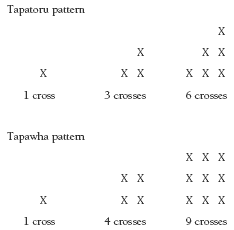
As an extension, you could encourage the students to look at and analyse pictures or examples of other kapa haka costumes or tukutuku patterns. Two other patterns that could be extended and analysed include:
Tapatoru pattern

The first pattern, and others, are explored on page 3 of Algebra, Figure It Out, Level 3.

Answers to Activities

Activity One
a. Discussion points should include using multiplication to count the square and
rectangular groups, using triangular numbers to count the triangular groups, and using multiplication and addition or subtraction to count the remaining groups.
Use subtotals to keep track of progress.
b. 165
Activity Two
1. a. 10 white, 8 orange
b.

c. The number of triangles of each colour increases by 2 at each successive row.
2. a.

b.