This problem solving activity has a geometry focus.
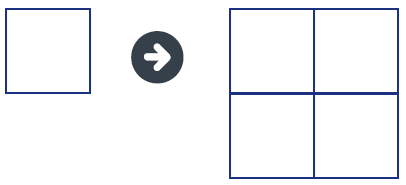
Simon has just discovered copycats. A square is a copycat because you can put four of them together to make another square.
Simon wonders if triangles and circles are copycats. What do you think?
- Tessellate using squares and triangles.
- Describe the properties of tessellating shapes.
- Devise and use problem solving strategies to explore situations mathematically (guess and check, make a drawing, use equipment).
This problem explores tessellation: covering a plane surface by the repeated use of a single shape, without gaps or overlapping. Students are challenged in the first instance to identify shapes that tessellate (squares and triangles) and those that do not (circles). To do this, students should have some knowledge of two-dimensional shapes, and their basic properties (e.g. edges, corners, equal interior angles).
The 'copycat' idea in this problem involves students using tessellating squares to make a larger square, which is a 'copycat' shape of the smaller shape (i.e. the square), and using tessellating triangles to make a larger triangle in the same manner. This prompts the investigation of square numbers, such as 1, 4, 9, 16, 25, and so on.
The extension challenges students to find other shapes that tessellate to form a larger 'copycat' shape.
- Copymaster of the problem (English)
- Copymaster of the Problem (Māori)
- Squares, circles, triangles (hard copy and/or a digital model)
The Problem
Simon has just discovered copycats. A square is a copycat because you can put four of them together to make another square.
Simon wonders if triangles and circles are copycats. What do you think?
Teaching Sequence
- Ask: What is a copycat?
- Discuss the definition of copycat as it applies to this problem.
- Pair up the students and give them an equilateral triangle. Ask them to find out whether it is a copycat. Discuss the properties of an equilateral triangle (3 equal sides, 3 corners of the same size/equal angles)
How do you know that they are equal? (fold and check) - Share findings.
- Pose the problem: Are all triangles copycats?
The students need to make their own triangle and see if it is a copycat. As they work ask:
What type of triangle are you using? (right triangle, scalene, isosceles, equilateral)
Describe the triangle that you are using?
Are circles copycats? Why do you think that? - Share findings.
Extension
- Simon talks to Ben about his copycats. Ben notices that you need four squares to make a bigger square and four triangles to make a bigger triangle. Can you make a square with any other number of squares? How many squares can you put together to make a square? Is there a pattern here?
- What other shapes are copycats?
Solution
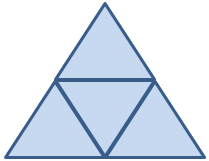
All triangles are copycats. This can be shown using four triangles as in the picture.
On the other hand, circles aren’t copycats. Clearly two or more circles don’t tessellate to make a circle because there are always gaps between the shapes.
Solution to Extension 1:
Any square number of squares 4, 9, 16, 25 can be used. Why can’t a non-square number be used? Is this the same for triangles too or do you have to use triangle numbers there?
Solution to Extension 2:
The L-shape in the drawing below is a copycat. To check this out, put one L-shape around each of the top-left, bottom-left and bottom-right corners.