Solve multiplication and division problems that involve fractions.
Number Framework Stage 8
Using Materials
Present the students with this problem:
“Toline has just filled up the petrol tank of her car. She drives to and from work each day. Each return trip takes three-tenths of a tank. How many trips can Toline make before she needs to fi ll up?”
Encourage the students to draw representations of the problem such as strip diagrams. As the students provide solutions, link their ideas to a visual or equipment model. For example, fraction strips might look like this:
![]()
Note that a conceptual obstacle is that the students must express the answer in terms of a new measurement unit, three-tenths. So the problem has two important units, the original one and the unit of measure, three-tenths. The number of trips is the answer to the question, “How many three-tenths measure one?” (Three whole trips and one-third of a trip)
This can be recorded symbolically as 1 ÷ 3/10 = 3 1/3 trips. Ask the students what 3 1/3 is as an improper fraction (10/3). Write the equation as 1 ÷ 3/10 = 10/3.
Students may notice that 3/10 and 10/3 are reciprocals. Ask, “Why is the answer 10/3?”
(There are ten-tenths in one, and three are used each time to make a unit of three tenths). Note the important connection to a ÷ b = a/b, e.g., 10 ÷ 3 = 10/3.
Provide the students with two other examples in which one whole is divided by a non-unit fraction. For example:
1. [Name]’s car has a full tank of petrol. Each trip takes five-eighths of a tank.
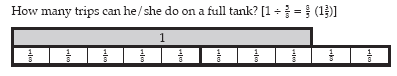
2. [Name]’s car has a full tank of petrol. Each trip takes two-ninths of a tank. How many trips can he/she do on a full tank?
Extend the concept of division by a fraction by varying the amount of petrol available. For example, “It takes two-fifths of a tank to make one trip. How many trips can [Name] make on three full tanks of petrol?” (3 ÷ 2/5 = 15/2 = 7 1/2).
Connect the answer to each problem to the answer for one full tank divided by the fraction consumed for one trip, e.g., 1 ÷ 2/5 = 5/3 (2 1/2) so 3 ÷ 2/5 = 15/2 (7 1/2). Students should notice the scaling effect of how much petrol is available.
Another problem might be:
“It takes one-sixth of a tank to make one trip. How many trips can [Name] make on three-quarters of a tank of petrol?”(3/4 ÷ 1/6 = 18/4 because it is three-quarters of the answer to 1 ÷ 1/6 = 6/1). Note the connection to fractions as operators, e.g. 3/4 x 6/1 = 18/4, and to any whole number as a fraction with a denominator of one, e.g., 8/1 = 8.
Using Imaging
Pose similar problems in context using students’ names from the teaching group. The emphasis should be on students anticipating the answer to a problem and, where necessary, checking their prediction using a diagram or equipment.
Recording the problems as division equations is vital in helping the students to generalise the process of solving such problems.
Be aware of other useful strategies that students may develop. Using equivalence is another way to solve division problems with fractions.
For example, [Name] has nine-tenths of a tank of petrol. Each trip takes one-quarter of a tank. How many trips can [Name] make? (9/10 ÷ 1/4 = ?) .
Both nine-tenths and one-quarter can be converted to fractions with the same denominator (e.g., twentieths), so the same units are involved.

Examples of other problems might be:
3/4 ÷ 3/5 = 15/12 = 5/4
11/12 ÷ 1/6 = 66/12 = 11/2
7/8 ÷ 3/5 = 35/24
4/5 ÷ 1/2 = 8/5
2/3 ÷ 3/4 = 8/9
5/12 ÷ 2/3 = 15/24 = 5/8
Note that the two problems on the second line may cause confusion because the divisor is larger than the dividend. This will mean that the car can make only a fractional part of a trip, not a full trip, e.g., 2/3 ÷ 3/4 = 8/ 9.
Using Number Properties
Encourage students who are able to anticipate the answers to fraction division problems by considering the numerators and denominators to generalise the operation algebraically. This can be facilitated by asking them to describe their common procedure given three similar problems from Using Imaging.
For example, “To solve 15/2 ÷ 2/3 = ?, I made the numerator of the answer 5 x 3 and the denominator 12 x 2.”
This can lead to expression of the common method using letters as variables to represent any integers that are chosen, i.e., a/b ÷ c/d = ad/bc. .
Give the students connected problems in which they must apply the properties of multiplication and division, particularly the associative property and multiplicationand division as inverse operations. Examples might be:
• 3/4 ÷ 2/3 = 9/8 so 3/4 ÷ 4/3 = ? (? = 9/16 because 4/3 is twice 2/3)
• 3/5 x 1/2 = 3/10 so 3/10 ÷ 1/2 = ? (? = 3/5 by inverse operation)
• 5/9 ÷ 3/4 = 20/27 so 5/3 ÷ 3/4 = ? (? = 60/27 because 5/3 is three times 5/9)