This is a level 4 number activity from the Figure It Out series. It relates to Stage 7 of the Number Framework.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (350 KB)
use a variety of strategies to solve problems
Number Framework Links
Use this activity to:
• help the students who are beginning to use advanced additive strategies (stage 6) to
become confident at this stage
• encourage advanced additive strategies (stage 6) for addition and multiplication, progressing to advanced multiplicative strategies (stage 7)
• help your students to consolidate and apply their knowledge of basic multiplication facts.
FIO, Levels 2-3, Number Sense and Algebraic Thinking, Book One, Among the Dolphins, page 5
These problems use addition and multiplication, and provide a practical context to broaden and extend strategies.
Knowledge of basic facts is a key part of this activity. Some students may need to have access to a tables chart. The students also need to know the place value of 3-digit whole numbers.
These types of problems are ideal for small groups. The students share their understanding of what the problem is about and discuss ways to solve it. Then they report back to the other groups, sometimes during the process if they are having difficulties, and always after they have a solution.
Discuss the concept of family concessions. Many students will have experienced these at mini-golf courses, theme parks, or sports events. Have the students attempt to solve question 1 in their groups. Bring the groups together to discuss their strategies and to ensure that they understand how the group or family concession can reduce costs. Take this opportunity to check that the students understand that 50 cents is also “point 5 or a half of a dollar”.
Question 2 increases the challenge. If the students follow the hint for question 2a, they will find they have to account for $94.50 in family tickets. Focus on number sense by asking them:
Does this amount give you a clue as to how many family tickets were bought?
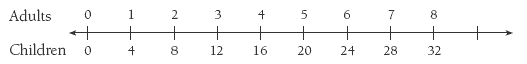
Question 3 gives a context for discussing ratio. You could explain the notation of 1:4 as a mathematical way to write this ratio. Use a double number line to show how it works as the numbers increase:
Compare the ratio of adults to children with that of children to adults so that the students see and understand the inverse relationship of 1:4 and 4:1 and say these as “1 to 4” and “4 to 1”.
Extend question 3 by asking the students what would happen if there was a group concession, for example, 4 adults and 16 children for $100. Use questions such as:
How many group concessions would the school need to buy? (6)
How much would the school pay altogether?
Have the students discuss this and record their thinking.
Students using this activity for independent practice or maintenance should be at least at the advanced multiplicative strategy stage.
Extension
The students could investigate what concessions are available at local attractions or on public transport. They could use this information to show how much money different groups would save by using the concession. They could also investigate why concessions are offered. This might involve a survey to establish “critical price points” over which people won’t buy tickets.
Answers to Activity
1. a. i. $38.50. (31.50 + 7)
ii. $38. ($10 x 4 = $40, 50c x 4 = $2.00,then $40 – $2 = $38; or $9 x 4 = $36,
50c x 4 = $2, $36 + $2 = $38)
iii. $55. (1 family pass, 1 adult’s ticket, and 2 children’s tickets:
31.50 + 9.50 + 7 + 7 = 55.
Strategies for adding these include:
50c + 50c = $1; then add $9 to $1 to make $10; then add $31 to $10 to make
$41; 2 x $7 = $14, so add that to $41 to make $55. Two family tickets cost
$63, so that is not the best option.)
b. $1.50. (2 x 9.50 = $19; 2 x 7 = $14; taking $1 from the $14 and adding it to $19 makes $20; together with the remaining $13, this makes $33, which is $1.50 more than the family ticket.)
2. a. 3. (One way to work out 9 adults is: [9 x $10] – [9 x 50c] = 90 – 4.50
= $85.50 Then: 3 children = $21. $85.50 + $21 = $106.50. $201 – $106.50 = $94.50, which is 3 x $31.50.)
b. 24. (9 adults and 3 children, plus 3 x 2 adults and 3 x 2 children)
3. 24. (96 ÷ 4 = 24; or 100 ÷ 4 = 25. 25 – 1 = 24)