This is a level 3 measurement strand activity from the Figure It Out series.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (240 KB)
find areas of irregular shapes
FIO, Level 3, Measurement, An Absorbing Challenge, page 7
classmate
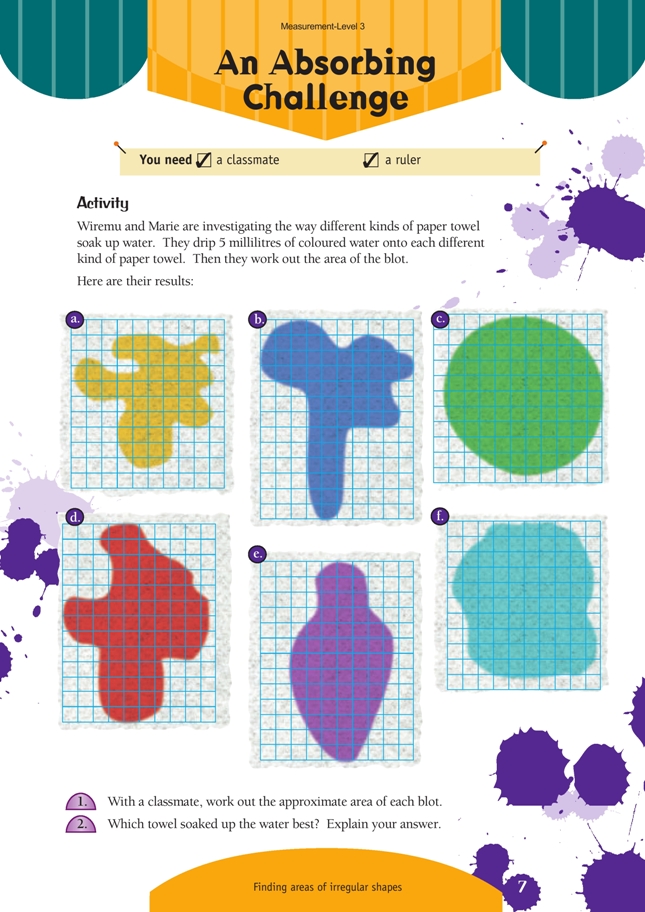
This is a good activity for encouraging students to estimate and make sensible approximations f area.
When students have made an estimate, they are usually motivated to check the accuracy of their efort.
You may wish to have students work in problem-solving groups of four people to come up with a grup list of the blots in order from largest blot to smallest blot, using estimation only. They could then split into pairs to answer question 1. After they have been working for 10 minutes, bring the groups together to discuss their estimation strategies. Their ideas may include: “We coloured all the whole squares red and then the half squares green. We used different colours for the one-quarters, three-quarters, one-thirds, and two-thirds. We then added them up to make whole squares and found the total.”
“After tallying the whole squares and crossing them off, we tried to pair up two squares that would join to make a whole. Then we tallied these and crossed them off as we went.”
“We copied the blot and then tried to cut and paste bits to make the blot into a rectangle. Then we could multiply the length by the width.”
After this discussion, the groups who have not been able to work out approximate areas for the blots could be sent back to continue solving the problem.
To clarify question 2, ask the groups: “How do we decide which towel soaked up the water best?” Students may decide that the best soaker would use the least area because it could hold more. Alternatively, they might say that the most regular shape must mean a better quality towel because it soaks up water more evenly. Or they could argue that the largest area blot may indicate the best towel because it might have been the fastest to soak up the water. Whatever their decision, the students’
choice of best soaker should match their reasoning for the way to determine the best soaker.
Answers to Activity
1. Answers will vary slightly because the part squares must be estimated as half, quarter, etc.
Approximate areas are:
a. 40 square units
b. 57 square units
c. 80 square units
d. 67 square units
e. 57 square units
f. 75 square units
2. Answers will vary, depending on what qualities students think are important, for example, absorbing all liquid in a small area.