This is a level 2 number activity from the Figure It Out series. It relates to Stage 5 of the Number Framework.
A PDF of the student activity is included.
Click on the image to enlarge it. Click again to close. Download PDF (276 KB)
add compatible numbers
Number Framework Links
Use this activity to:
• encourage transition from advanced counting strategies (stage 4) to early additive strategies (stage 5) and progression towards advanced additive strategies (stage 6)
• help your students to consolidate and apply their knowledge of groups of 5 and 10 up to 100 (place value, stage 4).
A classmate
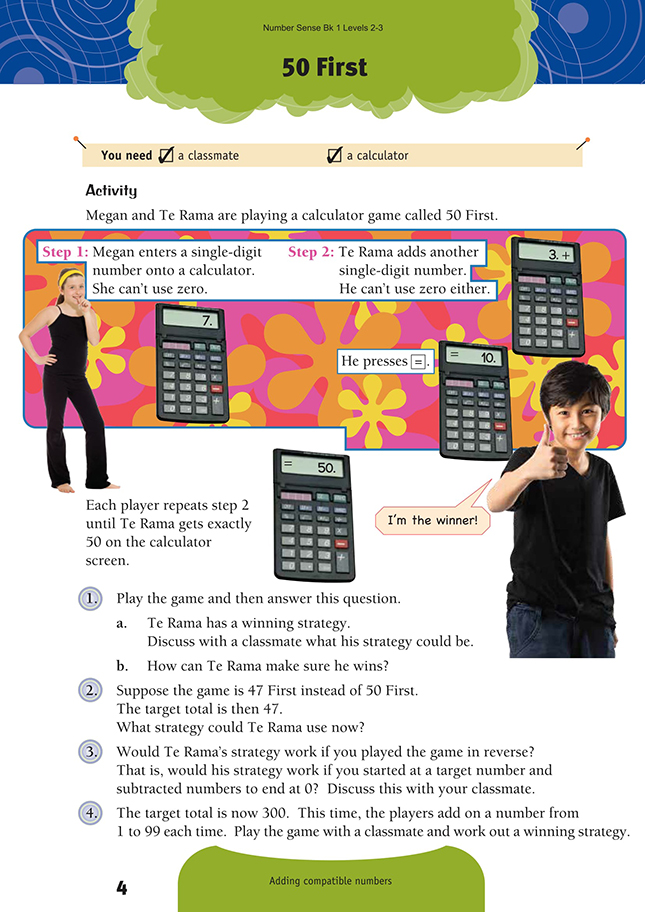
FIO, Levels 2-3, Number Sense and Algebraic Thinking, Book One, 50 First, page 4
A calculator
This activity is a variation on the traditional games of nim and murder 21. It has been structured so that students will use compatible numbers up to 10 (and later, up to 100) as a winning strategy, which will reinforce these number facts. Until the students become aware of this strategy, you will be able to focus on improving their mental calculation strategies using advanced counting or early additive part–whole thinking. It is also a great way for the students to have some fun with number.
To do this activity, the students need to be able to sequence numbers to at least 50 for questions 1 to 3 and to 300 for question 4.
To make the most of this game in a guided teaching group, make sure the students play it a number of times before they answer question 1. During this initial phase, don’t allow the students to enter their number into the calculator until they have explained the counting-on or part–whole strategies they used as they mentally added on their number. Use questions such as:
What number are you thinking of entering?
What total will that give you?
How did you get that total?
Question 1 brings out the number patterns that make the winning strategy. A good focusing question here is: What “special number” do you need to reach to be sure that your next number will add up to the final winning number?
When the students discover that they can control the outcome of the game if they’re the player who adds a number that results in 40, ask them: Is there a special number that you need to reach that lets you always make 40? Work backwards repeating this procedure until all the special numbers are identified (10, 20, 30, and 40).
Question 2 challenges the students to find a winning strategy for a target number that is not a multiple of 10. The special numbers for 47 will be 37, 30, 20, and 10.
Question 3 provides a chance for students to use counting back or part–whole subtraction strategies. The winning strategy to hit 0 means that the first special number is 10. The number that controls 10 is 20, and so on back to 40.
Make sure the students look for the connection between the largest number they can add or subtract and the range in the special numbers. They should find that it is 1 more than the largest number. As 9 is the largest number, the first special number is 10 less than the target.
To generalise the full pattern, they will need to vary the range of numbers they can add or subtract. Stipulate that they can only add from 1 to 5 to make 50. Challenge them to find all the special numbers and test them out by playing some games with a classmate. They should find that the special numbers are found by subtracting 1 more than 5 from 50 and so on. They will be 44, 38, 32, 26, 20, 14, 8, and 2.
Subtracting this new range of numbers from 50 will provide many more opportunities to have the students explain and share their subtraction strategies. These may be counting-back strategies or early part–whole ones.
Question 4 extends the numbers used into the hundreds and changes the range of numbers that they can use to add. A target number of 300 with a range of numbers from 1 to 99 will mean that 200 and 100 are the special numbers for ensuring victory.
The students should note that in all these examples, the person who goes second can always win as long as they follow the strategy. Conversely, the first person can never win unless the other person makes a mistake.
Answers to Activity
1. a. Practical activity. Discussion will vary. Te Rama tries to be the first one to get to 40. Megan’s next number will take her to a total between 41 and 49, and then Te Rama can make the answer up to 50 in 1 turn. For example, 43 + 7 = 50.
b. Te Rama can win by making sure that, whatever Megan enters on any of her turns,
he enters a digit that will take the total to the next 10. (But if he takes the first turn,
Megan will win if she follows the same strategy.)
2. Te Rama could use the same make-10 strategy up to 30. On his next turn, he would need to make the total 37 so that Megan can’t make 47. (If Megan’s total is already more than 37, Te Rama would go straight to 47.)
3. Yes, Te Rama’s strategy would work. You would simply aim to get to a decade (10) as quickly as you can.
4. You can win if you are the first to get to 200. To be certain of a win, you need to be the one to make 100 and then 200.