The key idea of patterns and relationships at level 1 is that some patterns are repeating and some are sequential.
- counting is a sequential pattern
- repeating pattern has a consistent element of repetition
- sequential pattern has a consistent element of growth.
At level 1, students learn that a repeating pattern has a consistent element of repetition. They are able to identify the repeating element, and extend the pattern. They are also able to create their own repeating patterns using symbols, numbers, shapes, sounds and movements. An example of a repeating pattern is:
The base element of repetition consists of the four counters red, blue, blue, yellow in that order.
Students will also be exploring sequential or ‘growth’ patterns where there is a consistent element of growth from one term in the pattern to the next. The most common sequential pattern is counting in ones where students learn that the next number in the sequence is found by adding one to the previous number. Similarly, taking one away will give the previous number in the counting sequence. The patterns derived from skip counting in 2’s, 5’s and 10’s are explored at this level.
Sequential patterns can also be made with geometric shapes and symbols, for example:
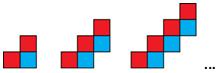
At this level, students will learn to identify the way a sequential pattern is growing, they will use this growth element to extend the pattern, and the will also be creating their own sequential patterns.
It is also important for students at this level to see and identify patterns in the built and natural environment, such as the way leaves are arranged on a branch, or different tukutuku patterns grow and repeat.
This key idea develops from informal exploration of patterns and relationships during pre-school years.
This key idea is extended in the key idea of patterns and relationships at level 2 where rules are used to describe sequential patterns.