This problem solving activity has a geometry focus.
In the game of Noughts, each player takes a turn to place a nought on the board (see below).
Each new nought goes into a new square.
The winner is the first person to place three noughts in a row.
Is it possible for either the first player or the second player, to always win? (Assume that each player plays to win and plays as well as is possible.)
If so, what is the winning strategy? If not, why not?
- Use symmetry as a game strategy.
- Apply problem solving strategies to a game context.
- Devise and use problem solving strategies to explore situations mathematically (guess and check, make a drawing, use equipment).
- Make conjectures in a mathematical context.
- Critically follow a chain of reasoning.
This problem develops the idea that games have strategies and that despite playing well, a player may still be unable to win.
Students will need to use logic and symmetry.
The Problem
In the game of Noughts, each player takes a turn to place a nought on the board (see below). Each new nought goes into a new square. The winner is the first person to place three noughts in a row. Is it possible for either the first player or the second player, to always win? (Assume that each player plays to win and plays as well as is possible.) If so, what is the winning strategy? If not, why not?

Teaching sequence
- Play a class game of Noughts. Let the class make the first move and you follow.
- Play another game of Noughts with you starting.
- Discuss their initial ideas about the game.
What ideas do you have about Noughts after 2 games?
Can you see any strategies that could be used to win?
Do you think the outcome would have been different if I had made the first move? - Pose the problem for the students to work on in pairs.
- As the students play the game ask questions that focus them on describing their reasoning.
What is a good (first) move? Why? How do you check that out? - Once the students have found the strategy check that they can identify the symmetries of the game strategy.
- Give the students the opportunity to test out their strategy with another pair.
- Share solutions – this may be in the following lesson giving the students the opportunity to test their strategies with other pairs and family members.
Extension
Try playing the game with a board with 11 squares. Will Player A still win? What if there were any odd number of squares on the board, 59, say. Who will win then? What happens if the board has an even number of squares?
Solution
The first player can always win if they plays correctly. Call the first player, Player A and the second player, Player B. To win, Player A needs to put their first nought in the centre square of the board. Then, wherever Player B goes, Player A should copy that move but on the opposite side of the board. We show this in the picture below.
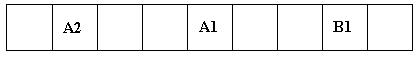
Player A has put the first nought in the centre (we have shown it as A1 to indicate that it was Player A’s first move). Then Player B has put a nought in the square marked B1. Player A has replied by putting her next nought in square A2, to match Player B’s move.
Now suppose that Player B has a move that will not mean that Player A can get three noughts in a row. There must be a square on the symmetrically opposite side of the board that is safe for Player A. If Player B doesn’t have a safe move, then Player A wins and doesn’t play symmetrically.
Solution to the Extension
Exactly the same argument holds for all odd boards as held for the 9 square board. The situation for even boards is more complicated. Sometimes Player A wins but sometimes Player B wins. You could explore which happens and when.
