Cups and Cubes

Session 1

In this session students learn to use a cup containing cubes to represent a variable.

1. Put two plastic cups of different colours on a table. Tell the students that you are going to put 12 cubes into the cups. Ask, “How many cubes might go in each cup?”

2. Draw up a table of values to organise the students’ responses. Note that students frequently neglect the options that have zero cubes in a cup. This task is an excellent opportunity to develop the concept of zero as the numbers representing ‘nothing of something.’ 

   Blue	Green
   0	12
   1	11
   2	10
   3	?

3. Tell the students to draw a graph to show the relation between the number of cubes in the blue cup and how many are in the green cup. Ask them to predict what the graph might look like. Students might hand draw the graph or use a spreadsheet like Microsoft Excel or Google Sheets to complete it. If necessary, you could model the construction of a graph, and then have students create their graphs independently or with their peers. This could also be done as a whole-class teacher-modelled activity.
   Ask students to explain why they think the points on the graph lie along a downward sloping straight line. They might notice that the value of the green cup variable goes down by one as the value of the blue cup variable goes up by one.
   

4. Using a range of different coloured cups and different numbers of cubes pose similar problems asking the students to come up with a table of values and a graph to represent the relations. The table below shows four examples of possible problems. The students should model the situation with actual cups and cubes to come with ordered pairs such as (1,3) to represent possible values that the variable may assume. Note that you are dealing with discrete variables (i.e. random variables that can take only distinct values, usually whole numbers). Therefore, the numbers of cubes should be whole numbers. Some students may consider fractions of cubes. Examples of useful relations might be:

   The red cup has two more cubes than the blue cup. This linear relation is effectively r = b + 2	Each blue cup has the same number of cubes. The number of cubes in the yellow cup equals the total number in both blue cups. This linear relation is effectively  y = 2b (b multiplied by 2)
   There are 18 cubes in total. Each yellow cup holds the same number. This linear relation is effectively 18 = r + 2y	The number of cubes in the yellow cup equals the total number of cubes in all the blue cups. This linear relation is effectively y = 3b (b multiplied by 3)

5. Note that in some of these relations, an infinite number of whole number values could be put in the table. For example, in the problem with one yellow cup an three blue cups, the table could read:

   Blue cup	Yellow Cup
   0	0
   1	3
   2	6
   3	9

6. Ask the students to come up with rules that describe the relation. If necessary, model coming up with a rule as a class. You could organise students to work in tuakana-teina relationships, and give students the option to work with the teacher for more direct support. For the relation above this might be, “There are three times as many cubes in the yellow cup as there are in each compared to the blue cup.” Algebraically, this might be recorded as y = 3b, though this is not a learning intention for students at this level. Using algebraic conventions to represent the rule for a pattern could be offered as an extension task. Choose a couple of relations for students to graph. Look at the pattern formed by points on the graph and ask students to explain why the graph is linear (it points in a straight line).
7. Pose real life scenarios for students to support them to understand the significance of relationships with variables. Model each scenario with cups and cubes, with a particular coloured cup representing the number of items possessed by each person.
   • Tammy is four years older than Hosepha. How old might both people be?
   • Anshul has three times as many toy cars as Kahu. How many toy cars might each boy have?
   • Altogether Liam and Moana have 24 video games. Moana has twice as many video games as Liam. How many games might each person have? (Note that zero values are not possible in this problem. Why?)

Session 2

In this session students explore the effect of operations on variables. Inverse operations are presented as “doing and undoing” processes.

1. Pose “think of a number” problems for students that result in some unexpected answers at the end. Encourage the students to reason why that occurs and discuss with a partner. The processes involved can be modelled using cups and cubes. Begin with a problem where the use of inverse operations is obvious. Note that the algebraic representation is not expected though you might use an empty box as n for a more accessible representation.

   Instructions	Cups and cubes model	Algebraically
   Think of a number		n
   Add five		n + 5
   Take away your starting number		5

   Ask: Why does everyone get an answer of five, no matter what starting number they thought of?
   Do your students realise that subtracting n undoes the adding of n in the first place?
    

2. Develop more complex examples. Encourage the students to model the steps with cups and cubes to explain the effect of the operations.
   For example:

   Instructions	Cups and cubes model	Algebraically
   Think of a number greater than 5		n
   Take away four		n – 4
   Double the answer		2 (n- 4) = 2n – 8
   Add ten		2n + 2
   Halve the answer		n + 1
   Take away your starting number		1
   Your answer is one!

   Instructions	Cups and cubes model	Algebraically
   Think of a number		n
   Add three to the number		n + 3
   Double the answer		2(n + 3) = 2n + 6
   Take away four		2n + 2
   Halve the answer		n + 1
   What number did you start with? Your final answer was one more than your starting number.

3. Discuss how each activity works. Look for students to explain that some operations undo others. In particular, subtraction undoes addition (and vice versa) and division undoes multiplication (and vice versa). 
   Tell your students that addition and subtraction, and multiplication and division are inverse operations. With the class, come up with a list of metaphors that describe inverse relationships (e.g. turning a light switch on and off).
   Students also need to recognise when an operation is only partly undone. For example, with 3n + 6 dividing by three will undo multiplying n by three, but the addition of six is not completely undone. Demonstrate this for students.
4. As a class, come up with a new (or several) “think of a number” problem(s) and represent the values in a table and graph. Highlight that the relationship on the graph is linear (i.e. is represented by a straight line). Emphasise the use of inverse operations, and draw on students’ experiences of working with cups and cubes. Keep the equations simple by using only two different operations, and numbers below 10 (e.g. 4n + 7). The letter ‘n’ can be used to keep the equation(s) consistent with what has previously been covered in the lesson.
5. Invite the students to develop their own “think of a number” problems. This could be done in pairs, small groups, or independently. Emphasise the use of inverse operations. At this stage, students are not expected to find the resulting answer of linear equations, rather they are expected to demonstrate their understanding of the effects of operations. Their attempts can be set as examples for other students to explain. Look for the following:
   • How complex are students’ attempts at inverse operations? In the early stages you will find that students tend to immediately undo a previous operation.
   • How comfortable are your students with variables being unknown?
   • Are they able to recognise the parts of an expression that are variable (the cups) and that are fixed (the cubes)?
   • Can they control variables and numbers under the operations? Division is particularly challenging. For example, 3n + 6 divided by three requires each part, 3 cups and 6 cubes, to be divided separately then recombined.

Session 3

In this session students model equality with cups and cubes models. They investigate operations that can be performed on both sides of the equation, that simplify the relationship while maintaining equality.

1. Begin with simple equalities such as:

   Each red cup has the same number of cubes and each blue cup has the same number of cubes. Which collection, left or right,  has the most cubes?
   Look for students to realise that structurally the collections are the same (Two blue cups and three red cups on each side). This can be provoked by putting different numbers of cubes into the cups, e.g. 3 cubes in each red cup, 8 cubes in each blue cup. The equality of the collections is preserved no matter what numbers are used.

2. Ask: Could I remove cups from both collections and the total number of cubes would remain equal? What could I do?
   Look for students to realise that taking away the same cups from each side leaves the equality maintained. Algebraically that is alike 3r + 2b = 2b + 3r so 2r + 2b = 2b + 2r (subtracting one red cup from each side)
   Also consider what cannot be done. For example, removing a red cup from the left collection and a blue cup from the right collection does not maintain the equality. 

3. Provide other examples where applying the same operation to both sides makes finding possible values for the variables much easier.

   Each yellow cup holds the same number of cubes as every other yellow cup.
   Each blue cup holds the same number of cubes as every other blue cup.
   The left collection of cubes equals the right collection of cubes.
   What numbers of cubes could be in the yellow and blue cups?
   
   
   Simplifying both sides by removing the same cups makes the problem much easier.
   
   In this case students are left with the equality that one blue cup equals two yellow cups (b = 2y). An infinite set of solutions like (0, 0), (2, 1), (4, 2),… is possible.
    

4. Get students to connect the concepts of variable and equality using group worthy problems like:

   
   Each cup of a given colour, in both collections, must contain the same number of cubes, e.g. all reds hold 4 cubes, all yellows hold 3 cubes.
   What numbers of cubes could be put in each cup so that the two collections contain the same total number of cubes?
   Students might realise that the number of cubes placed in the red cups is irrelevant to the problem since it is the equivalence of two yellow cups to one blue cup that determines whether or not the collections are equal. They are likely to arrive at this conclusion by experimenting and looking for commonality among the solutions.

5. Recording their solutions systematically will help:
   Solutions for r = 1                              Solutions for r = 2                 Solutions for r = 3
   y = 0 so b = 0                                      y = 0 so b = 0                         y = 0 so b = 0
   y = 1 so b = 2                                      y = 1 so b = 2                          y = 1 so b = 2
   y = 2 so b = 4                                      y = 2 so b = 4                          y = 2 so b = 4
   y = 3 so b = 6                                      ....                                          ....
    
6. Explore other group-worthy equivalence problems such as:
   •                              
     What numbers of cubes for each colour of cup will make each collection equal?
     (Two reds must equal one blue plus six)
     What is in common with these solutions? 
      
   •                                
     What numbers of cubes for each colour of cup make each collection equal? (One green plus one blue must equal one yellow plus three)

Session Four

In this session cups and cubes models are created to solve problems more traditionally associated with algebra. Students attempt to find specific unknowns from the information they are given.

1. Start with this problem:
   Manaia began the weekend with some dollars in his money jar.
   He worked all day on Saturday weeding Mr Barkley’s gardens.
   After he was paid Manaia saw he had five times as much money as he had to start with.
   On Sunday, he delivered advertising, and made an extra $12.
   By the end of the weekend he had a total of $72.
   How much money did he have at the start of the weekend?
2. Ask students: How could we use cups and cubes to help us solve the problem?
3. Students might suggest using a cup to represent the amount of money Manaia had at the start. They might then track what happens to the model as Manaia earns money.
   
   Starts with some dollars…      Then has five times as much…Then adds $12 to that amount
    
4. Set up an equality like this (Equivalent to 5m + 12 = 72):
   
    
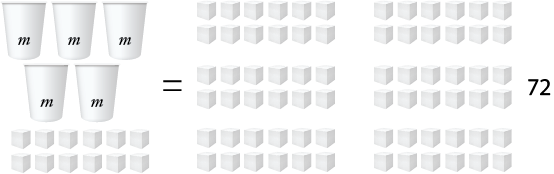
5. Ask: What can we do to make this equation simpler?
   Students should suggest some undoing, using inverse operations. The order is important. The undoing needs to occur in the reverse order to the first operations.
   Take $12 off each side: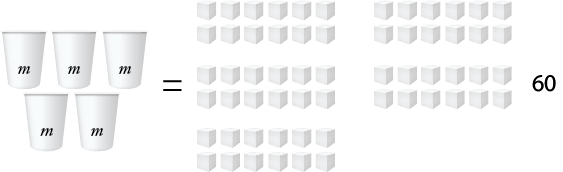
   
   Divide both sides by five:
   
    
6. Manaia had $12 at the start of the weekend. Check the answer by acting out the original problem. Put 12 cubes in each cup then work through five times as much then adding $12.
   Has Manaia got $72 or are we incorrect?
   You might use a calculator to check (5 x 12 + 12 = 72)
7. Pose this problem for the students to solve in pairs. The numbers are smaller, but the operations are more complex. This problem could be adapted to better reflect your classroom context.
   Jodie collected lemons to squeeze juice. She started with a few lemons.
   After she visited her Nana’s tree, Jodie had six times as many lemons as she had to start.
   She got another 15 lemons from her neighbour.
   Jodie’s Mum was a bit worried about her lemon collecting habit. She told Jodie to share her lemons equally with her two sisters.
   “That’s fair,” said Jodie, “I still have 13 lemons to make juice.”
   How many lemons did Jodie have at the start?
8. Watch as your students try to model the problem.
   • Do they set up a cups and cubes model or find another strategy, e.g. trial and improvement?
   • Do they control the order of the inverse operations?
   • Do they check to see that their solution meets the conditions of the problem?
9. Gather the class to discuss strategies, and check the solution of four for the starting number of lemons.
10. Give your students Copymaster 1 to work through.  They can use cups and cubes if they want to model the problem though drawing diagrams is a useful alternative.
11. Students share their strategies with the class and justify their thinking.

Session 5

In this session students use the cups and cubes model to solve problems from growth patterns. Matchsticks are used to create the figurative patterns.

1. Connect the students’ understanding of equivalent structures with rules to describe relations. Pose problems like:
   Petra and Clive are looking at this matchstick pattern: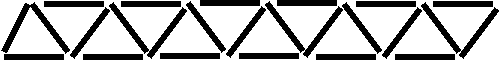
   
   They have worked out that it takes 25 matches to make 12 triangles in this way. Their teacher has challenged them to work out how many matches 100 triangles might take to make.
   What rules can you find to help Petra and Clive so they do not have to build 100 triangles?
   Students are likely to come up with different direct rules that describe the relation between the number of triangles and the number of matches. For example:
   • The first triangle took three matches. Each triangle after that took two matches. So to make 100 triangles takes 99 x 2 + 3 matches.
   • There was one match to start with and each triangle took two more matches. So 100 triangles takes 100 x 2 + 1 matches to make.
   • Each triangle takes three matches to make but when two triangles are joined there is one extra match. So to make 100 triangles takes 100 x 3 - 99 matches.
2. Ask the students to explain where the numbers come from in each person’s rule. Check to see that all three rules give the same answer to the number of matches. Use the explanations to model the structure of each rule using a cup to represent the chosen number of triangles.
   • Take one off the number of triangles (99), double it, and then add three. 
                            
      
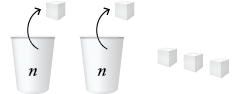
   • Take the number of triangles (100), double it, and then add one.
     
      
   • Take the number of triangles (100), multiply by three, then take away one less than the number of triangles (99). Note 99 is the number of joins of two triangles and taking away one less than n is like taking n away but leaving one behind.
     
     With the cups and cubes model it is easier for students to appreciate the structural similarity of the rules in that one model can be converted to the others. For example, in the first rule if two of the three cubes are used to replace the two cubes taken out then the model becomes the second model. This is equivalent to proving physically that 2(n-1) + 3 = 2n + 1
3. Provide the students with a related stick problem and ask them to find as many rules as they can. Tell them to then explain the equivalence of the rules using cups and cubes models. For example:
   
   How many matches would it take to make 20 houses in this pattern?
   Rules might include:
   • 19 x 5 + 7 (seven for the first house, five for each house after that)
   • 20 x 5 + 2 (five for each house plus two matches to start)
   • 20 x 7 – 19 x 2  (seven for each house less two matches for each join)

Session 6 (extension session)

In this session students learn how cups and cubes models can represent why number relationships work.

1. Get the students to apply the structural strategies they have to problems that involve proof. Begin with simple properties of consecutive numbers.
   Take any three consecutive numbers, e.g. 3, 4, 5.
   If you add the numbers (3 + 4 + 5 = 12), this equals three times the middle number (3 x 4 = 12).
   Does this work for any set of three consecutive whole numbers? Why?
2. Invite students to explain why the rule holds for any set of consecutive whole numbers, and to model their explanation with the cups and cubes model.  Let n be the first number:
   
   Students may recognise that both collections total three lots of the first number plus three. Algebraically this could be written as, n + (n + 1) + (n + 2) = 3n + 3 = 3 (n + 1)
3. Pose another problem involving consecutive numbers and ask the students to explain why the property holds irrespective of which four numbers are chosen.
   Choose any four consecutive odd numbers, e.g. 3 + 5 + 7 + 9.
   Add the two outside numbers, e.g. 3 + 9 = 12.
   Add the two inside numbers, e.g. 5 + 7 = 12.
   Are the sums always equal no matter what consecutive odd numbers you choose? Why?
   Would this work for consecutive even numbers? Why?
    

4. Pose other more complex problems that can be modelled with cups and cubes.
   For example: 
   Choose any two single digit numbers, e.g. 3 and 8 (modelled by two different cups).
   Use the digits to make two different two-digit numbers,
   e.g. 38 and 83, 38 = 10 x 3 + 8, 83 = 10 x 8 + 3

   Add the two sums, e.g. 38 + 83 = 121
   Divide the answer by 11, e.g. 121 ÷ 11 = 11
   The result is always the sum of the two digits, e.g. 3 = 8 = 11.
   Does this always happen? Why?

   Hint: If b is the first digit and r is the second digit, then a model of 10b + r looks like this. 
   What will the model of 10r + b look like?

   
   Why will the sum of 10b + r and 10r + b equal 11 x (b + r)?