This series of lessons provides different contexts to explore multiplication concepts using arrays such as the one below. This array has 5 rows and 10 columns.

Session One: Getting started

1. We begin the week with the ‘Orchard Problem’. A picture book about gardens, such as Nana's Veggie Garden - Te Māra Kai a Kui by Marie Munro, could be used to ignite interest in this context.
   Jack the apple tree grower has to prune his apple trees in the Autumn. He has 6 rows of apple trees and in every row there are 6 trees. How many apple trees does Jack have to prune altogether?
   

The start of PowerPoint 1 shows the whole array. Show the complete array. Ask your students to open their eyes and take a mind picture of what they see. Click once to remove all the trees and ask your students to draw what their mind picture looks like. One child could draw their picture on the whiteboard. This could then be referred back to throughout the rest of the lesson.

Look to see if they attend to the rows and columns layout even if the numbers of trees have errors. Discuss the layout.

1. Have a pile of counters in the middle of the mat. Ask a volunteer to come and show what the first row of trees might look like. Or get 6 individuals to come forward and act like trees and organise themselves into what they think a row is.
   Alternatively click again in the PowerPoint so it’s easy for all to see what the first row of apple trees will look like. Ask your students to improve their picture if they can.
   What will the second row look like? 
   It’s important for students to understand what a row is so they can make sense of the problem. It is also important for them to notice that all rows have the same number of trees.
2. Arrange the class into small mixed ability groups with 3 or 4 students in each. Give each group a large sheet of paper. Ask them to fold their piece of paper so it makes 4 boxes (fold in half one way and then in half the other way).
3. Allow some time for each group to see if they can come up with different ways to solve the Orchard Problem and record their methods in the four boxes. Tell them that you are looking for efficient strategies, those that take the least work.
   Allow students to use equipment if they think it will help them solve the problem.
   Rove around the class and challenge their thinking with questions like:
   • How could you count the trees in groups rather than one at a time?
   • What facts do you know that might help you?
   • What sets of numbers do you know that might help you?
   • What is the most efficient way of working out the total number of trees?
4.  Ask the groups to cut up the 4 boxes on their large sheet of paper and then come to the mat. Gather the class in a circle and ask the groups to share what they think is their most interesting strategy. Place each group’s strategy in the middle of the circle as they are being shared. Once each group has contributed, ask the students to offer strategies that no one has shared yet. 

5. Likely strategies	Possible teacher responses
   1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11 …. Tahi, rua, toru, whā, rima, ono, whitu, waru, iwa, tekau, tekau mā tahi…	Can you think of a more efficient way to work out how many trees there are? How many trees are there in one row?
   6, 12, 18, 24, 30, 36	Do you know what 6 + 6 =? Or 3 + 3 = ?  Can that knowledge help you solve this problem more efficiently?
   6 x 6 = 36	What if Jake had 6 rows of trees and there were 7 trees in each row?
   6 + 6 = 12; 12 + 12 = 24; 24 + 12 = 36	You used addition to work that out.  Do you know any multiplication facts that could help?
   2 x 6 = 12; 12 + 12 + 12 = 36	If 2 x 6 = 12, what does 4 x 6 =? How could you work out 6 x 6 from this?
   3 x 6 = 18 and then doubled it	That is very efficient. Could you work out 9 rows of 6 for me using 6 x 6 = 36?
   5 x 6 = 30; and 6 more = 36

   The shared strategies can be put into similar groups.
   Who used a strategy like this one?

6. Show students PowerPoint 2. The PowerPoint encourages students to disembed a given smaller array of trees from within a larger array. They are also asked to use their knowledge of the smaller array to work out the total number of trees in the larger array. This is a significant ability for finding the totals of arrays using the distributive property of multiplication.
7. Provide your students with Copymaster 1. The challenge is to find the total number of trees in each orchard. Challenge your students to find efficient strategies that do not involve counting by ones.
8. As a class, share the different ways that students used to solve the Orchard Problems. You might model on the Copymaster to show how various students partitioned the arrays.

Sessions Two and Three: Exploring through work stations

The picture book Hooray! Arrays! by Jason Powe could be used to ignite interest in this learning. In the next two sessions students work in pairs or threes to solve the problems on Copymaster 2. Consider choosing these pairs to encourage tuakana teina through the pairing of more knowledgeable and less knowledgeable students. Enlarge the problem cards and place them at each station. Provide students with access to copies of Copymaster 3 and Copymaster 4 (arrays students can draw on), and physical equipment such as counters, cubes, and the Slavonic Abacus.

Read the problems from Copymaster 2 to the class one at a time to clarify the wording. You may need to revisit the meaning of rows and columns by creating simple examples.
As students work on a station activity, ask them to create a record of their thinking and solutions. The record might be a recording sheet or in their workbook. Note that Part 2 of each problem is open and requires a longer period of investigation.

As the students work watch for the following:

• Can they interpret the problem wording either as a physical representation or as symbolic equations?
• Do they create arrays of equal rows and columns?
• Are they able to use skip counting, additive or multiplicative strategies to find the total number of trees?
• Do they begin to see properties of whole numbers under multiplication? (for example, Apple Orchard Part 2 deals with the commutative property)

At times during both sessions you might bring the class together to discuss confusions or misconceptions, clarify language and share efficient strategies and ways of representing the problems.

Below are specific details related to each problem set.

Orange Orchard

Orange Orchard (Part 1) involves 6 x 8 (or 8 x 6). Students might use their knowledge of 6 x 6 = 36 and add on 12 more (two columns of six). That would indicate a strong understanding of the multiplicative structure of arrays.

Most students will use strategies that involve visualising the array and partitioning the array into manageable chunks (dis-embedding). For example, they might split rows of eight into two fours (6 x 8 = 6 x 4 + 6 x 4), or into fives and threes (6 x 8 = 6 x 5 + 6 x 3). Other students will use less sophisticated strategies such as counting in twos and fives, or a combination of skip counting and counting by ones.

Part 2 is an open task which requires students to identify the factor pairs of 24.

Encourage capable students to be systematic in finding all the possibilities (1 x 24, 2 x 12, 3 x 8, 4 x 6).

Kiwifruit Orchard

Part 1 requires students to coordinate three factors as the problem can be written as 3 x (4 x 5). Multiplication is a binary operation so only two factors can be multiplied at once. Do your student recognise the structure of a single orchard (4 x 5) and realise that the total is consists of three arrays of that size?

Similarly, in Part 2 students must restructure 36 plants into two sets. Do they partition 36 into two numbers, preferably that have many factors? The problem does not say that the two orchards must contain the same number of plants though 18 and 18 is a nice first solution. Once the two sets of plants are formed can your students find appropriate numbers of rows and columns that equal the parts of 36?

Strawberry Patch

Part 1 is a single array (5 x 12). Students might use the distributive property and solve the problem or 5 x 10 + 5 x 2 (partitioning 12) or 5 x 6 + 5 x 6. Some may re-unitise two fives as ten to create 6 x 10. These strategies are strongly multiplicative. Most students will use smaller units such as fives or two and apply a combination of repeated addition (5 + 5 = 10, 10 + 10 = 20, etc.) or skip counting (2, 4, 6, 8, …).

Part 2 is about factors that have the same product (24). This gives students a chance to recognise that some numbers have many factors and the expressions of those factors have patterns. For example, 6 x 4 and 3 x 8 are related by doubling and halving. The logic behind the relationship may be accessible for some students. If the rows are halved in length, then twice as many rows can be made with the same number of plants.

Apple Orchard

Part 1 gives students a chance to ‘discover’ the commutative property, the order of factors does not affect the product. In this case 5 x 10 = 10 x 5.

Part 2 applies the distributive property of multiplication though many students will physically solve the problem with objects. Look for students to notice that 12 extra trees shared among six rows results in two extra per row. So, the number of rows stays the same, but the rows increase in length to six trees. Similarly, if more rows are made the 12 trees are formed into three rows of four. The number of rows would then be 9. 6 x 6 and 9 x 4 are the possible options.

Sessions Four and Five

Sessions Four and Five give students an opportunity to recognise the application of arrays in other contexts.

The chocolate block problem involves visualising the total number of pieces in a block even though the wrapping is only partially removed. PowerPoint 3 provides some examples of partially revealed chocolate blocks. For each block ask:

• How many pieces are in this block?
• How do you know?

Look for students to apply two types of strategies, both of which are important in measurement:

Iteration: That is when they take one column or row and see how many times it maps into the whole block.

Partitioning: That is when they imagine the lines that cut up the block, particularly halving lines. They look to find a partitioning that fits the row or column that is given.

Copymaster 5 provides students with further examples of visualising the masked array.

The Kapa Haka problem is designed around the array structure of seating arrangements for Kapa Haka performances at school.

Begin by role playing the Kapa Haka problem. Use chairs to make a simulated arrangement of seats. You might like to include grid references used to locate specific seats.

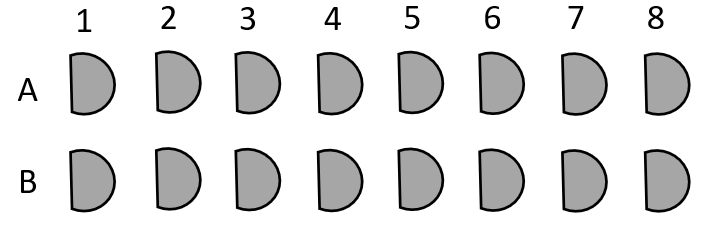
Try questions like:

• How many rows are there? How many columns are there?
• How many audience members could be seated altogether?
• If the performance needed 24 seats what could they do?

Use different arrangements of columns and rows.

Give the students counters, cubes or square grid paper to design possible seat layouts with 40 seats. Encourage them to be systematic and to look for patterns in the arrangements. Some students will find efficient ways to record the arrangements such as:

2 rows of 20 seats                4 rows of 10 seats                5 rows of 8 seats

Record these possibilities as multiplication expressions on rectangles of card. Put pairs of cards together to see if students notice patterns, like doubling and halving.

It is important to also note what length rows do not work.

• Could we make rows of 11 sets? 9 seats? Why not? (40 is not divisible by 11 or 9 as there would be remaining seats left over)

If students show competence with finding factors, you could challenge them to find seating arrangements with a prime number of seats such as 17 or 23. They should find that only one arrangement works; 1 x 17 and 1 x 23 respectively.

Reflecting

As a final task for the unit, ask the students to make up their own array-based multiplication problems for their partner to solve.

1. Tell the students that they are to pretend to be kūmara growers. They decide how many rows of kūmara plants they want in each row and how many rows they will have altogether. As part of this learning, you could look into how early Maori people grew kūmara. This plant arrived in New Zealand with Polynesian settlers in the 13th Century. However, the climate here was much colder than the Polynesian islands. As a result, the kūmara had to be stored until the weather was warm enough for it to grow. The kūmara plant became even more important once settlers discovered that some of their other food plants would not grow at all in New Zealand’s climate. These kūmara were different to the ones we eat today - which came to us from North America. The books Haumia and his Kumara: A Story of Manukau by Ron Bacon, and Kumara Mash Forever by Calico McClintock could be used to engage students in this context.
2. Then they challenge their partner to see if the partner can work out how many kūmara plants they will have altogether.
3. Tell the students to create a record of their problem with the solution on the back. The problems could be made into a book and other students could write other solution strategies on the back of each problem page.
4. Conclude the session by talking about the types of problems we have explored and solved over the week. Tell them that the problems were based on arrays. Let them know that there are many ways of solving these problems, tough multiplication is the most efficient method. Ask students where else in daily life they might find arrays.

Session 1

In this session students revise how to calculate the area of a rectangle. The knowledge covered in this session is foundational to understanding how to calculate the area of a triangle.

1. Begin with a discussion around the question “what is area?”. Encourage students to differentiate between perimeter and area, and to identify contexts in which measuring area is needed (e.g. construction, pattern-making, planning a sports event). Ensure that students understand area as a measure of the size of a two-dimensional surface that is measured in square units - square metres (m²), square millimetre (mm²), square centimetres (cm²).
2. Draw an unlabelled rectangle on the board and ask students to tell you what its area is.
3. If students are unsure of what they need to do to work out the area of the rectangle, ask them to tell you what features of the shape they could measure (i.e. length, height). 
4. If they say they could measure “two sides” then label two opposite sides and see what they say. It is important that students realise that they need to know the base and the height, or two sides at right angles to each other, to be able to find the area of a rectangle.
5. If students can correctly work out the area of the rectangle from its base and height measurements ask them to describe their method and explain why it works. If nobody can give an explanation then a brief discussion is in order. Drawing a grid to link the concept of area with the array model of multiplication may help to clarify students’ understanding.
   
6. Encourage students to use any multiplicative strategies they are confident working with to multiply the base and height measurements. You could also use this as an opportunity to reinforce a target multiplication strategy. 
   How did we work out the area of the rectangle?
   How did we work out 5x7? 
7. Ensure that students have a clear understanding that the area of a rectangle equals its base times its height. Ensure that correct units are used; if the lengths are labelled in centimetres then the area has to be given in square centimetres (cm²), if the lengths are not given units then the area should be given in square units (units²).

Session 2

In this session students divide rectangles diagonally to produce right angled triangles. They recognise that the two triangles formed are equal in area.

1. Ask students to cut a rectangle out of grid paper, ensuring that its base and height are whole square amounts. (You may want to put limits on the size of the rectangle depending on the ability of your students – eg. No more than 10 squares along each side.)
    
2. Ask students to work out the area of their rectangle (remind them to record their answers in units²). Ask students to explain their method to a partner.
3. Ask students to rule a line from one corner of their rectangle to the diagonally opposite corner. Model how to do this, emphasising the accurate use of a ruler. Ensure that they can see the two right angled triangles they have created. If students are unfamiliar with the term ‘right angle triangle’, explain to them that this type of triangle is called a ‘right angle’ triangle because the space between the two arms of the angle (point to the arms) is 90 degrees. We can mark a 90 degree angle with a small square in the corner. This shows us that this angle is exactly 90 degrees.
   
4. Ask them to find the area of each triangle. They may need to count all the squares within the triangles.
   What do you notice about the areas of the two triangles?
   Students should notice that the two triangles have the same areas and that therefore the area of each is half the area of the rectangle. If they had worked out the areas by counting, ask them to use multiplication to check their counting.
   What is the area of each triangle exactly?
   How could you work out the areas of the triangles? [Halve the area of the rectangle]
   What numbers would you need to multiply or divide? [Either base times height times half or base times height divided by two.]
   What would be the easiest strategy for you? [Initially students are likely to mistakenly think that they have to multiply the base and height before dividing - often a better strategy is to halve one of these factors before multiplying.]

5. Have students cut along the diagonal and rotate one triangle to sit on top of the other. Emphasise that the triangles are identical, meaning that they must have the same area.
   

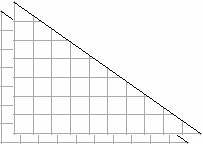
   Ask: Will this work for any rectangle you can make?

6. Students should be given the opportunity to experiment with a few different rectangles so that they can see that the rule holds true for any rectangle. They can work with a partner to discuss and justify their findings. If necessary, they can work with a small group to consolidate understanding

Session 3

In this session students draw right angled triangles, complete the rectangle and calculate the area of the original triangle.

1. Draw a right angled triangle on the board; label its base 10cm and its height 5cm.
    
2. Ask students whether they can tell you its area (remind them of the correct units – cm²).
3. Discuss suggestions for how you could work out its area.
   Do we have enough information to work out the area?
   Could we work out the area of another shape that would help?
   Remember what we discovered about rectangles last maths lesson – two identical right angle triangles can be positioned together to make a rectangle - could that help?
4. Ask students to draw a triangle on their grid paper so that two of its sides are along lines of the grid paper. (You may want to put limits on the size of the triangle depending on the ability of your students – eg. No more than 10 squares along each side.)
   
5. Now get them to draw the matching triangle that makes a rectangle.  Draw the triangle to make a rectangle on your diagram on the board to illustrate.
6. Challenge students to work out the area of their completed rectangle and then that of the triangle.
7. Get each student to complete several triangles to ensure they are doing it correctly. Ask them to show their triangles to a partner. Their partner should confirm whether or not the student has drawn the triangles correctly, worked out the area of the rectangle, and correctly worked out the area of the triangle.
8. Return together as a class to discuss:
   Does this work for every right angled triangle?
   Can you describe a rule for the area of a right angled triangle?
   Are there any clever tricks to make the maths easier? [Divide one of the sides by two before multiplying]
9. Get students to record a rule for the area of right angled triangles in their own words. Students may see that the area of any right angled triangle is equal to the area of the rectangle with the same base and height divided by two. Get them to record the statement “For right angled triangles, area equals half base times height.” 

Session 4

In this session students draw non right angled triangles, and experiment with finding their area.

1. Draw an unlabelled non right angled triangle on the board. Draw one of its sides horizontal. 
   
2. Ask students what information they will need to be able to work out its area.  It is likely that students will try to apply their learning from the previous session and tell you that they need to know the length of two sides.
3. Ask students to draw a triangle of their own on grid paper, so that all three corners are on grid intersections, but only one of its sides is along a line of the grid.
4. Now challenge them to find its area by measuring two sides.
5. If they apply their rule “area equals half base times height”, ask them to draw the rectangle to illustrate. They will be unable to.
6. Bring the class back together and discuss why it does not work. Emphasise that in a non-right angled triangle the height is not equal to either of the sides. Discuss this then send students to try to find a rectangle that will work for their triangle.
7. When most students have identified that the height of the rectangle needs to be at right angles to the base since rectangles have all right angles bring the class back together.
8. Draw the diagram below on the board and ask students whether the rectangle is twice the size of the triangle. 
   
9. If students cannot see that the two smaller triangles join to make the larger triangle, add an extra line as illustrated below.
   
   What is the area of the left hand rectangle?
   What is the area of the left hand part of the right angled triangle?
   What is the area of the right hand rectangle?
   What is the area of the right hand part of the right angled triangle?
10. Give students time to draw some of their own triangles with only one side along a grid line, and work out their area. Ensure that they are including units in their answers.
11. Return together as a class to discuss:
    Does this work for every triangle?
    Can you describe a rule for the area of any triangle?
12. Get students to record a rule for the area of right angled triangles in their own words. Emphasise that the area of any triangle is equal to the area of the rectangle with the same base and height divided by two. Get them to record the statement “For all triangles, area equals half base times height.” refer back to the diagram to consolidate understanding.

Session 5

In this session students state a rule for the area of a triangle and use it to find the area of some triangles.

1. Begin the lesson by asking students to tell you the rule for the area of a triangle. Confirm that it is "area equals half base times height".
2. Have students complete the triangle area worksheet (Copymaster 1) individually, in pairs or as a class. This should give you a good idea of any students who need further support to understand the key concepts.
3. As an extension. challenge students to find triangles in the classroom or in the wider school environment and make the measurements required to calculate their area. Provide measuring equipment as required. Insist on correct units. During this time, you could work further with students in need of more support. Follow the sequence of the prior sessions, focussing on specific gaps in knowledge.
4. Ask students to challenge each other with triangles to calculate the areas of. Compare answers and strategies for measuring and calculating and discuss differences.