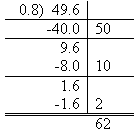Getting Started

The purpose of this session is to explore the range of strategies that students have to solve multiplication and division problems. This will enable you to elicit the strategies that students currently use and evaluate which strategies need to be focused on in greater depth as well as identifying students in your group as "expert" in particular strategies. Consider adapting the contexts reflected in the problems presented to better relate to the cultural backgrounds, interests, and learning from other curriculum areas that are relevant to your students.

After posing each problem, provide time for students to work out the answer. Encourage them to work out the answers mentally. If the use of materials is necessary for students, encourage them to work towards imaging and folding back to materials (as opposed to only relying on materials). Provide calculators for students to use as a way to check their calculations. Ask students to share their solutions and strategies with a partner. Alternatively, you might group students who have used the same strategy, and have them share their work together, before sharing with the class. 

As a class, discuss the strategies used. As different strategies arise ask the students to explain why they chose to solve the problem in that way. Have them model the strategy for the class. Accept all the strategies that are elicited at this stage, recording them to reflect upon later in the unit (perhaps in a modelling book or on a class chart).

Students who solve these problems quickly could be challenged to prove their solution with other strategies, or could be asked to make up similar problems.

Problem 1:
Shawna swims 0.5 kilometres each day for 28 days. How many kilometres has she travelled by the end of the five days?

Rounding and Compensating: 
28 x 0.5
28 is rounded to 30, so the equation becomes 30 x 0.5 (15). Then 2 x 0.5 (1) must be removed to complete the equation.

Proportional adjustment: 
28 x 0.5
Solve instead 14 x 1 - doubling and halving

Place value partitioning: 
28 x 0.5
Solve (20 x 0.5) + (8 x 0.5).

Problem 2:
Ruataniwha House are selling bags of nuts for a fundraiser. They buy a sack of nuts weighing 27kg, and repackage the nuts into 0.45 kg bags for sale. How many bags will come from the sack? (27 kg ÷ 0.45 kg)

Place Value Partitioning (Chunking): 
27 ÷ 0.45
10 bags would make 4.5 kg, so 20 bags would make 9 kg. 3 lots of 9 is 27… they can make 60 bags.

Proportional adjustment: 
If I double both sides I get 54 ÷ 0.9. 54 ÷ 9 is 6, so 54 ÷ 0.9 must be 60.

Ask students to reflect on the strategies that have been discussed in the session and evaluate which strategies that they personally need further work on, perhaps using thumb signals - thumbs up - confident and competent with the strategy, thumbs sideways - semi confident, and thumbs down - not yet confident. Use this information to plan for your subsequent teaching, using ideas from the exploring section outlined below.

Exploring

Over the next two to three days, explore the following strategies, making explicit the strategy you are concentrating on and your reason for doing so.

e.g. In the problem 2.9 x 7, rounding and compensating would be a useful strategy to use because 2.9 is close to 3.

Each day follow a similar lesson structure to the introductory session, with students sharing their solutions to the initial questions and discussing why these questions lend themselves to the strategy being explicitly taught. Conclude each session by having students make some statements about when this strategy would be useful and why (e.g. "place value is useful when there is limited renaming required" or "factorisation is useful when one of the factors is able to be renamed as a series of smaller factors"). It is important to record these key ideas as they will be used for reflection at the end of the unit.

The following questions are provided as examples for the promotion of the identified strategies. If the students are not secure with a strategy you may need provide extended opportunities for practise, using your own questions, to address student needs.

Consider providing a means for all students to record their thinking, from throughout the unit, in a structured way (e.g. a Think Mat, graphic organiser, mind map, booklet).

If necessary, use and provide materials to model problems and support students' thinking.

Rounding and compensating (Multiplication) 
Bozo the Clown fires 4.8 L of water from his water pistol each night at the circus. How many litres does he fire over two weeks?

The tidy numbers strategy involves rounding a number in a question to make it easier to solve. In the above question 4.8 can be rounded to 5 (by adding 0.2). The problem then becomes 5 x 14. The 14 groups of 0.2 L added to ‘tidy’ the problem now need to be subtracted, leaving a total of 67.2 L.

The following questions can be used to elicit discussion about the strategy:

• What tidy number could you use that is close to one of the numbers in the problem?
• What do you need to do if you tidy up this number? Why?
• Why is this strategy useful for this problem?
• What knowledge helps you to solve a problem like this?

Place value equipment can be also be used to show the problems physically. 

Further questions for practice:
6.9 x 9
1.48 x 7
13.98 x 6
12.96 x 32
7 x 9.998
5.48 x 3

Note that the problems posed here are using a tidying up strategy rather than tidying down. If one of the factors is just over a tidy number (such as 203) then place value tends to be a more useful strategy.

Rounding and compensating (Division) 
Ohau House uses 0.7L of detergent every time they do a fundraising car wash. They have 13.3L of detergent left… for how many car washes will it last?

Rounding and compensating for division involves finding a number that is close to the total, and working from that number to find an answer. For the question above, a student might say:

I know that 0 .7 times 20 would be 14. 13.3 is 0.7 less than 14, so the detergent would last 19 car washes.

The following questions can be used to elicit discussion about the strategy:

• What tidy number could you use that is close to one of the numbers in the problem?
• What do you need to do if you tidy to this number?
• Why is this strategy useful for this problem?
• What knowledge helps you to solve a problem like this?

Further questions for practice:
34.3 ÷ 7
19.8 ÷ 9
119.4 ÷ 6
13.3 ÷ 0.7
1683 ÷ 1.7

Proportional Adjustment (Multiplication) 
Bob the bodybuilder adds 2.5 kg to his weightlifting bar each day. How much will he have added after 32 days?

Proportional adjustment involves using knowledge of multiples to create equivalent equations. Factors are proportionally adjusted to make one (or both) factors easier to work from. In the above problem the factors could be adjusted as follows: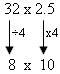

Alternatively, students might double the 2.5 to 5, and halve 32 to 16.

The following questions can be used to elicit discussion about the strategy:

• What could you multiply one of these numbers by to make it easier to work with?
• What would you then need to do to the other number?
• Why is this strategy useful for this problem?
• What knowledge helps you to solve a problem like this?

Further questions for practice:
3.33 x 18 (thirding and trebling)
60 x 7.5
300 x 1.8 (thirding and trebling)
120 x 2.25
24 x 1.25

Proportional Adjustment (Division) 
Jonno has 168m of rope to cut into 1.2m lengths. How many bits of rope can he make?

In division proportional adjustment involves reducing or increasing both numbers in the equation by the same number. Therefore, the numbers used to proportionally adjust the problem must be factors of both numbers in the equation. For example,
If I divide the 168 and the 1.2 by 2 my equation becomes 84 ÷ 0 .6 and I can divide them both by 2 again to get 42 ÷ 0 .3 which is 140 or I could divide them both by 4 to get the same equation.

The following questions can be used to elicit discussion about the strategy:

• What could you divide both of these numbers by to make an easier equation?
• Why is this strategy useful for this problem?
• What knowledge helps you to solve a problem like this?

Further questions for practice:
180 ÷ 1.5 (→ 360 ÷ 3)
367.5 ÷ 3.5 (→ 735 ÷ 7)
196.2 ÷ 18 (→ 98.1 ÷ 9)
1498 ÷ 1.4 (→ 749 ÷ .7)
172.8 ÷ 16 (→ 86.4 ÷ 8)

Place Value Partitioning (Multiplication) 
Mae Ling uses 3.12m to make a traditional dance outfit. How much fabric will she use to make 8 outfits?

The place value strategy involves multiplying in place value (e.g. ones, tenths and hundredths). In the above problem the student might say the following:
I multiplied 3 x 8 and got 24. Then I added the 0.8 (0.1 x 8) and 0.16 (0.02 x 8) to get 24 .96 m

The following questions can be used to elicit discussion about the strategy:

• How can you use your knowledge of place value to solve this problem?
• Why is this strategy useful for this problem?

Further questions for practice:
613.23 x 30
7 x 4.1112
10.21 x 40
354 x 0.11

An extension of the place value strategy involves the use of standard written form for multiplication.

Place value partitioning (division) 
Sheila mixes 0.8kg of milk powder with water each time she feeds the calves. If there is 49.6kg left in the bag, for how many feeds will the milk powder last?

The place value partitioning strategy for division involves ‘chunking’ known facts and subtracting them from the answer. The long division written form will be familiar to most teachers. In the case above, a student might think:

Ok, 10 lots would be 8kg. That means 50 lots would be 40kg. That leaves me with 9.6 kg. So if I take off another 10 lots that’s another 8kg. That leaves me with 16 kg left over, or 2 lots. So the answer is 50, plus 10, plus 2… 62 feeds!

This thinking could be recorded as:

The following questions can be used to elicit discussion about the strategy:

• How can you use your knowledge of place value to solve this problem?
• Why is this strategy useful for this problem?

Further questions for practice:
67.6 ÷ 0.4
97.6 ÷ 0.8
380.8 ÷ 0.7
472 ÷ 1.5
546 ÷ 1.8

An extension of the place value strategy involves the use of standard written form for division.

Factorisation (multiplication and division) 
Maree is putting up a 148.8m long fence. She places a post every 8m. How many posts does she need?

The factorisation strategy involves using factors to simplify the problem. In this instance eight can be factorised as 2 x 2 x 2. This means dividing by two, then two, then two has the same net effect as dividing by 8. Likewise, multiplying by two, then two, then two has the same net effect as multiplying by 8. In applying factorisation to the above problem, a student might think:

Dividing by 8 is the same as dividing by 2, then 2, then 2. So, 148.8 ÷ 2 = 74.4. Then 74.4 ÷ 2 = 37.2. And last, 37.2 ÷ 2 = 18.6. The answer is 18.6m.

The following questions can be used to elicit discussion about the strategy:

• How can you use your knowledge of factors to solve this problem?
• Why is this strategy useful for this problem?

Further questions for practice:
53.2 ÷ 8 (÷2, ÷2, ÷2)
3.48 ÷ 12 (÷2, ÷2, ÷3)
43.2 ÷ 27 (÷3, ÷3, ÷3)
1.35 x 12 (x2, x2, x3)
4.3 x 8 (x2, x2, x2)
2.7 x 16 (x2, x2, x2, x2)

Reflecting

To conclude the week's work, give the students the following five problems to solve asking them to predict which strategy they think will be useful for each problem and why they think this is the most efficient strategy before they solve them. After they have solved the problems engage in discussion about the effectiveness of their selected strategies for the problems. It is fine if students do not agree with the group about the usefulness of a particular strategy in a given problem. Encourage them to justify their thinking and model the use of their preferred strategy. 

Problems for discussion (more than one strategy might be suitable for these) 
68 x 3.5 (proportional adjustment)
46.2 ÷ 1.4 (place value partitioning)
2.93 x 6 (rounding and compensating)
169.5 ÷ 1.5 (proportional adjustment)
23.2 x 11 (place value partitioning)
7.04 ÷ 8 (factorisation)
161.7 ÷ 1.63 (rounding and compensating)
4.11 x 16 (factorisation)

Ask the students to create problems for a partner where one of the strategies covered in this unit is the most useful.

Conclude the unit by showing the students the questions asked in the initial session again. Have students discuss whether they would solve them in a different way now, and explain why. Review the modelling book/chart or record of statements or generalisations about the strategies and make any changes.