The purpose of this activity is to engage students in recognising patterns in recurring decimals, and linking the size of those decimals to the division of 100 that produces them.
This activity assumes the students have experience in the following areas:
- Applying the properties of whole numbers under multiplication and division.
- Understanding and applying the place value of decimals in calculation.
The problem is sufficiently open ended to allow the students freedom of choice in their approach. It may be scaffolded with guidance that leads to a solution, and/or the students might be given the opportunity to solve the problem independently.
The example responses at the end of the resource give an indication of the kind of response to expect from students who approach the problem in particular ways.
When 100 is divided by a whole number sometimes the answers are interesting decimals.
If you calculate 100 ÷ 11 you get this display on a calculator:
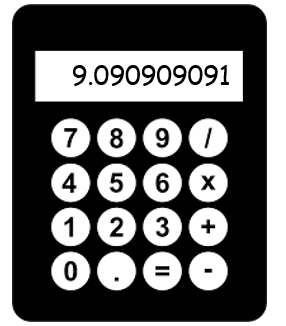
The pattern would carry on forever if the calculator screen was big enough. The 1 at the end comes from rounding.
Here are some other decimal patterns that come from dividing 100 by a whole number. What number is 100 divided by to get each pattern?

What other decimal patterns can you find by dividing 100 by a whole number?
The following prompts illustrate how this activity can be structured around the phases of the Mathematics Investigation Cycle.
Make sense
Introduce the problem. Allow students time to read it and discuss in pairs or small groups.
- How are the number patterns in the display created?
- What missing number do I need to find in each case? (The divisor.)
- What do I know about multiplication and division for other problems I have solved?
- Which examples would be the easiest to try first?
Plan approach
Discuss ideas about how to solve the problem. Emphasise that, in the planning phase, you want students to say how they would solve the problem, not to actually solve it.
- Which examples would be the easiest to try first?
- How big is the divisor in each case? Make an estimate. How do you make an estimate?
- Why do the quotients (answers) result in non-terminating decimals?
- Which strategies should I pursue? (Can I progress from trial and improvement to a more efficient method?)
- What tools (digital or physical) could help my investigation? (Can I configure the column width in my spreadsheet to get more digits?)
Take action
Allow students time to work through their strategy and find a solution to the problem.
- Have I shown my workings in a step-by-step way?
- Have I organised my examples in a way that helps me to see pattern?
- Is there a pattern? If not, is there still something going on in all cases?
- Can I move beyond specific examples to find an algorithm that works all the time?
Convince yourself and others
Allow students time to check their answers and then either have them pair share with other groups or ask for volunteers to share their solution with the class.
- What is the solution to the cases I am given?
- What other cases did I try?
- How would I find n unknown divisor if the numbers were whole numbers? Would that strategy work with decimals as well?
- Have I considered all possible cases to come up with an algorithm that always works?
- Which representations (text, table, graph, numbers, diagram, equations) will be easy to understand and will help me justify my generalisation?
Examples of work
Work sample 1
The student uses trial and error approaches to find the missing divisor.
Click on the image to enlarge it. Click again to close.
Work sample 2
The student applies the properties of multiplication and division, particularly the inverse property to find the missing divisor and creates other patterns by systematically trialling divisors.
Click on the image to enlarge it. Click again to close. 
Conceptually focused students are likely to use their understanding of divisibility to choose appropriate divisors in the search for patterns.
Click on the image to enlarge it. Click again to close.
If students have access to computer spreadsheets, they can find all the quotients easily and look for recurring patterns. Students might notice that multiples of four and five mostly give terminating decimals while multiples of three, seven and eleven usually give recurring patterns. They may recognise that recurring decimals occur when any prime factors of the divisor are not factors of 100. For example, 15 = 3 x 5 (3 is not a factor of 100) and 14 = 2 x 7 (7 is not a factor of 100).

