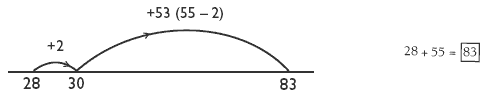Maths Detective

This activity is designed to encourage students to choose from a broad range of mental strategies by exploring how a subtraction problem can be solved in at least six different ways. It also gives students opportunities to compare the efficiency of strategies when they are applied to different problems, to interpret various recording techniques, and to be exposed to ways of describing solution paths.
This activity would be a useful follow-up for students who have just learned a range of additive strategies because it encourages them to explore the concept of “efficiency” and when each strategy might be useful. Note that for many calculations, several different strategies can be equally efficient.
Students need to be at least advanced additive part–whole thinkers (stage 6) to do this activity.
They need to be familiar with the following part–whole strategies:

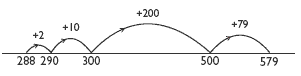
• Adding on by jumping to tidy numbers on a number line.For example, for 28 +  = 83:

28 + 2 = 30, 30 + 50 = 80, 80 + 3 = 83, 2 + 50 + 3 = 55
or in just two jumps:

28 + 2 = 30, 30 + 53 = 83, 2 + 53 = 55

• Adding or subtracting tidy numbers and then compensating. For example, for 28 +  = 83:

28 + 60 = 88, 88 – 5 = 83
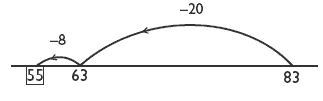
or for 83 – 28 = :

83 – 30 = 53, 53 + 2 = 55

• Making equal adjustments to give a tidy number that’s easier to work with.

For example, for 83 – 28 = :

Add 2 to 83 and 28 to make 28 a tidy number: 85 – 30 = 55.

• Re-partitioning to make one number a tidy number. For example, for 28 + 55 = :

Take 2 from the 55 and add it to the 28 to make a tidy number 30 + 53 = 83

• Using place value partitioning to break up the numbers. For example, for 83 – 28 = :

83 – 20 = 63, 63 – 8 = 55 (or 83 – 20 = 63, 63 – 3 = 60, 60 – 5 = 55)
Another possibility is 80 – 20 = 60, 3 – 8 = -5, 60 – 5 = 55. Note the significance here of going “back through a 10” to solve 63 – 8.

• Reversing a problem to change it from subtraction to addition or vice versa.For example: 83 – 28 =  → 28 +  = 83.

Students working independently could discuss parts of this activity in pairs or groups of 3–4. Before they start the activity, you could ask:
Why is it a good idea to know lots of different strategies for solving problems? (Although one strategy might work for lots of problems, other strategies will sometimes be better. If you only use one strategy all the time, you might be taking longer than you need to or finding the mental calculations harder than you should. Also, it might be useful to use a different strategy to check an answer.)
When a strategy is “efficient”, what does that mean? (It has fewer steps than other strategies and you don’t waste your time doing extra working out.)
You may need to define these terms:
• Adjust: change a number by adding or subtracting a bit.
• Compensate: make a further change to cancel the effect of an earlier one.
• Place value: the value of the place each digit occupies. (For example, the 5 in 57 is in the tens place.)
• Tidy number: a number that makes the calculation easy and is close to a number ina problem. Usually, in addition and subtraction, we use numbers like 50, 90, 300,and 4 000.
 

With a guided teaching group, ask the students to solve Mrs Coey’s problem (579 – 288 = ) and to record how they solved it using number sentences or an empty number line. It’s a good idea to have place value materials accessible in case a particular strategy needs clarification or validation.
Encourage comparisons by asking questions such as:
What clues did you use to match the students to their strategies?
Which ones were the easiest to match? Which ones were the most difficult? Why?
Did the students in Mrs Coey’s class come up with any strategies that were different from ours?
Did we use any strategies that they didn’t?
Focus the students on recording methods:
When you’re recording your solution strategy on a number line, how can you make it easy for someone else to understand? Use your own ideas or ideas you saw from someone in your group or from the students in the book.
This activity gives students lots of opportunities to develop appropriate language to talk about their problem-solving strategies and to communicate their thinking clearly. It also helps them to come to a shared understanding of terminology such as “adding on”, “using place value”, “using a tidy number and compensating”, or “making equal adjustments”.
The students could make a “strategy toolbox” display by writing the name of each strategy onto a picture of a different tool. The metaphor of a toolbox may remind the students that particular strategies are likely to be suited to particular problems, even though they might be able to solve many problems with just one strategy. (You could probably use any of the tools in a toolbox to bang in a nail, but a hammer will be most efficient.) Keep the “toolbox” as a reference on the wall and use it to encourage the students to choose their strategy before they solve a problem and to justify that choice.
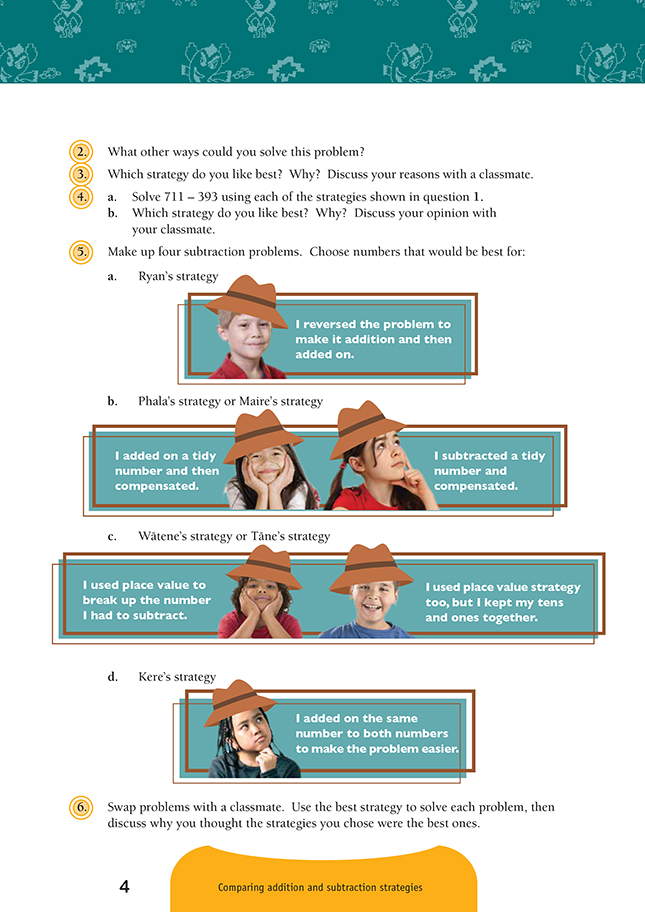
Question 6 is a useful formative assessment point to judge whether the students can explain why they’ve chosen particular numbers to suit certain strategies.
Some students are reluctant to use a variety of strategies and prefer to use one trusted method, even if it is less efficient. Try to give the students more practice in trying different strategies. Use phrases such as: Let’s all use Kere’s method to solve this next problem.
One way to encourage them to diversify their strategies is to deliberately choose numbers for problems that would be cumbersome for their preferred method but very quick using a different strategy. For example, 803 – 497 is easily solved using tidy numbers (803 – 500 + 3) or reversing (497 + 3 + 303).

Extension

In pairs, get the students to make a Wanted poster or to write a newspaper Situations Vacant ad that describes the characteristics of problems that can be solved efficiently using a particular strategy. Starters might include:
• Wanted! Problems that can be solved by making equal adjustments!
• We are looking for …
• These numbers can be recognised by …
 

These can be displayed for students to use as a reference to remind them to choose their strategies wisely.
 

Answers to Activity

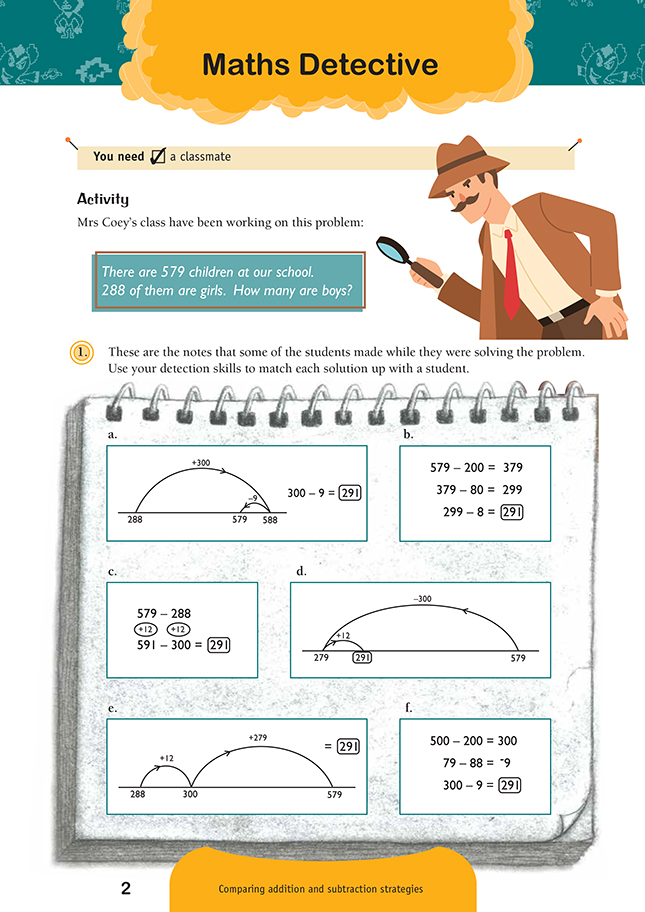
1. a. Phala
b. Wātene
c. Kere
d. Maire
e. Ryan
f. Tāne
2. Answers will vary. Some students may use the given strategies but add more interim steps, such as:

Ryan’s strategy could also be varied by subtracting in jumps to tidy numbers:

3. Answers will vary. All the strategies worked well.
For example, Ryan’s adding-on strategy (1e) is straightforward for keeping track of in his head; Kere’s equal adjustment strategy (1c) is concise and is a good use of number properties.
4. a. Phala (strategy 1a):

This step (793 – = 711) is difficult in your head.
Wātene (strategy 1b):
711 – 300 = 411
411 – 90 = 321
321 – 3 = 318
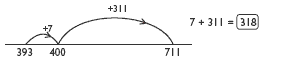
Kere (strategy 1c):
711 – 393
+7 +7
718 – 400 = 318

Maire (strategy 1d):

Ryan (strategy 1e):

Tāne (strategy 1f):
700 – 300 = 400
11 – 93 = -82
400 – 82 = 318
b. Answers will vary. Efficient strategies are Ryan’s way of reversing and adding on
(because it only takes two jumps), Maire’s tidy numbers and compensating (because
393 is close to a tidy number), and Kere’s equal adjustments strategy (again, because 393 is close to a tidy number). The other strategies work, but the mental calculations are trickier.
5. Problems will vary.
a. Ryan’s adding on strategy will suit most pairs of numbers.
b. Phala’s strategy of adding a tidy number and compensating will be straightforward if the starting number can be rounded up to a close tidy number. Maire’s strategy of
subtracting a tidy number and compensating will work well if the number being subtracted can be rounded up to a close tidy number.
c. Wātene’s place value strategy will be most efficient for numbers where the
corresponding digit in each place is smaller in the number being taken away, for example, in 584 – 362. Tāne’s place value strategy works most efficiently when the starting number can be rounded up to a close tidy number.
d. Kere’s equal adjustments strategy is simplest to use when the number being subtracted can be rounded up to a close tidy number.
6. Strategies and discussion will vary.