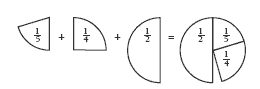Galloping Greyhounds

This activity provides a context in which students can see the need for a common denominator when adding or subtracting fractions. They use multiplication to find fractions of whole number amounts and make connections between fractions and ratios.
If the students are to do this activity independently, they will need to understand the significance of both the numerator and denominator, how a common denominator is used, and how to express parts of a whole as ratios.
Denominator:  The number on the bottom of a fraction is called the denominator because it specifies (or denominates) the unit value of the fraction. For example, 1/4,  2/4, 3/4, 4/4, 5/4, 9/4 and 15/4 are all fractions of the quarter kind (all multiples of 1/4). Compare the use of the word denomination to specify the face value of coins or banknotes.
Numerator The number on the top of a fraction is called the numerator because it tells us how many of that kind of unit fraction we have. 5/4, 5/5, 5/6, 5/12, and 5/100 are all different kinds (denominations) of fractions, but in each case, we have 5 of them.
Use the speech bubbles in the student book as a starting point for discussion. Ask “Why would this person choose to think of 3/5 as 12/20?” and “Why do we need to use a common denominator when adding or subtracting fractions?”
Ask your students to add together a number of Fraction Pieces that have the same denominator (are of the same denomination). For example:

Then ask them to add some pieces with unrelated denominators (that are of different denominations). For example:

Through discussion, reinforce the fact that adding fractions with different denominators is a little trickier.
Students are used to the idea that multiplying increases size or quantity. For this reason, when they multiply the denominators of two fractions to get a common denominator and then rewrite the fractions with the new denominator, they may believe that these fractions are larger than the originals. Fraction models can be used to help them see that this isn’t the case.

One method involves creating three overhead projector transparencies showing (i) a large square as a whole, (ii) the square divided into fifths, using four parallel lines, and (iii) the square divided into quarters, using three parallel lines. Superimposing the transparencies shows that twentieths are smaller pieces. You can use this grid to model all the fractions encountered in this activity.

A similar method involves paper folding (see Paper Partitions, pages 6–7 in the student book). In question 1b, students are asked to express the parts of the whole as a ratio instead of fractions. Fractions and ratios are closely related concepts, but students easily confuse the process by which the one can be expressed as the other. (The most common mistake is to think that 3/4 can be written as 3:4, when in the case of the fraction, the whole is divided into 4 parts and in the case of the ratio, it is divided into 7 parts.)
Because the three fractions add up to a whole (the prize pool), their common denominator shows how many parts the whole must be split into before it can be shared out in a ratio. The numerators of the three fractions: 12/20, 5/20, and 3/20, show the relative size of each share: 12, 5, and 3. This information is written as the ratio 12:5:3.

Your students may be puzzled as to why the two methods co-exist. Use the 5 by 4 grid illustrated above as the basis for discussion. Note:
• A fraction contains only information about itself: if Phil gets 3/5 of the prize pool, this tells us nothing about how many others share the pool or what part of it they receive.
• A ratio contains complete information about the sharing process: if we know that the prize pool is divided in the ratio 12:5:3, we know that there are 3 claimants and that each receives 12/20, 5/20, 3/20 or of the total. (Adding the parts of the ratio gives the denominator.)
Students need to learn to make sense of information expressed in either form.
Encourage your students to solve the parts of question 2 without a calculator. By doing this, they will further develop their proportional reasoning skills. Each of the prize pools divides neatly by 20 (as suggested by the speech bubble). Once the students have done this, all they need to do is multiply a single share (a twentieth) by the three numbers in the ratio to find what each person gets.
Suggest that the students look for short cuts when doing the calculations. For example, the answers to question 2c will be half as much again as the answers to question 2a because $900 is half as much again as $600. The $1,900 in question 2d may be thought of as $2,000 – $100 and the answers found easily in this way. The answers to question 2e can be found by adding the answers to questions 2a and 2d because $2,500 is $600 + $1,900.
In questions 3 and 4, students learn to write basic percentages as fractions and to find the value of 1/10 of the prizes for each place. Ensure that they can interpret question 3 and record a statement, an equation, or equations that will lead to a solution, for example: 1/10 x 3/5 x $800.
To solve question 4, students will need to be able to multiply two fractions together.

Answers to Activity

1. a. 3/20. (3/5 + 1/4 = 12/20 + 5/20 = 17/20; 1 – 17/20 = 3/20)
b. 12:5:3
2. a. $360, $150, $90
b. $450, $187.50, $112.50
c. $540, $225, $135
d. $1140, $475, $285
e. $1500, $625, $375
3. a. $48. (3/5 x 800 = $480. 10% of $480 = $48)
b. 3/50. (48/800 = 12/200 = 6/100= or 6%)
4. a. 1/40. ( 1/4 x 1/10 = 1/40 or 2.5%)
b. 3/200. (3/20 x 1/10 = 3/200 or 1.5%)