This problem solving activity has a geometry focus.
Ravi has designed plans for a castle that show the front, top, and side views.
Build a castle to Ravi’s design using the cubes.
What is the largest number of cubes that you can use in the construction of a castle from Ravi’s plans?
What is the smallest number of cubes that you can use in the construction of a castle from Ravi’s plans?
Can you build a symmetrical castle that is different from any of the ones you have built so far?
How many symmetrical castles can you build to Ravi’s specifications?
- Construct 3-D shapes from 2-D drawings.
- Describe the symmetries of 3-D shapes.
- Draw 3-D shapes on isometric paper.
- Devise and use problem solving strategies to explore situations mathematically (guess and check, use equipment).
This problem explores the relationship between 2-D and 3-D shapes and helps to visualise 3-D shapes from 2-D representations. It shows that different 3-D objects can be represented by the same set of 2-D objects. The problem also investigates the symmetry of objects.
- Copymaster of the problem (Māori)
- Copymaster of the problem (English)
- Small cubes
- Isometric dot paper
Problem
Ravi has designed plans for a castle that show the front, top, and side views.
Build a castle to Ravi’s design using the cubes.
What is the largest number of cubes that you can use in the construction of a castle from Ravi’s plans?
What is the smallest number of cubes that you can use in the construction of a castle from Ravi’s plans?
Can you build a symmetrical castle that is different from any of the ones you have built so far? How many symmetrical castles can you build to Ravi’s specifications?
Teaching sequence
- Introduce the problem – create a scenario that the students are builders who have been given the task of constructing a castle from a set of plans. Make sure that the students understand how to use the 2-D views and the isometric paper.
- Give blocks and isometric paper to pairs of students.
- As the students work ask questions that focus their thinking on the models they are building.
What does the view from the top and side of your model look like?
Is there any other way to use the blocks and get the same view?
How do you know if you have found all the possibilities?
Is your castle symmetric? Describe.
What advice would you give someone about drawing with isometric paper? - Share solutions – both models and isometric drawings.
- If you are using this problem to reinforce symmetries require that the students record the symmetries on the drawing or describe them.
Extension
- Is it possible to design plans for a castle so that it can only be built in one way (uniquely)?
- On a base of three cubes by three cubes, design your own construction using top, side and front views only. Ask the questions above about your design. Stick to a three by three base. What design will give you the biggest difference between the largest number of cubes you can use and the smallest number?
Solution
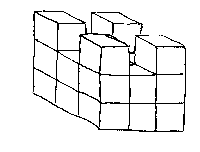
Here we show one possible castle that could be drawn from Ravi’s design.
This castle looks exactly the same from each side. It has 22 blocks.
Actually this is the most blocks that can be used for Ravi’s design. If any other block is added, there will either be three consecutive blocks across a ‘face’ of the castle or there will be an extra block right in the middle of the top layer. Either of these situations can only occur if either the front or side views look like B. So no more blocks can be added.
There may be some dispute as to what constitutes the smallest castle. Here we assume that there have to be 9 blocks on the base. Given that, we have shown the smallest number of cubes in the castle below. It is assumed that the castle looks the same from the corner block opposite the nearest block.
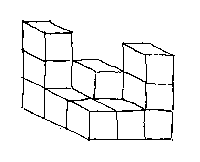
By having ‘towers’ in opposite corners the castle has the correct front and side views. This castle has 14 blocks. Is this the only smallest castle?
It is possible to build other symmetrical castles. Just add the same tower to the two opposite corners that only have one block in the picture above. This tower can have one block in it or two. The version with two blocks has more symmetry than the other one. There are other possibilities too. How many can you find?
Solution to the Extension
To make unique plans we need to have another view of the castle. Giving the view from the back might help in some cases. However, the back view of the two castles we have shown above, is the same! It would seem that four views are not good enough. So we can’t always represent 3-D objects accurately with 2-D snapshots of the kind we have used here, where we have tried a plan view and several elevation views. However, in this type of ‘cube’ building, we can determine the construction precisely if we use horizontal or vertical cross-sectional views. How many 2-D snapshots do we need, to precisely determine any castle that is three cubes high?
